In an equilateral triangle of side 12 cm, a circle is inscribed touching its sides. Find the area of the portion of the triangle not included in the circle. [ Take √3=1.73 abd π=3044]
To solve the problem, we need to find the area of the equilateral triangle and then subtract the area of the inscribed circle from it. Here are the steps to find the required area:
Step 1: Calculate the Area of the Equilateral Triangle
The formula for the area A of an equilateral triangle with side length a is given by:
A=√34a2
Substituting a=12 cm:
A=√34×(12)2
Calculating (12)2:
(12)2=144
Now substituting back into the area formula:
A=√34×144=36√3
Using the approximation √3=1.73:
A=36×1.73=62.28 cm2
Step 2: Calculate the Radius of the Inscribed Circle
The radius r of the inscribed circle in an equilateral triangle can be calculated using the formula:
r=a√36
Substituting a=12 cm:
r=12×√36=2√3
Using √3=1.73:
r=2×1.73=3.46 cm
Step 3: Calculate the Area of the Inscribed Circle
The area Ac of the circle is given by the formula:
Ac=πr2
Substituting r=3.46 cm and π=3.14:
Ac=3.14×(3.46)2
Calculating (3.46)2:
(3.46)2=11.97
Now substituting back into the area formula:
Ac=3.14×11.97≈37.6 cm2
Step 4: Calculate the Area of the Triangle Not Included in the Circle
Now, we subtract the area of the circle from the area of the triangle:
Area not included in the circle=A−Ac
Substituting the values we found:
Area not included in the circle=62.28−37.6≈24.68 cm2
Final Answer
The area of the portion of the triangle not included in the circle is approximately 24.68 cm2.
---
The formula for the area A of an equilateral triangle with side length a is given by:
The radius r of the inscribed circle in an equilateral triangle can be calculated using the formula:
The area Ac of the circle is given by the formula:
Now, we subtract the area of the circle from the area of the triangle:
The area of the portion of the triangle not included in the circle is approximately 24.68 cm2.
Topper's Solved these Questions
- AREA OF CIRCLE, SECTOR AND SEGMENT BOOK - RS AGGARWALCHAPTER - AREA OF CIRCLE, SECTOR AND SEGMENT EXERCISE - SOLVED EXAMPLE_TYPE
1 Videos
- AREA OF CIRCLE, SECTOR AND SEGMENT BOOK - RS AGGARWALCHAPTER - AREA OF CIRCLE, SECTOR AND SEGMENT EXERCISE - Exercise 16A
65 Videos
- ARITHMETIC PROGRESSIONBOOK - RS AGGARWALCHAPTER - ARITHMETIC PROGRESSIONEXERCISE - Multiple Choice Questions (Mcq)
30 Videos
Similar Questions
Knowledge Check
In an equilateral triangle of side . 24 cm., a circle is inscribed touching its sides. The area of the remaining portion of the triangle is (√3=1.732)
A98.55 sq cmB100 sq cmC101 sq cmD95 sq cmIn an equilateral triangle of side . 24 cm., a circle is inscribed touching its sides. The area of the remaining portion of the triangle is (√3=1.732)
A36.6cm2B54.2cm2C72.8cm2D98.5cm2An equilateral triangle is inscribed in a circle of radius 6 cm. Find its side.
A6√3B6√3C8√3D2√3
An equilateral triangle of side 9 cm is inscribed in a circle. Find the radius of the circle.
View SolutionAn equilateral triangle of side 6 cm is inscribed in a circle. Then radius of the circle is
View SolutionABC is an equilateral triangle inscribed in a circle of radius 4 cm. Find the area of the shaded portion.

View SolutionAn equilateral triangle is inscribed in a circles of radius 'a' unit. Find the length of the side of the triangle in terms of 'a'.
View SolutionThe area of an equilateral triangle is 100√3cm2. Taking each vertex as centre, a circle is described with radius equal to half the length of the side of the triangle, as shown in the figure. Find the area of that part o the triangle which is not included in the circles.
[Take π=3.14 and √3=1.732]
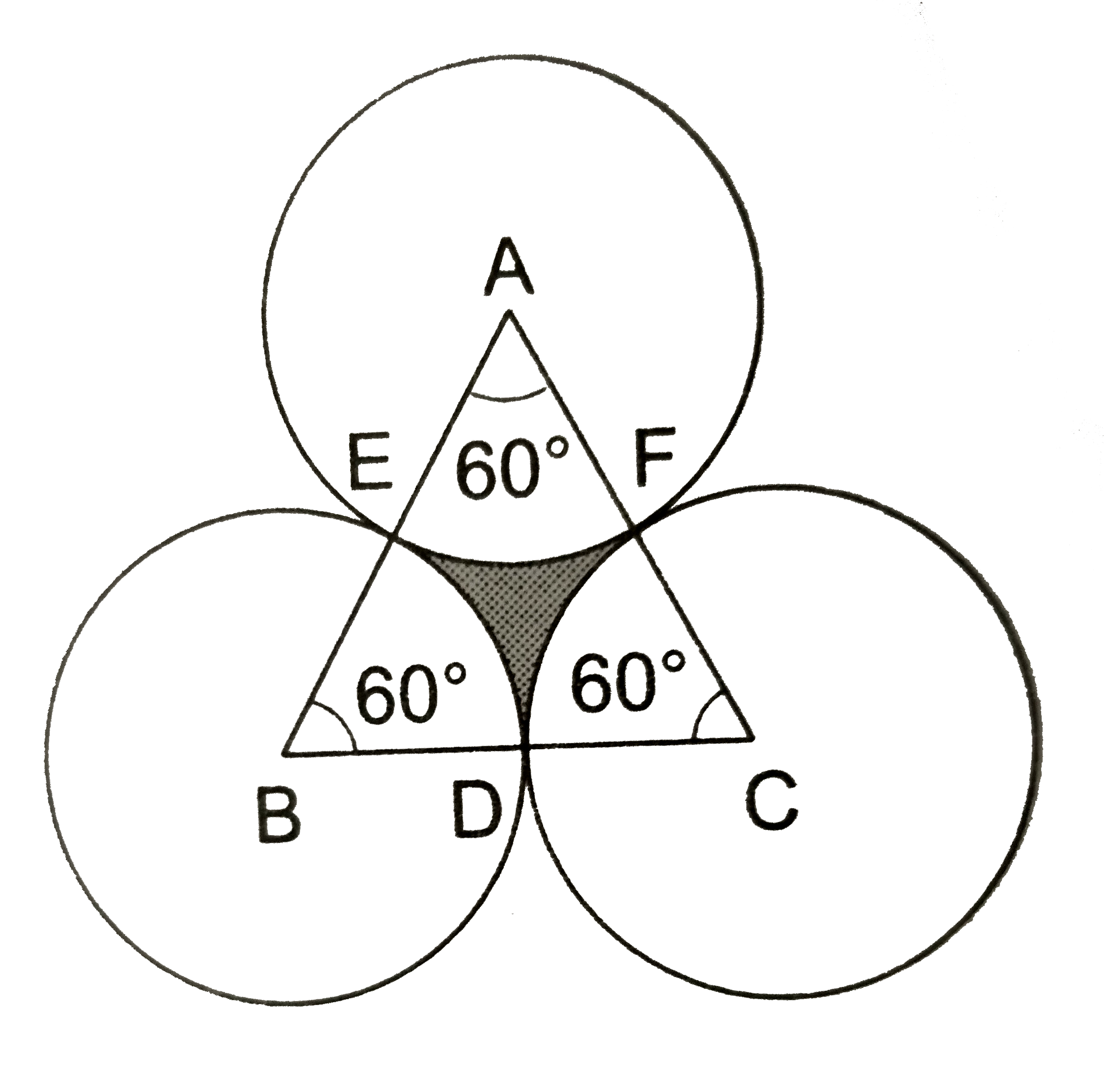
View Solution
