Find the area of a right-angled triangle, if the radius of its circumcircle is 7.5 cm and the altitude drawn to the hypotenuse is 6 cm long.

Video Solution

Text Solution

Verified by Experts

Let $Δ A B C$ be right angled at B.
Hypotenuse AC

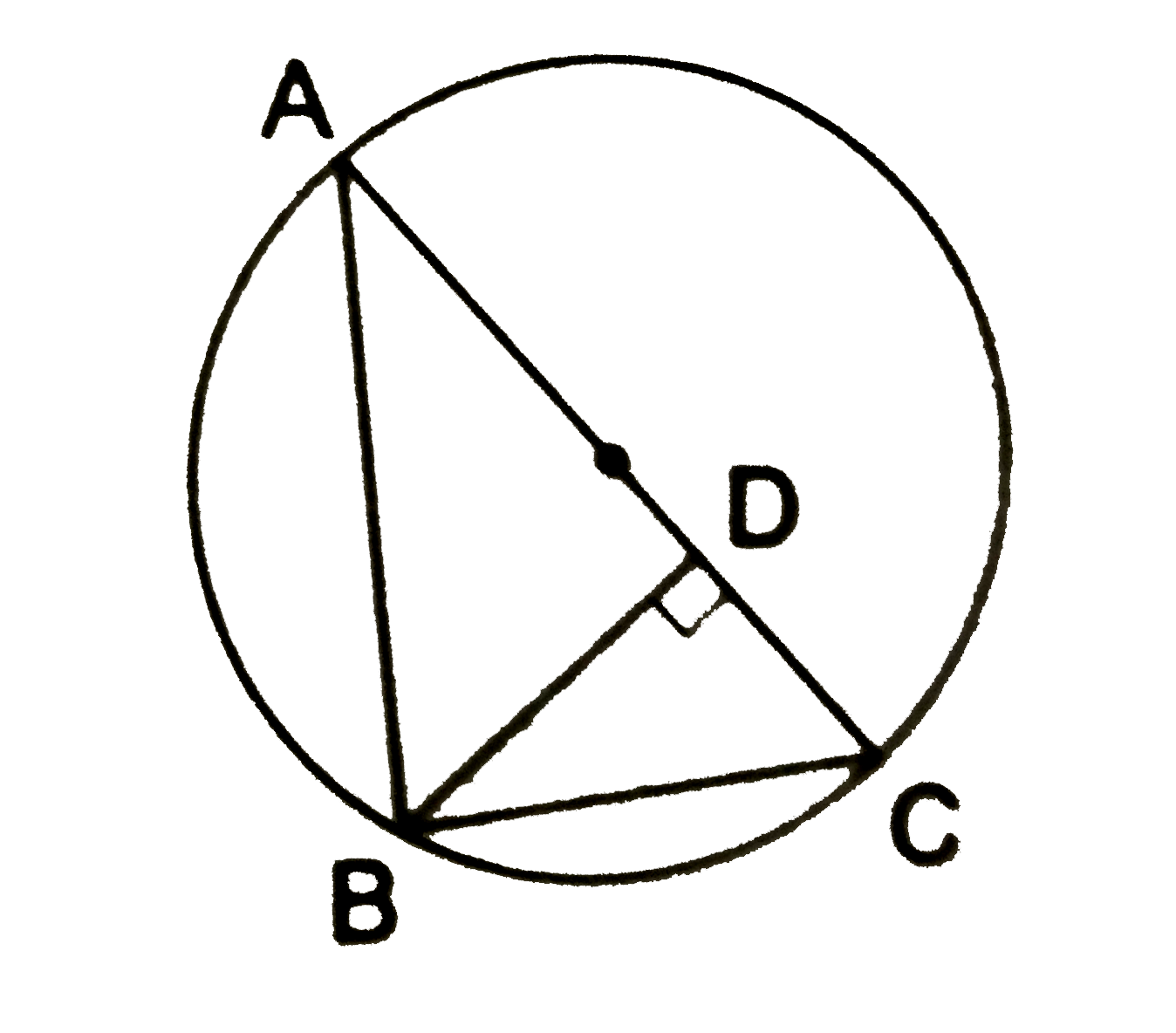
$=$ diameter of its circumcircle
$= (2 \times 7.5) c m = 15 c m$
Let $B D ⊥ A C$ . Then $B D = 6 c m$
$∴ a r (Δ A B C) = (\frac{1}{2} A C \times B D) = (\frac{1}{2} \times 15 \times 6) c m^{2} = 45 c m^{2}$

|

Updated on:21/07/2023

Class 10 MATHS AREAS RELATED TO CIRCLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
$Δ A B C$ is a right angled triangle, the radius of its circumcircle is 3 cm and the length of its altitude drawn from the opposite vertex to the hypotenuse is 2 cm. Then the area of the triangle is
A12 sq. cm
B3 sq. cm
C6 sq. cm
D5 sq. cm
Question 2 - Select One
If the perimeter of a right-angled triangle is 56 cm and area of the triangle is 84 sq. cm, then the length of the hypotenuse is (in cm)
A25
B50
C7
D24
Question 3 - Select One
In a right-angled triangle with sides a and b, hypotenuse c, the altitude drawn on the hypotenuse is x. Then
A $a b = x^{2}$
B $\frac{1}{a} + \frac{1}{b} = \frac{1}{x}$
C $a^{2} + b^{2} = 2 x^{2}$
D $\frac{1}{a^{2}} + \frac{1}{b^{2}} = \frac{1}{x^{2}}$

RS AGGARWAL-AREA OF CIRCLE, SECTOR AND SEGMENT -Test Yourself

Home
Ask Doubt
Profile