A parabolic reflector is 9 cm deep and its diameter is 24 cm. How far is its focus from the vertex?

Video Solution

Text Solution

Verified by Experts

Let us take the vertex of the reflector at the origin, and let its axis lie along the positive direction of the x-axis.

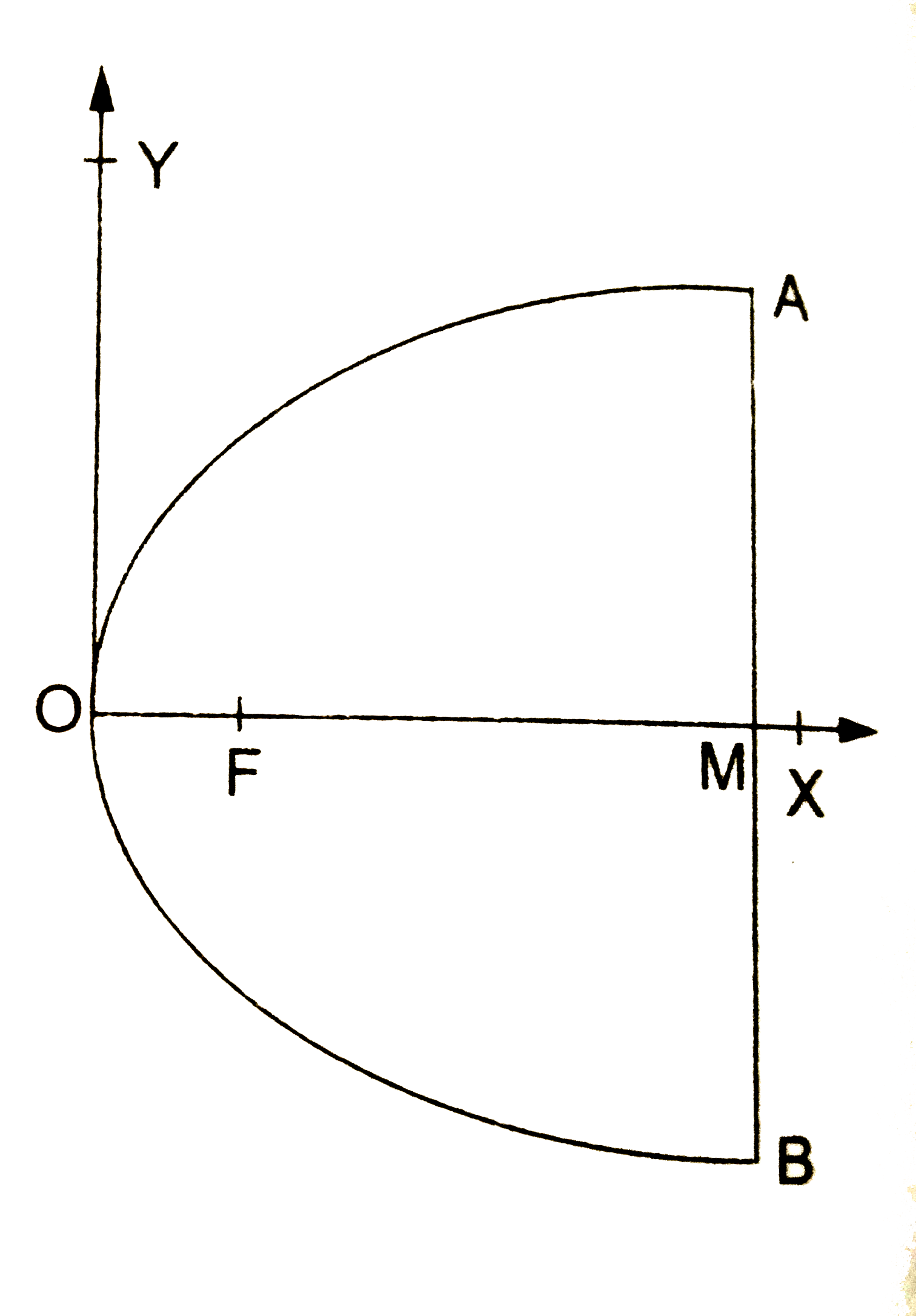
Let the equation of the parabolic shape of the reflector be
$y^{2} = 4 a x .$ ....(i)
As given, we have
OM = 9 cm and AB = 24 cm
$\Rightarrow O M = 9 c m and A M = \frac{1}{2} A B = 12 c m .$
$∴$ the coordinates of A are (9, 12).
Since A lies on (i), we have
$12 \times 12 = 4 \times a \times 9 \Rightarrow a = 4 .$
$∴$ the equation of the parabola is $y^{2} = 16 x .$
Its focus is $F (a, 0), i . e ., F (4, 0) .$
Hence, the focus is at a distance of 4 cm from the vertex.

Updated on:21/07/2023