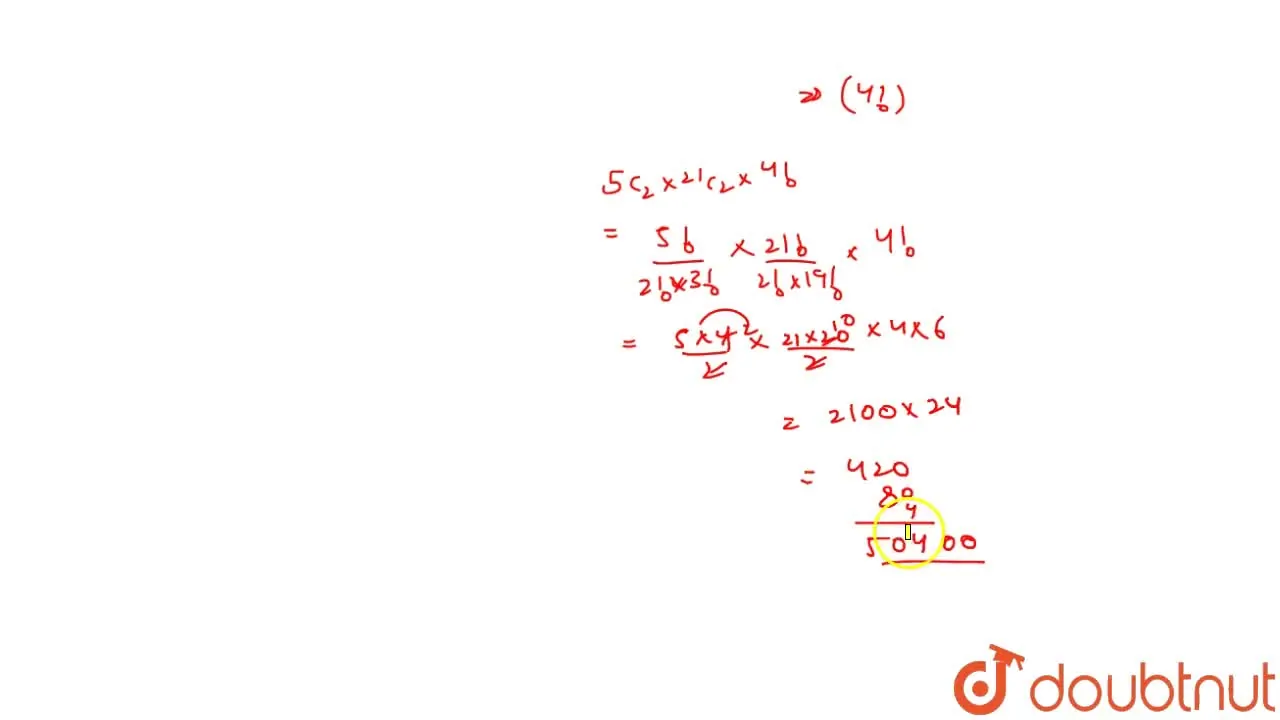
The English alphabet has 5 vowels and 21 consonants. How many words with 2 different vowels and 2 different consonants can be formed from the alphabet?

Video Solution

Text Solution

Verified by Experts

Total number of ways of choosing
(2 consonants out of 21) and (2 vowels out of 5)
$= .^{21} C_{2} \times .^{5} C_{2} = (\frac{21 \times 20}{2 \times 1} \times \frac{5 \times 4}{2 \times 1}) = 2100$ .
Number of groups, each containing 2 consonants and 2 vowels
=2100.
Each group contains 4 letters.
Number of ways of arranging 4 letters amongst themselves
$= 4 \neq (4 \times 3 \times 2 \times 1) = 24$ .
Hence, the required number of words $= (2100 \times 24) = 50400$ .

Updated on:21/07/2023

How many words, with or without meaning, each of 2 vowels and 3 consonants can be formed from the letters of the word DAUGHTER ?
A30
B3000
C36
D3600
In how many wasys can 3 consonents be selected from the English alphabet?
A $.^{21} C_{3}$
B $.^{26} C_{3}$
C $.^{21} C_{5}$
D $.^{26} C_{5}$
How many words can be formed with 3 vowels and 2 consonants taken from the word 'EQUATION'?
A $30$
B $120$
C $1200$
D $3600$