A cuboid is of dimensions 60cm×54cm×30cm.How many small cubes with side 6 cm can be placed in the given cuboid?
To find out how many small cubes with a side of 6 cm can be placed in a cuboid with dimensions 60 cm x 54 cm x 30 cm, we will follow these steps:
Step 1: Calculate the volume of the cuboid.
The volume V of a cuboid is given by the formula:
V=length×width×height
For our cuboid:
- Length = 60 cm
- Width = 54 cm
- Height = 30 cm
Calculating the volume:
V=60cm×54cm×30cm
V=97200cm3
Step 2: Calculate the volume of one small cube.
The volume V of a cube is given by the formula:
V=side3
For our small cube:
- Side = 6 cm
Calculating the volume:
V=6cm×6cm×6cm
V=216cm3
Step 3: Calculate the number of small cubes that can fit in the cuboid.
To find the number of small cubes that can fit in the cuboid, we divide the volume of the cuboid by the volume of one small cube:
Number of cubes=Volume of cuboidVolume of one cube
Number of cubes=97200cm3216cm3
Calculating the number of cubes:
Number of cubes=450
Final Answer:
Thus, 450 small cubes with a side of 6 cm can be placed in the given cuboid.
---
The volume V of a cuboid is given by the formula:
V=length×width×height
For our cuboid:
- Length = 60 cm
- Width = 54 cm
- Height = 30 cm
V=60cm×54cm×30cm
V=97200cm3
The volume V of a cube is given by the formula:
V=side3
For our small cube:
- Side = 6 cm
V=6cm×6cm×6cm
V=216cm3
To find the number of small cubes that can fit in the cuboid, we divide the volume of the cuboid by the volume of one small cube:
Number of cubes=Volume of cuboidVolume of one cube
Number of cubes=97200cm3216cm3
Calculating the number of cubes:
Number of cubes=450
Thus, 450 small cubes with a side of 6 cm can be placed in the given cuboid.
Topper's Solved these Questions
Similar Questions
Knowledge Check
A cuboid has dimensions 8 cm x 10 cm x 12 cm. It is cut into small cubes of side 2 cm. What is the percentage increase in the total surface area?
A286.2B314.32C250.64D386.5In a box whose dimensions are 12cm×4cm×3cm, how long stick can be placed ?
A13 cmB14 cmC15 cmD16 cmA cuboid of dimensions (6 cm × 4 cm × 1 cm) is painted black on both the surfaces of dimensions (4 cm × 1cm), green on the surfaces of dimensions (6 cm × 4 cm) and red on the surfaces of dimensions (6cm×1cm). Now, the block is divided into various smaller cubes of side 1 cm each. The smaller cubes, so obtained are separated.
How many cubes are there in this diagram?
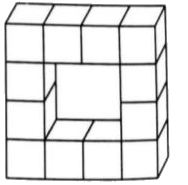 A1. 16B2. 12C3. 10D4. 8
A1. 16B2. 12C3. 10D4. 8
A cuboidal block of solid iron has dimensions 50 cm,45 cm and 34 cm. How many cuboids of size 5 cm by 3 cm by 2 cm can be obtained from this block? Assume cutting causes no wastage.
View SolutionHow many cuboids of sides 3cm,6cm and 5cm are needed to form a cube?
View SolutionA solid cuboid with dimensions 18 cm × 12 cm × 8 cm is melted and turned into a cube. What is the length of its edge ?
View SolutionA jewel box is in the shape of a cuboid of dimensions 30cm×15cm×10cm surmounted by a half part of a cylinder as shown in the figure. Then the volume of the box is
View SolutionSixteen glass spheres each of radius 2 cm are packed into a cuboidal box of internal dimensions 20 cm × 10 cm × 10 cm and then the box is filled with water. Find the volume of water filled in the box.
View Solution