Two sets A and B are as under $A = | (a, b) \in R \times R | a - 5 | < 1 and | b - 5 ∣ < 1} B = [(a, b) \in R \times R : 4 {(a - 6)}^{2} + 9 {(b - 5)}^{2} \leq 36} T h e n :$ (1) $B \subset A$ (2) $A \subset B$ (3) $A \cap B = ϕ (a n e m p t y s e t) (4) \neq i t h e r$ A sub B $n or$ B sub A

A

neither $A \subset B nor B \subset A$

B

$B \subset A$

C

$A \subset B$

D

$A \subset B = ϕ$ (an empty set)

Video Solution

Text Solution

Verified by Experts

Let a x=a and b=y
So, we have $| x - 5 | < 1 and | y - 5 | < 1 (1)$
$| x - 5 | < 1$
$\Rightarrow - 1 < x - 5 < 1$
$\Rightarrow 4 < x < 6$
Similary, $4 < y l, t 6$
Thus, we have a square for inequality (1)
Now, $4 {(x - 6)}^{2} + 9 {(y - 5)}^{2} \leq 36$
$\Rightarrow \frac{{(x - 6)}^{2}}{9} + \frac{{(y - 5)}^{2}}{4} \leq (2)$
Points satsfying above indquality lies inside ellipse or on the ellipse having center at (6,5).
Regios of both the inequalities are plotted as shown in the following figure :

Updated on:21/07/2023

|

Class 12 MATHS QUESTION BANK

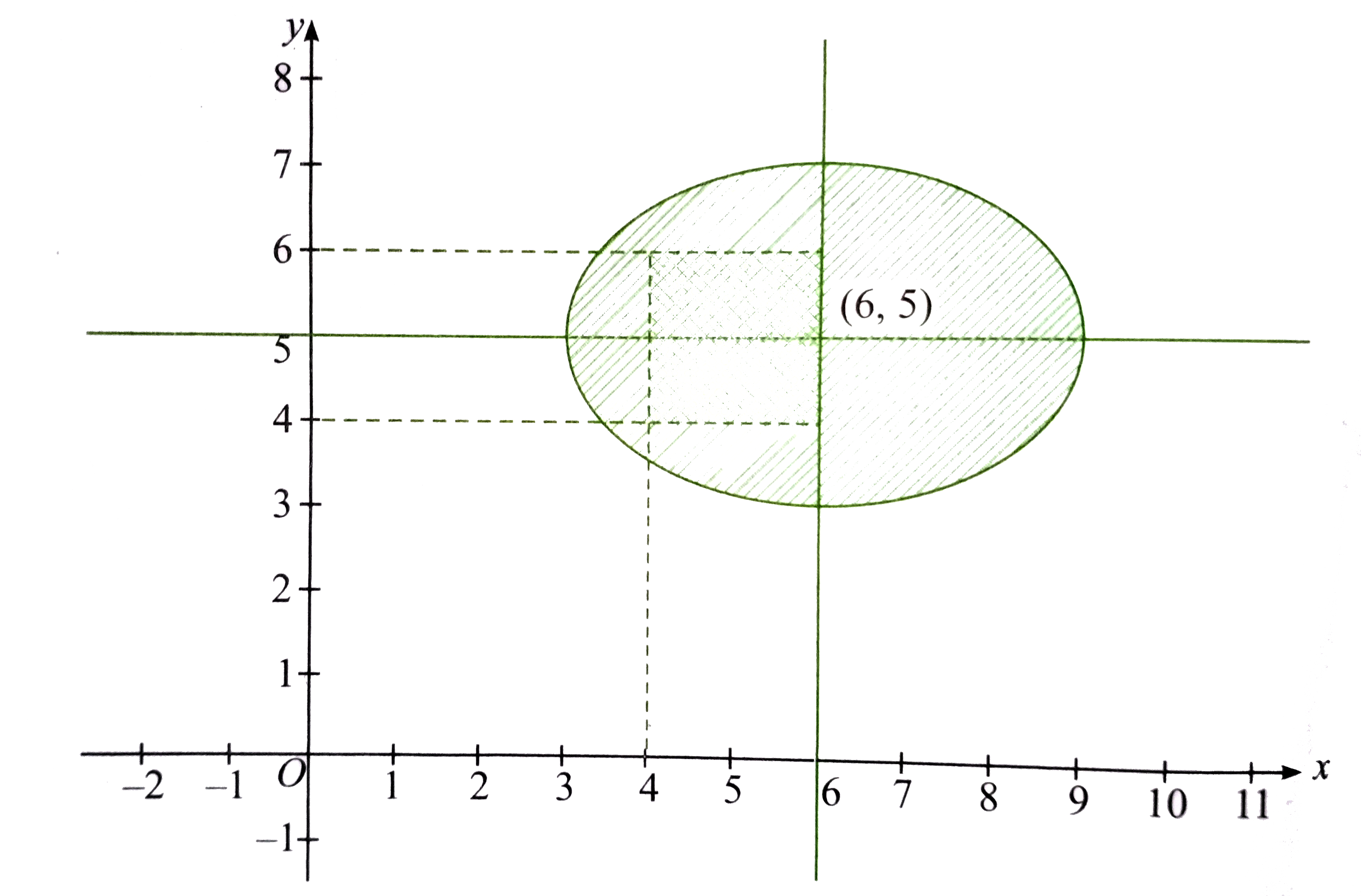
Similar Questions

Knowledge Check

If $A \subset B$ , then $A \cup B = ?$
A $ϕ$
BA
CB
DNone of these
If $A \subset B$ and $A \neq B$ , then
AA is called a proper subset of B
BA is called a super set of B
CA is not a subset of B
DB is a subset of A

Recommended Questions

Home
Ask Doubt
Profile