The radius of the circle passing through the foci of the ellipse $\frac{x^{2}}{16} + \frac{y^{2}}{9}$ and having its center (0, 3) is 4 (b) 3 (c) $\sqrt{12}$ (d) $\frac{7}{2}$

$x^{2} + y^{2} - 6 y - 7 = 0$

$x^{2} + y^{2} - 6 y + 7 = 0$

$x^{2} + y^{2} - 6 y - 5 = 0$

$x^{2} + y^{2} - 6 y + 5 = 0$

Video Solution

Text Solution

Verified by Experts

Coordinates for focia re (ae, 0) , (-ar, 0)
$∴ e = \sqrt{1 - \frac{9}{16}} \Rightarrow e = \frac{\sqrt{7}}{4}$
Coordinates of foci are $(\sqrt{7}, 0), (- \sqrt{7}, 0)$

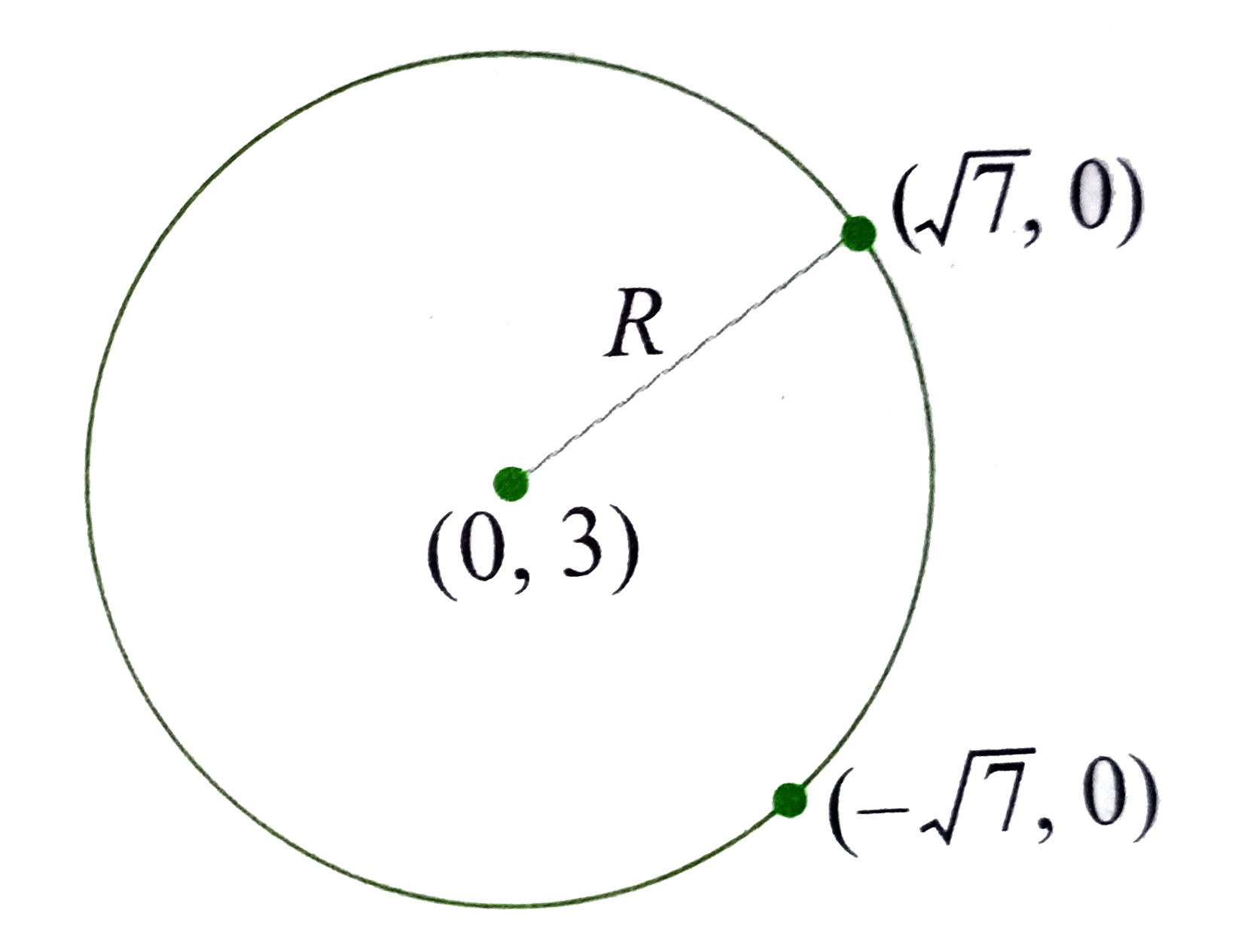
Radius $R = \sqrt{7 + 9} = 4$
Thus, equation of circle is ${(x - 0)}^{2} + {(y - 3)}^{2} = 4^{2} or x^{2} + y^{2} - 6 y + 9 = 16$
or $x^{2} + y^{2} - 6 y 7 = 0$

Updated on:21/07/2023

The radius of the circle passing through the foci of the ellipse $\frac{x^{2}}{16} + \frac{y^{2}}{9} = 1$ and having its centre (0, 3) is
A4
B $\frac{3}{7}$
C $\sqrt{12}$
D $\frac{7}{2}$
The radius of the circle passing through the foci of the ellipse $\frac{x^{2}}{16} + \frac{y^{2}}{9} = 1$ and having its centre (0, 3) is
A4
B $\frac{3}{7}$
C $\sqrt{12}$
D $\frac{7}{2}$
The radius of the circle passing through the foci of the ellipse $9 x^{2} + 16 y^{2} = 144$ and having its centre at (0, 3), is
A4
B3
C $\sqrt{12}$
D $7 / 2$