Tangents drawn from the point $P (2, 3)$ to the circle $x^{2} + y^{2} - 8 x + 6 y + 1 = 0$ points A and B. The circumcircle of the $Δ P A B$ cuts the director circle of ellipse $\frac{{(x - 5)}^{2}}{9} + \frac{{(y - 3)}^{2}}{b^{2}} = 1$ orthogonally. Find the value of $b^{2}$ .

Video Solution

Text Solution

Verified by Experts

The center of the given circle is O(4,3)

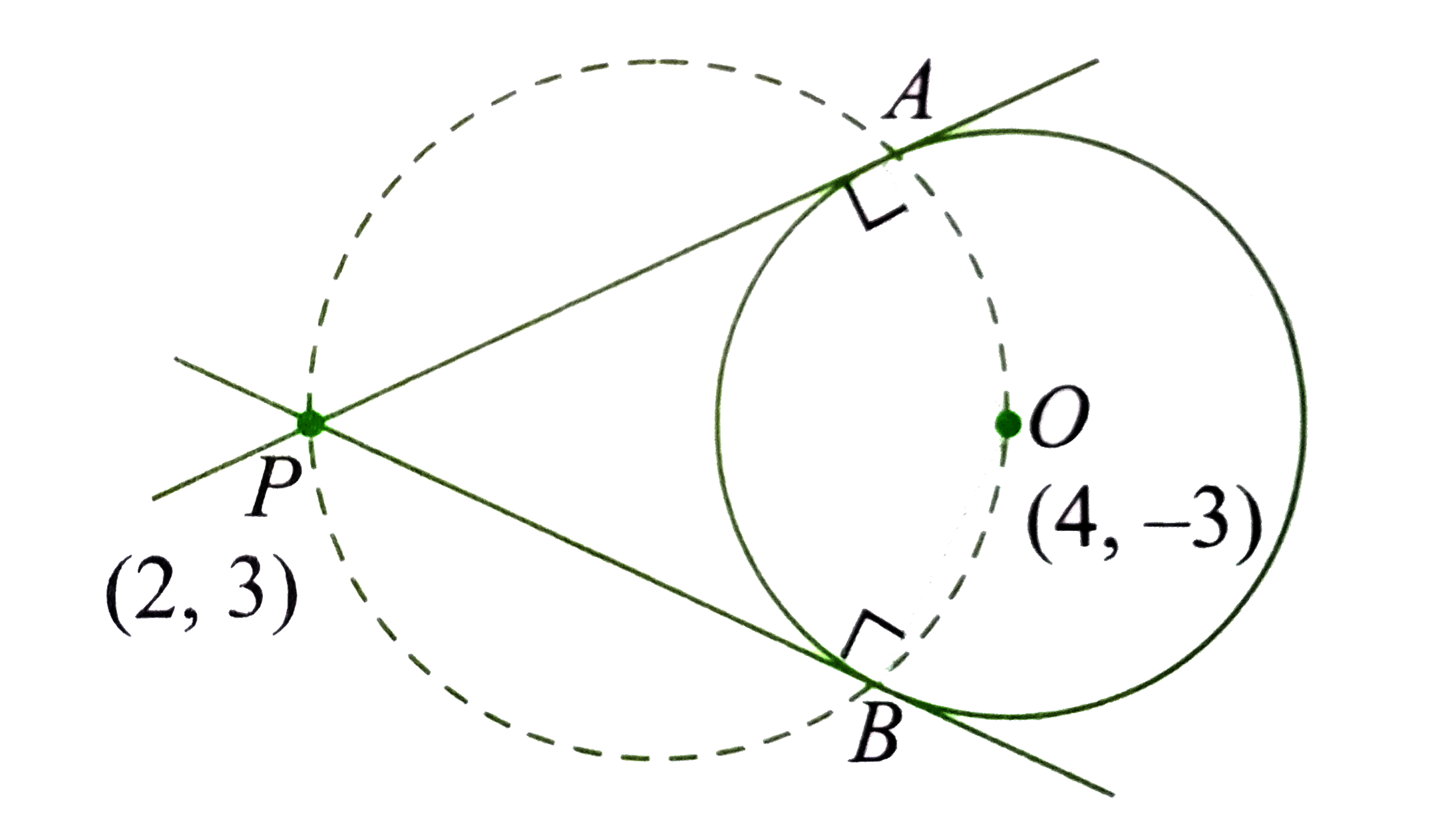
The crircumericle of $Δ P A B$ will circumscribe the quadrilateral PBOA alos. Hence one of the diameters must be OP
So, the quations of circumcricle of $Δ P A B$ will be
$(x - 2) (x - 4) + (y - 3) (y + 3) = 0$
or $x^{2} + y^{2} - 6 x = 0 (1)$
Director circle of the given ellipe will be
${(x + 5)}^{2} + {(y - 3)}^{2} = 9 + b^{2}$
or $x^{2} + y^{2} + 10 x - 6 y + 25 = 0 (2)$
So,from (1) and (2), by applying the condition of orthogonoality we ge
$2 [- 3 (5) + 0 (- 3)] = - 1 + 25 - b^{2} or - 30 = 24 - b^{2}$
Hence, $b^{2} = 54$

Updated on:21/07/2023

Tangents drawn from the point P(1,8) to the circle $x^{2} + y^{2} - 6 x - 4 y - 11 = 0$ touch the circle at the points A and B the equation of the circumcircle of $△ P A B$ is
A $x^{2} + y^{2} \div 4 x - 6 y + 19 = 0$
B $x^{2} + y^{2} - 4 x - 10 y + 19 = 0$
C $x^{2} + y^{2} - 2 x + 6 y - 29 = 0$
D $x^{2} + y^{2} - 6 x - 4 y + 19 = 0$
Tangents drawn from the point P(1,8) to the circle $x^{2} + y^{2} - 6 x - 4 y - 11 = 0$ touch the circle at the points A and B. The equation of the circumcircle of the triangle PAB is:
A $x^{2} + y^{2} + 4 x - 6 y + 19 = 0$
B $x^{2} + y^{2} - 4 x - 10 y + 19 = 0$
C $x^{2} + y^{2} - 2 x + 6 y - 20$
D $x^{2} + y^{2} - 6 x - 4 y + 19 = 0$
Tangents drawn from the point P(1,8) to the circle $x^{2} + y^{2} - 6 x - 4 y - 11 = 0$ touch the circle at the points A and B. The equation of the circumcircle of the triangle PAB is:
A $x^{2} + y^{2} + 4 x - 6 y + 19 = 0$
B $x^{2} + y^{2} - 4 x - 10 y + 19 = 0$
C $x^{2} + y^{2} - 2 x + 6 y - 20$
D $x^{2} + y^{2} - 6 x - 4 y + 19 = 0$