Which of the following is/are true about the ellipse x2+4y2−2x−16y+13=0? the latus rectum of the ellipse is 1. The distance between the foci of the ellipse is 4√3. The sum of the focal distances of a point P(x,y) on the ellipse is 4. Line y=3 meets the tangents drawn at the vertices of the ellipse at points P and Q . Then PQ subtends a right angle at any of its foci.
A
The latus rectum of the ellipse is 1
B
The distance between the foci of the ellipse is 4√3
C
The sum of the focal distances of a point P(x,y) on the ellipse is 4
D
Liney=3 meets the tangents drawn at the vertices of the ellipse at points P and Q. Then PQ subtends a right angle at any of its foci.
Video Solution
Text Solution
x2+4y2−2x−16y+13=0
or (x2−2x+1)+4(y2−4y+4)=4
or (x−1)24+(y−2)21=1
∴ Length of latus rectum =2×12=1
Also, e=√1−14=√34
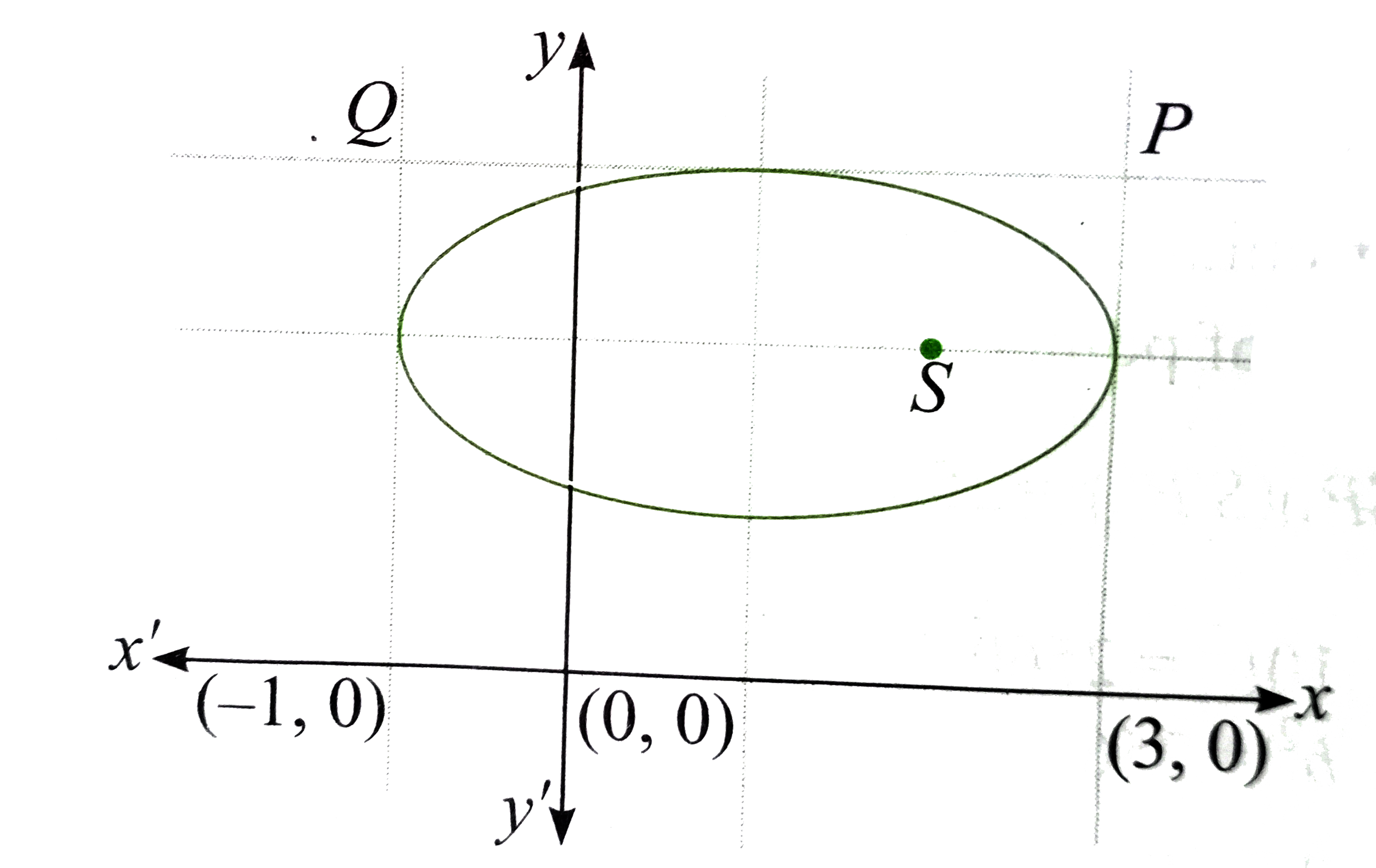
or 2ae=2×2×√32=√3
Sum of the focal distance =2a=4
Tenagents at the vertices are
x−1=±2
or x=3,−1
Therefore the line y=3 intersects these at points P(3,3) and Q(-1,3)
One of the foci is S(√3+1,2)
Slop of PS=12−√3
Slopo of QS=1−2−√3
∴ Product of slops =12−√3×1−2−√3=−1
|
Similar Questions
Knowledge Check
The sum of focal distances of a point on the ellipse x24+y29=1 is:
A4 unitsB6 unitsC8 unitsD10 unitsThe sum of focal distances of a point on the ellipse x24+y29=1 is:
A4 unitsB6 unitsC8 unitsD10 units3x+4y=12√2 is the tangent to the ellipse x2a2+y29=1 then the distance between focii of ellipse is-
A2√5B2√7C2√11D2√13
The sum of the distances of any point on the ellipse 3x2+4y2=24 from its foci is
View SolutionThe sum of the distances of any point on the ellipse 3x2+4y2=12 from its directrix is
View SolutionThe sum of the distances of any point on the ellipse 3x2+4y2=24 from its foci , is
View SolutionDistance between the foci of the ellipse 25x2+16y2+100x−64y−236=0 is
View SolutionFocal distances of the point P(5,4√3) on the ellipse
64x2+100y2=6400 are
View Solution
