The locus of the point which divides the double ordinates of the ellipse x2a2+y2b2=1 in the ratio 1:2 internally is
A
x2a2+9y2b2=1
B
x2a2+9y2b2=19
C
9x2a2+9y2b2=1
D
none of these
Video Solution
Text Solution
The correct Answer is:A
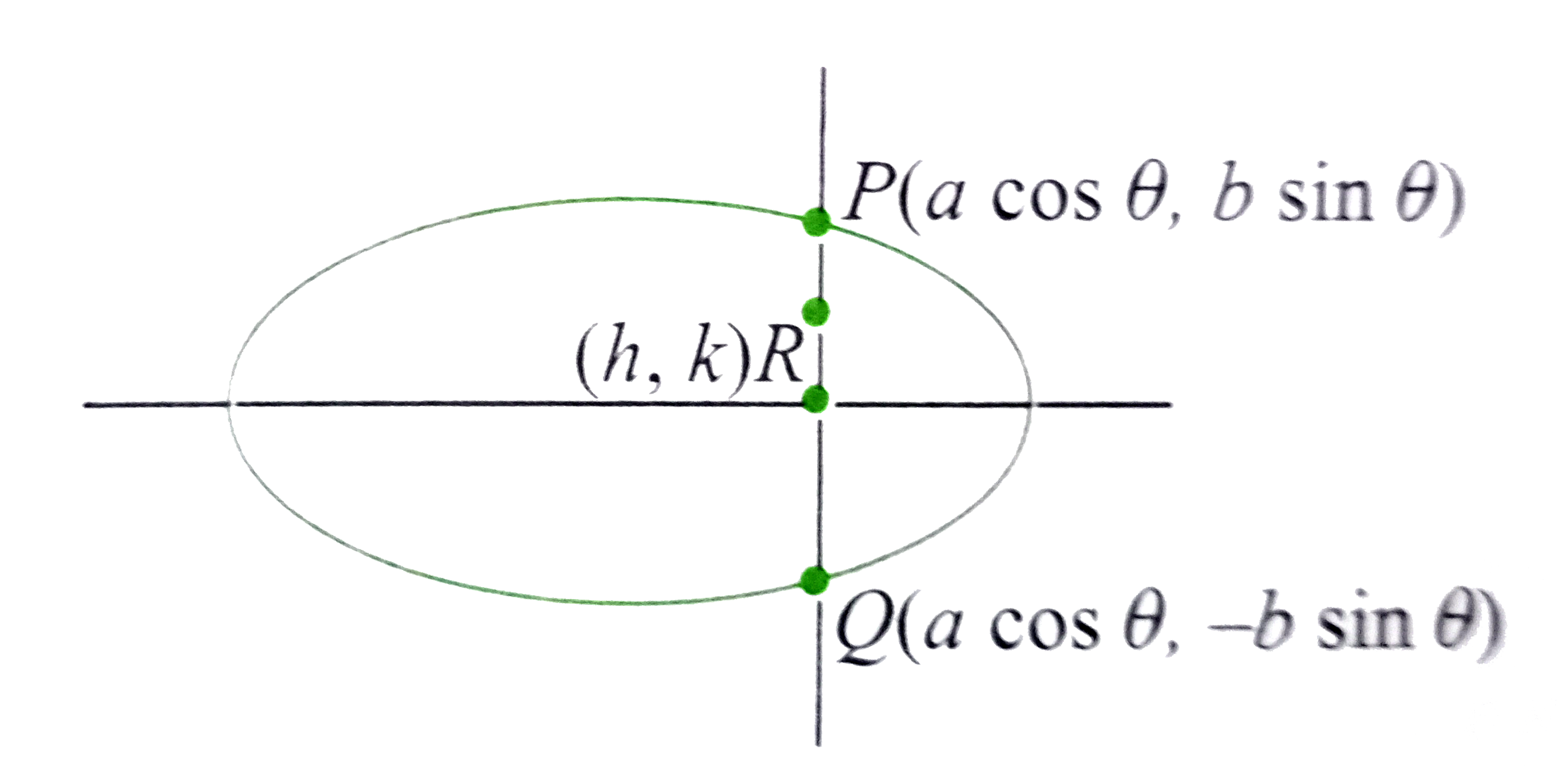
Let P≡(acosθ,bsinθ),Q≡(acoθ,−bsinθ)
PR:RQ=1:2
∴h=acosθ
or cosθ=ha (1)
or k=b3sinθ
or sinθ=3kb (2)
On squaring and adding (1) and (2), we get
x2a2+9y2b2=1
|
Similar Questions
Knowledge Check
Locus of mid-point of the focal chord of ellipse x2a2+y2b2=1 with eccentricity e is
Ax2a2−y2b2=exaBx2a2+y2b2=exaCx2+y2=a2+b2DnoneThe locus of the mid-points of the portion of the tangents to the ellipse x2a2+y2b2=1 intercepted between the axes is
Ax2a2+y2b2=4Ba2x2+b2y2=4Cx2a2−v2a2=4Dnone of thesethe locus of the point of intersection of tangents to the ellipse x2a2+y2b2=1 which meet at right , is
Aa circleBa parabolaCan ellipseDa hyperbola
The locus of the point which divides the join of A(−1,1) and a variable point P on the circle x2+y2=4 in the ratio 3:2 is
View SolutionThe locus of the point of intersection of two prependicular tangents of the ellipse x29+y24=1 is
View SolutionThe locus of a point which divides the join A(-1,1) and a variable point on the circle x2+y2=4 in the ratio 3:2 is ...........
View SolutionThe locus of mid-points of a focal chord of the ellipse x2a2+y2b2=1
View SolutionThe locus of the point the chord of contact of tangents from which to the ellipse x2a2+y2b2=1 touches the circle x2+y2=c2 is...........
View Solution