An equilateral triangle is inscribed in an ellipse whose equation is x2+4y2=4 If one vertex of the triangle is (0,1) then the length of each side is
8√313
24√313
16√313
48√313
The correct Answer is:C
We have x24+y2=1 (1)
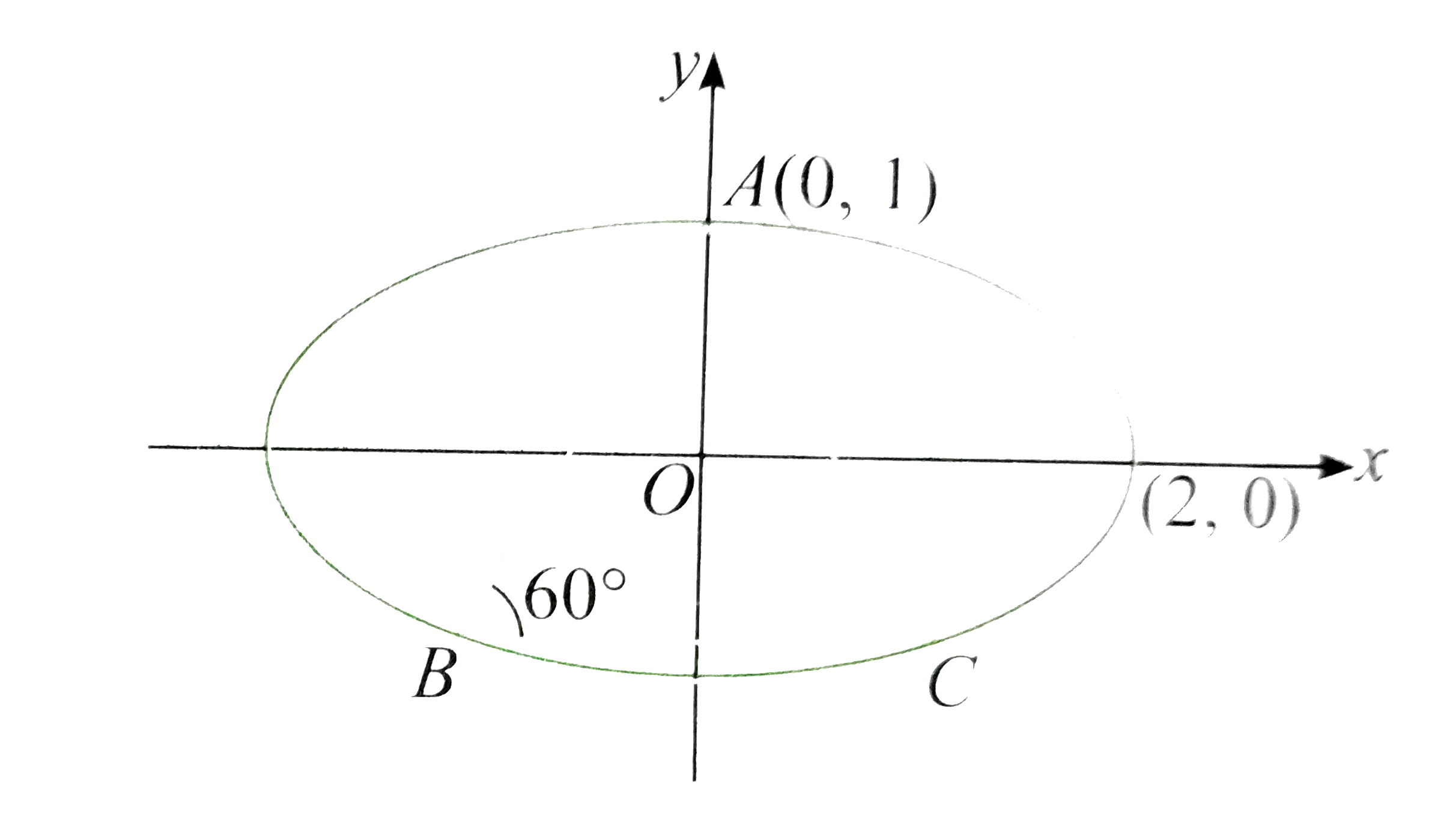
Equation of the sides AB is
y−1=√3(x−0)
⇒y=√3x+1 (2)
Solving (1) and (2), we get
x24+(√3x+1)2=1
⇒x2+4(3x2+1+2√3x)=4
⇒13x2+8√3x=0
rArx=0orx=−8√313
Putting x=−8√313 in (2), we get
y=−√3(8√313)+1=1−2413=−1113
Hence, B≡(8√313,1113)
∴C≡(8√313,1113)
∴BC≡16√313
Similar Questions
The base of an equilateral triangle is along theline given by 3x+4y=9. If a vertex of the triangle is (1,2), then the length of a side of the triangle is
View SolutionAn equilateral triangle is inscribed in the parabola y2=4ax, such that one vertex of this triangle coincides with the vertex of the parabola. Then find the side length of this triangle.
View Solution
Knowledge Check
An equilateral triangle is inscribed in ellipse whose equation is x2+4y2=4, one vertex of triangle is (0, 1) and one altitude is contained in y -axis and length of each side is √mn (where m and n are relatively prime), then m + n is:
A937B973C793D739An equilateral triangle is inscribed in the ellipse whose equation is x2+4y2=4. One vertex of the triangle (0, 1) and one altitude is contained in the y - axis. If the length of each side is k√3 units, then k is
A1613B813C1316D138An equilateral triangle is inscribed in the ellipse x2+3y2=3 such that one vertex of the triangle is (0, 1) and one altitude of the triangle is along the y-axis. The length of its side is
A4√3/5B3√3/5C6√3/5D2√3
An equilateral triangle is inscribed in the parabola y2=4ax where one vertex is at the vertex of the parabola. Find the length of the side of the triangle.
View SolutionAn Equilateral triangle is inscribed in the parabola y2=4x if one vertex of triangle is at the vertex of parabola then Radius of circum circle of triangle is
View SolutionAn Equilateral triangle is inscribed in the parabola y^(2)=4x if one vertex of triangle is at the vertex of parabola then Radius of circum circle of triangle is
View SolutionIf one vertex of an equilateral triangle is at (2.−1) 1base is x+y−2=0, then the length of each side, is
View SolutionAn equilateral triangle is inscribed in y2=8x so that one angular point of the triangle is at the vertex of the parabola . Length of side of this triangle is
View Solution