Find the range of eccentricity of the ellipse x2a2+y2b2=1, (where a > b) such that the line segment joining the foci does not subtend a right angle at any point on the ellipse.
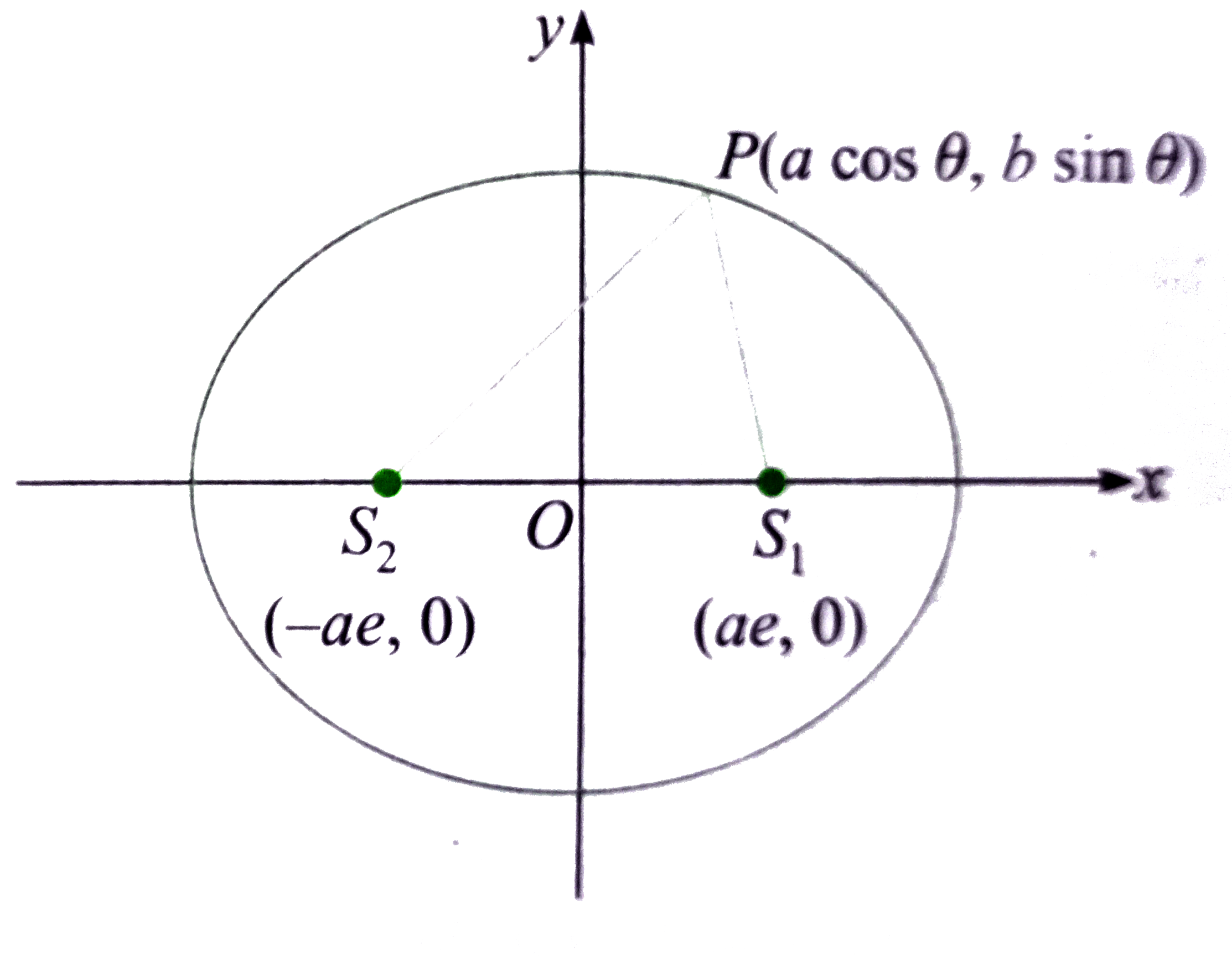
Let any point P on the ellipse b P(acosθ,bsinθ) .
If ∠S1PS2=po/2, then P less on the circle having S1S2 as its diameter
Therefore, the equation of the circle drawn on S1s2 as its x2y2=a2e2
a2−b2
Since the point P should not lie on the ellipse, there should not be any point on the intersection of
x2+y2=a2−b2andx2a2+y2b2=1
∴a2−b2<b2
or 2b2>a2
or1−e2>12
or e2<12
or e∈(0,1√2)
Similar Questions
Find the range of eccentricity of the ellipse x2a2+y2b2=1, (where a > b) such that the line segment joining the foci does not subtend a right angle at any point on the ellipse.
View Solution" Eccentricity of ellipse "x2a2+y2b2=1" such that the line joining the foci subtends a right angle only at two points on ellipse is "
View Solution
Knowledge Check
If e is eccentricity of the ellipse x2a2+y2b2=1(where,a<b), then
Ab2=a2(1−e2)Ba2=b2(1−e2)Ca2=b2(e2−1)Db2=a2(e2−1)If e' is the eccentricity of the ellipse x2a2+y2b2=1 (a>b), then
Aa2=b2(1−e2)Bb2=a2(1−e2)Ca2=b2(e2−1)Db2=a2(e2−1)The intercept made by the auxiliary circle of the ellipse x2a2+y2b2=1(a>b>1) on any tangent to the ellipse, subtends a right angle at its ceotre if
Aa≤b2Ba≤b√2Ca<2bDnone of these
Find the foci and eccentricity of ellipse x216+y24=1.
View SolutionIn an ellipse x2a2+y2b2=1 where a>b, the distance of the normal from the centre does not exceed by ?
View SolutionThe condition that the chord of the ellipse x2/a2+y2/b2=1, whose middle point is (x1,y1) subtends a right angle at the centre of the ellipse is .......... .
View SolutionNumber of points on the ellipse x2a2+y2b2=1 at which the normal to the ellipse passes through at least one of the foci of the ellipse is
View SolutionIf the ellipse of the auxiliary circle of the ellipse x2a2+y2b2=1(a>b) is twice the area of the ellipse, then the eccentricity of the ellipse is
View Solution
