P is the point on the ellipse is $\frac{x^{2}}{16} + \frac{y^{2}}{9} = 1 and Q$ is the corresponding point on the auxiliary circle of the ellipse. If the line joining the center C to Q meets the normal at P with respect to the given ellipse at K, then find the value of CK.

Video Solution

Text Solution

Verified by Experts

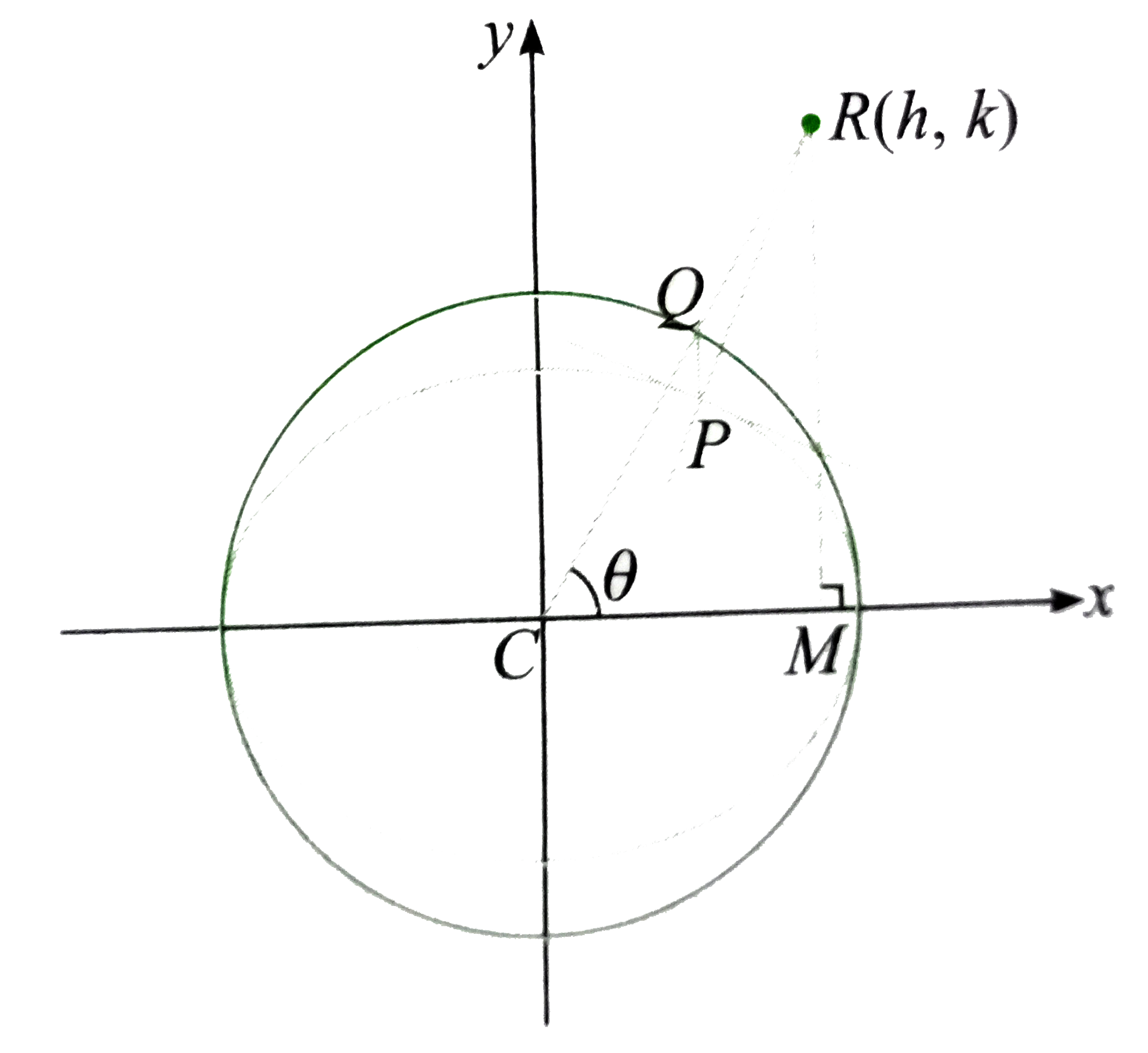
Equation of normal to ellipse
$\frac{x^{2}}{16} + \frac{y^{2}}{9} = 1$ at $P (4 cos θ, 3 sin θ)$ is $\frac{4 x}{cos θ} - \frac{3 y}{sin θ} = 7$
Normal passes through R(h,k)
$∴ 4 \frac{h}{cos θ} - 3 \frac{k}{sin θ} = 7$
$\Rightarrow 4 C R - 3 C R = 7$
$\Rightarrow C R = 7$

Updated on:21/07/2023

P is a point on the ellipse $E : \frac{x^{2}}{a^{2}} + \frac{y^{2}}{b^{2}} = 1$ and P' be the corresponding point on the auxiliary circle C: $x^{2} + y^{2} = a^{2}$ . The normal at P to E and at P' to C intersect on circle whose radius is
Aa+b
Ba-b
C2a
D2b
P is a point on the ellipse $\frac{x^{2}}{a^{2}} + \frac{y^{2}}{b^{2}} = 1 (a > b)$ and Q is the point corresponding to P on the auxiliary circle $x^{2} + y^{2} = a^{2}$ . N is the foot of the perpendicular from P on the major axis of the ellipse. $\frac{P N}{P Q}$ is equal to:
A $\frac{b}{a - b}$
B $\frac{a}{a - b}$
C $\frac{b}{a + b}$
D $\frac{a}{a + b}$
Q is a point on the auxiliary circle of an ellipse. P is the corresponding point on ellipse. N is the foot of perpendicular from focus S, to the tangent of auxiliary circle at Q. Then
ASP=SN
BSP=PQ
CPN=SP
DNQ=SP