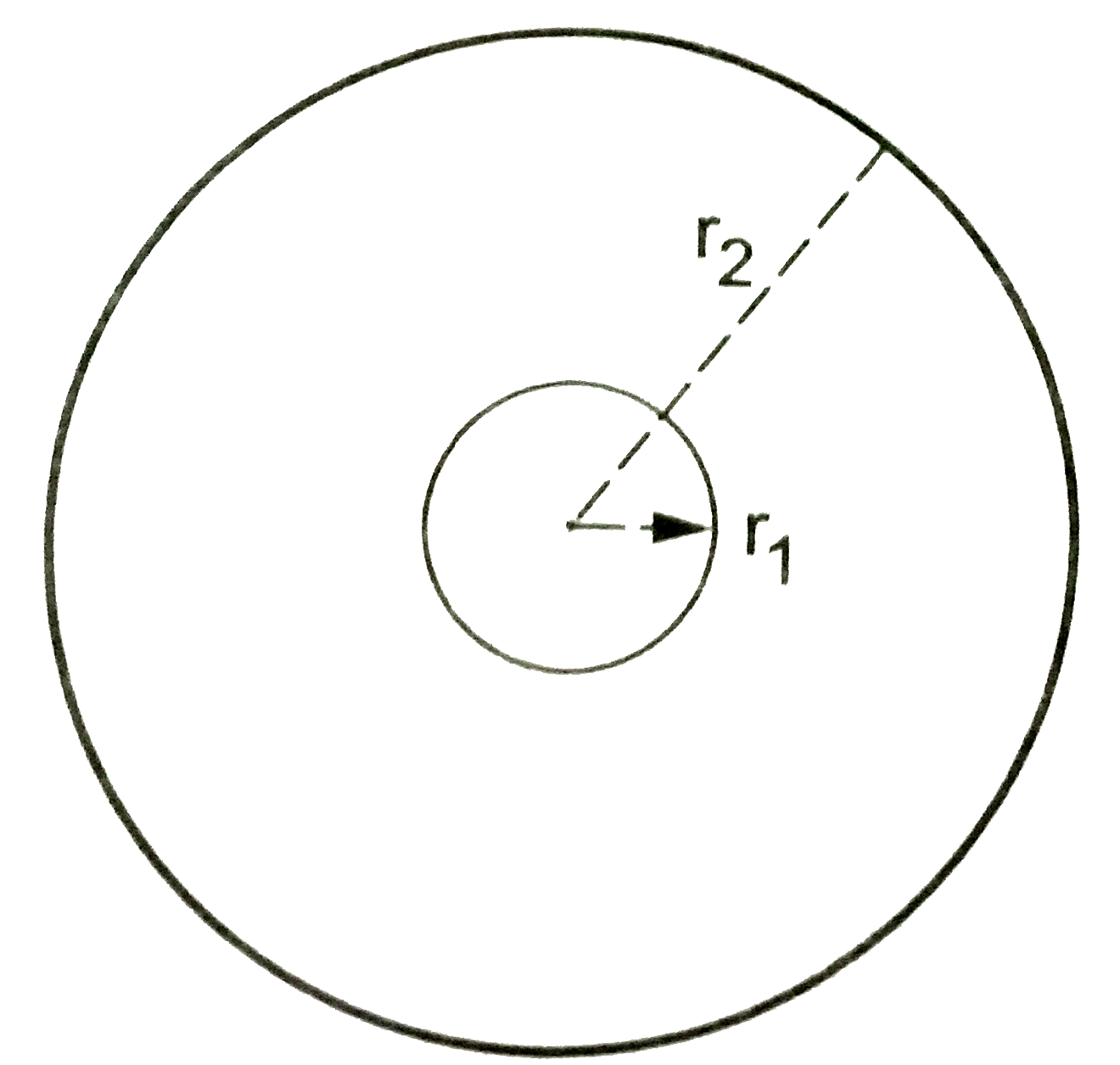
Two concentric circular coil,s one of small radius $r_{1}$ and the other of large radius $r_{2}$ , such that $r_{1} < < r_{2}$ , are placed co-axially with centres coinciding. Obtain the mutual inductance of the arrangement.Solution in Bengali

Video Solution

Text Solution

Verified by Experts

Let a current $I_{2}$ flow through the outer circular coil. The field at the centre of the coil is $B_{2} = μ_{0} I_{2} / 2 r_{2}$ . Since the other co-axially placed coil has a very small radius, $B_{2}$ may be considered constant over its cross-sectional area. Hence,
$Φ_{1} = π r_{1}^{2} B_{2}$
$= \frac{μ_{0} π r_{1}^{2}}{2 r_{2}} I_{2}$
$M_{12} I_{2}$
Thus,
$M_{12} = \frac{m i u_{0} π r_{1}^{2}}{2 r_{2}}$
From Eq. (6.14)
$M_{12} = M_{21} = \frac{μ_{0} π r_{1}^{2}}{2 r_{2}}$
Note that we calculated M12 from an approximate value of $Φ_{1}$ , assuming the magnetic field $B_{2}$ to be uniform over the area $π r_{1}^{2}$ . However, we can accept this value because $r_{1} < < r_{2}$ .

Updated on:21/07/2023

|

Class 12 PHYSICS ELECTROMAGNETIC INDUCTION

Topper's Solved these Questions

Similar Questions

NCERT BANGLISH-ELECTROMAGNETIC INDUCTION-ADDITIONAL EXERCISES

Home
Ask Doubt
Profile