In deep space, sphere A of mass 20 kg is located at the origin of an x axis and sphere B of mass 10 kg is located on the axis at x = 0.95 m. Sphere B is released from rest while sphere A is held at the origin. (a) What is the gravitational potential energy of the two-sphere system just as B is released? (b) What is the kinetic energy of B when it has moved 0.15 m toward A?
To solve the problem step by step, we will break it down into two parts as per the question.
Part (a): Gravitational Potential Energy of the Two-Sphere System Just as B is Released
1. Identify the masses and their positions:
- Mass of sphere A, mA=20kg (at the origin, x=0m)
- Mass of sphere B, mB=10kg (at x=0.95m)
2. Calculate the distance between the two spheres:
- The distance rAB=0.95m.
3. Use the formula for gravitational potential energy (U):
U=−GmAmBrAB
where G is the gravitational constant, G≈6.67×10−11N m2/kg2.
4. Substitute the values into the formula:
U=−(6.67×10−11)×(20)×(10)0.95
5. Calculate the value:
U=−(6.67×10−11)×2000.95≈−1.404×10−8J
Part (b): Kinetic Energy of B When It Has Moved 0.15 m Toward A
1. Determine the new position of sphere B:
- Sphere B has moved 0.15 m toward A, so its new position x′=0.95−0.15=0.80m.
2. Calculate the new distance between the two spheres:
- The new distance r′AB=0.80m.
3. Calculate the new gravitational potential energy (U'):
U′=−GmAmBr′AB=−(6.67×10−11)×(20)×(10)0.80
4. Substitute the values into the formula:
U′=−(6.67×10−11)×2000.80
5. Calculate the value:
U′≈−1.6675×10−8J
6. Use the conservation of energy principle:
Uinitial+Kinitial=Ufinal+Kfinal
Since Kinitial=0 (sphere B is released from rest):
Uinitial=U′+Kfinal
Rearranging gives:
Kfinal=Uinitial−U′
7. Substituting the values:
Kfinal=(−1.404×10−8)−(−1.6675×10−8)
8. Calculate the kinetic energy:
Kfinal=−1.404×10−8+1.6675×10−8=0.2635×10−8J≈2.635×10−9J
Final Answers:
(a) The gravitational potential energy of the two-sphere system just as B is released is approximately −1.404×10−8J.
(b) The kinetic energy of B when it has moved 0.15 m toward A is approximately 2.635×10−9J.
- Mass of sphere A, mA=20kg (at the origin, x=0m)
- Mass of sphere B, mB=10kg (at x=0.95m)
- The distance rAB=0.95m.
U=−GmAmBrAB
where G is the gravitational constant, G≈6.67×10−11N m2/kg2.
U=−(6.67×10−11)×(20)×(10)0.95
U=−(6.67×10−11)×2000.95≈−1.404×10−8J
- Sphere B has moved 0.15 m toward A, so its new position x′=0.95−0.15=0.80m.
- The new distance r′AB=0.80m.
U′=−GmAmBr′AB=−(6.67×10−11)×(20)×(10)0.80
U′=−(6.67×10−11)×2000.80
U′≈−1.6675×10−8J
Uinitial+Kinitial=Ufinal+Kfinal
Since Kinitial=0 (sphere B is released from rest):
Uinitial=U′+Kfinal
Rearranging gives:
Kfinal=Uinitial−U′
Kfinal=(−1.404×10−8)−(−1.6675×10−8)
Kfinal=−1.404×10−8+1.6675×10−8=0.2635×10−8J≈2.635×10−9J
(a) The gravitational potential energy of the two-sphere system just as B is released is approximately −1.404×10−8J.
Topper's Solved these Questions
- GRAVITATIONBOOK - RESNICK AND HALLIDAYCHAPTER - GRAVITATIONEXERCISE - PRACTICE QUESTIONS (SINGLE CORRECT CHOICE TYPE)
16 Videos
- GRAVITATIONBOOK - RESNICK AND HALLIDAYCHAPTER - GRAVITATIONEXERCISE - PRACTICE QUESTIONS (MORE THAN ONE CORRECT CHOICE TYPE)
7 Videos
- GRAVITATIONBOOK - RESNICK AND HALLIDAYCHAPTER - GRAVITATIONEXERCISE - CHECKPOINT
5 Videos
- GEOMETRICAL OPTICS : REFRACTIONBOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFRACTIONEXERCISE - Practice questions(Integers)
4 Videos
- HEAT-MEASUREMENT AND TRANSFERBOOK - RESNICK AND HALLIDAYCHAPTER - HEAT-MEASUREMENT AND TRANSFEREXERCISE - PRACTICE QUESTIONS( INTEGER TYPE)
5 Videos
Similar Questions
Two spheres each of mass 104 kg are separated by a distance of 100 m. What will be the gravitationel potential energy of the system?
View SolutionA heavy point of mass 15 kg is placed at the origin of coordinate axis. Find the gravitational potential at a point located at x=5cm on X-axis.
View Solution
Knowledge Check
A sphere of mass 10 kg and radius 0.5 m rotates about a tangent. The moment of inertia of the sphere is
A5kgm2B2.7kgm2C3.5kgm2D4.5kgm2A sphere of mass 10 kg and radius 0.5 m rotates about a tangent. The moment of inertia of the sphere is
A5 kg m2B2.7 kg m2C3.5 kg m2D4.5 kg m2An iron sphere of mass 10 kg has the same diameter as an aluminium sphere of mass 3.5 kg. Both spheres are dropped simultaneously from a tower. When they are 10 m above the ground, they have the same
AaccelerationBmomentaCpotential energyDkinetic energy
Calculate the moment of inertia of a solid sphere of mass 10 kg and radius 0.5 m, rotating about an axis 0.2 m from the centre of the sphere .
View SolutionA sphere centered at the origin has radius 0.200m.A−500μC point charge is on the x-axis at x=0.300m. The net flux through the sphere is 360N−m2/C. What is the total charge inside the sphere?
View SolutionA particle of mass 1kg is kept on the surface of a uniform sphere of mass 20kg and radius 1.0m. Find the work to be done against the gravitational force between them to take the particle away from the sphere.
View Solution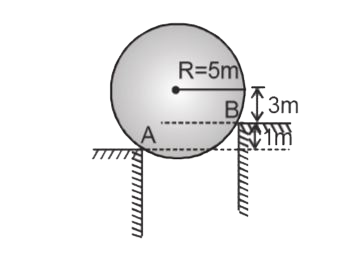
Find out the normal reaction at point A and B if the mass of sphere is 10kg.
View SolutionTwo spheres of masses 16 kg and 4 kg are separated by a distance 30 m on a table. Then, the distance from sphere of mass 16 kg at which the net gravitational force becomes zero is
View Solution
