The position vectors of the vertices A, B and C of a tetrahedron ABCD are ˆi+ˆj+ˆk, ˆk, ˆi and ˆ3i,respectively. The altitude from vertex D to the opposite face ABC meets the median line through Aof triangle ABC at a point E. If the length of the side AD is 4 and the volume of the tetrahedron is2/2/3, find the position vectors of the point E for all its possible positfons
The correct Answer is:−ˆi+3ˆj+3ˆk and 3ˆi−ˆj−ˆk
F is mid- piont of BC i.e., F=ˆi+3ˆi2=2ˆi and AE⊥DE
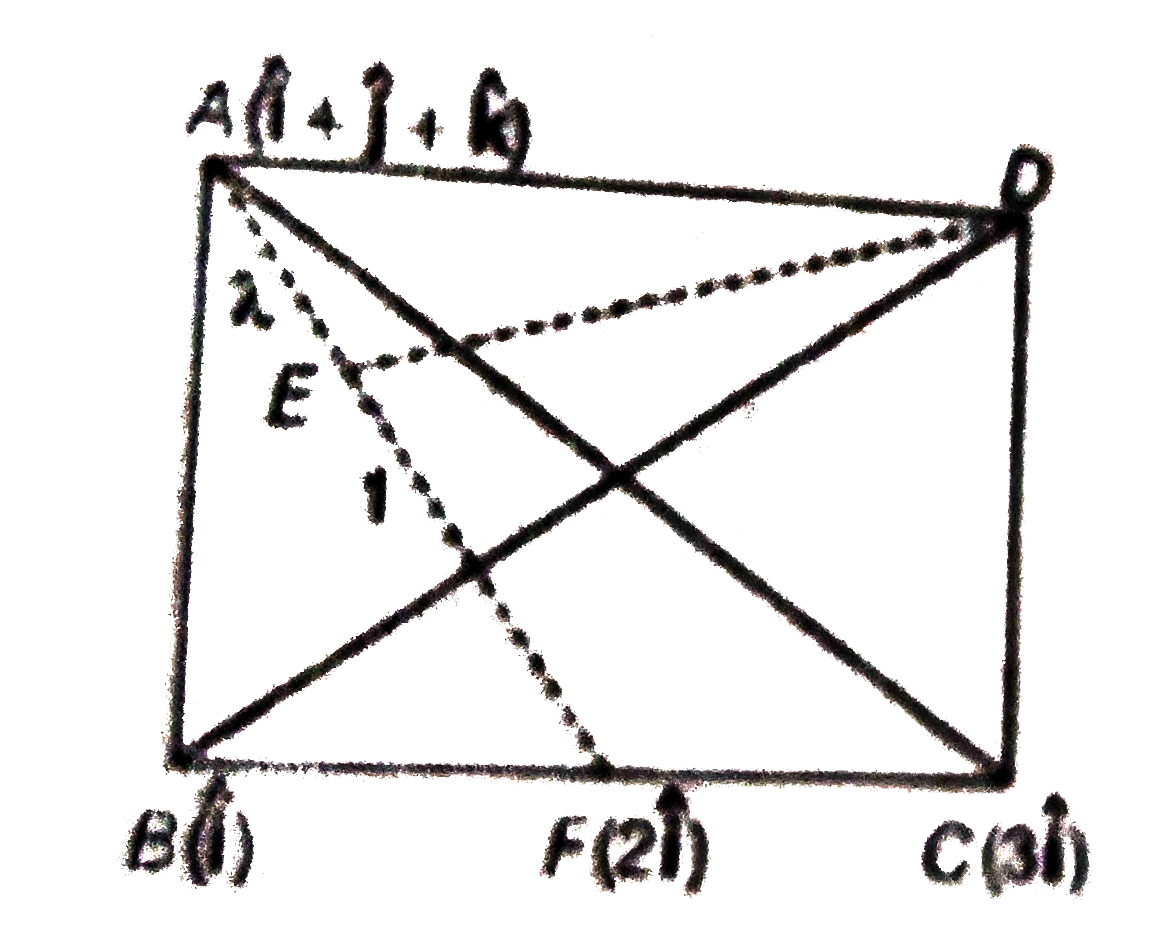
Let E divides AF in λ:1. The position vectors of E is given by
(2λˆi+1ˆi+ˆj+ˆkλ+1=(2λ+1λ+1)ˆi+1λ+1ˆj+1λ+1ˆk
Now volume of the tetrahedron
=13 (area of the bases ) (height)
⇒2√23=13(area of the ΔABC)(DE)
But area of the ΔABC=12∣∣∣−−→BC×−−→BA∣∣∣
=12∣∣2ˆi×(ˆj+ˆk)∣∣=∣∣ˆi׈j+ˆi׈k∣∣=∣∣ˆk−ˆj∣∣=√2
∴2√23=13(√2)(DE)⇒DE=2
Since ΔADE is a right angle triangle then
⇒AD2=AE2+DE2
But −−→AE=2λ+1λ+1ˆi+1λ+1ˆj+1λ+1ˆk−(ˆi+ˆj+ˆk)
=λλ+1ˆi−λλ+1ˆj−λλ+1ˆk
⇒∣∣∣−−→AE∣∣∣2=1(λ+1)2[λ2+λ2+λ2]=3λ2(λ+1)2
Theerefore 12=3λ2(λ+1)2
⇒4(λ+1)2=λ2⇒4λ2+4+δλ=λ2
⇒3λ2+δλ+4=0⇒3λ2+6λ+2λ+4=0
⇒3λ(λ+2)+2(λ+2)=0
⇒(3λ+2)(λ+2)=0⇒λ−2/3.λ=−2
when λ=−2/3 position vectors of E is given by
(2λ+1λ+1)ˆi+1λ+1ˆj+1λ+1ˆk
=2.(−2/3)+1−2/3+1ˆi+1−2/3+1ˆj+1−2/3+1ˆk
=−4/3+1−2+33ˆi+1−2+33ˆj+1−2+33ˆk
=−4+331/3ˆi+11/3ˆj+11/3ˆk=−ˆi+3ˆj+3ˆk
and when λ=−2, position vector of E is given by
2×(−2)+1−2+1ˆi+1−2+1ˆj+1−2+1ˆk=−4+1−1ˆi−ˆj−ˆk
=3ˆi−ˆj−ˆk
Therefore −ˆi+3ˆj+3ˆk and +3ˆi−ˆj−ˆk are the answer.
Topper's Solved these Questions
- VECTOR ALGEBRABOOK - IIT JEE PREVIOUS YEARCHAPTER - VECTOR ALGEBRAEXERCISE - Solving Equations and Reciprocal of Vectors (Objective Questions II) (Fill in the Blanks)
4 Videos
- TRIGONOMETRICAL RATIOS AND IDENTITIESBOOK - IIT JEE PREVIOUS YEARCHAPTER - TRIGONOMETRICAL RATIOS AND IDENTITIESEXERCISE - HEIGHT AND DISTANCE
10 Videos
Similar Questions
The position vectors of the vertices A,B,andC of a triangle are ˆi+ˆj,ˆj+ˆkandˆi+ˆk , respectively. Find the unite vector ˆr lying in the plane of ABC and perpendicular to IA,whereI is the incentre of the triangle.
View SolutionIf the position vectors of the points A, B, C are −2ˆi+2ˆj+2ˆk,2ˆi+3ˆj+3ˆkand−ˆi2ˆj+3ˆk respectively, show that ABC is an isosceles triangle.
View Solution
Knowledge Check
The position vectors of vertices of a ΔABC are 4ˆi−2ˆj,ˆi−3ˆk and −ˆi+5ˆj+ˆk respectively, then ∠ABC is equal to
Aπ6Bπ4Cπ3Dπ2The position vectors of vertices of a ΔABC are 4ˆi−2ˆj,ˆi−3ˆk and −ˆi+5ˆj+ˆk respectively, then ∠ABC is equal to
Aπ6Bπ4Cπ3Dπ2The position vectors of the vectices A, B, C of a △ABC are ˆi−ˆj−3ˆk,2ˆi+ˆj−2ˆkand−5ˆi+2ˆj−6ˆk
respectively. The length of the bisector AD of the angle ∠BAC where D is on the line segment BC, isA152B112C14Dnone of these
The position vectors of the angular points of a tetrahedron are A(3ˆi−2ˆj+ˆk),B(3ˆi+ˆj+5ˆk),C(4ˆi+3ˆk) and D(ˆi) Then the acute angle between the lateral face ADC and the base face ABC is:
View SolutionThe position vectors of the four angular points of a tetrahedron are A(ˆj+2ˆk),B(3ˆi+ˆk),C(4ˆi+3ˆj+6ˆk)andD(2ˆi+3ˆj+2ˆk). Find the volume of the tetrahedron ABCD.
View SolutionIf the vertices of a tetrahedron have the position vectors →0,ˆi+ˆj,2ˆj−ˆkandˆi+ˆk then the volume of the tetrahedrom is
View SolutionIf position vectors of two points A and B are 3ˆi−2ˆj+ˆkand2ˆi+4ˆj−3ˆk, respectively then length of −−→AB is equal to?
View SolutionThe adjacent sides AB and AC of a triangle ABC are represented by the vectors −2ˆi+3ˆj+2ˆkand−4ˆi+5ˆj+2ˆk respectively. The area of the triangle ABC is
View Solution