If S is a set of triangles whose one vertex is origin and other two vertices are integral coordinates and lies on coordinate axis of area 50 square units, then number of elements in set S is equal to (a) 9 (b) 18 (c) 36 (d) 40
36
32
18
9
The correct Answer is:A
According to given information, we have the following figure.
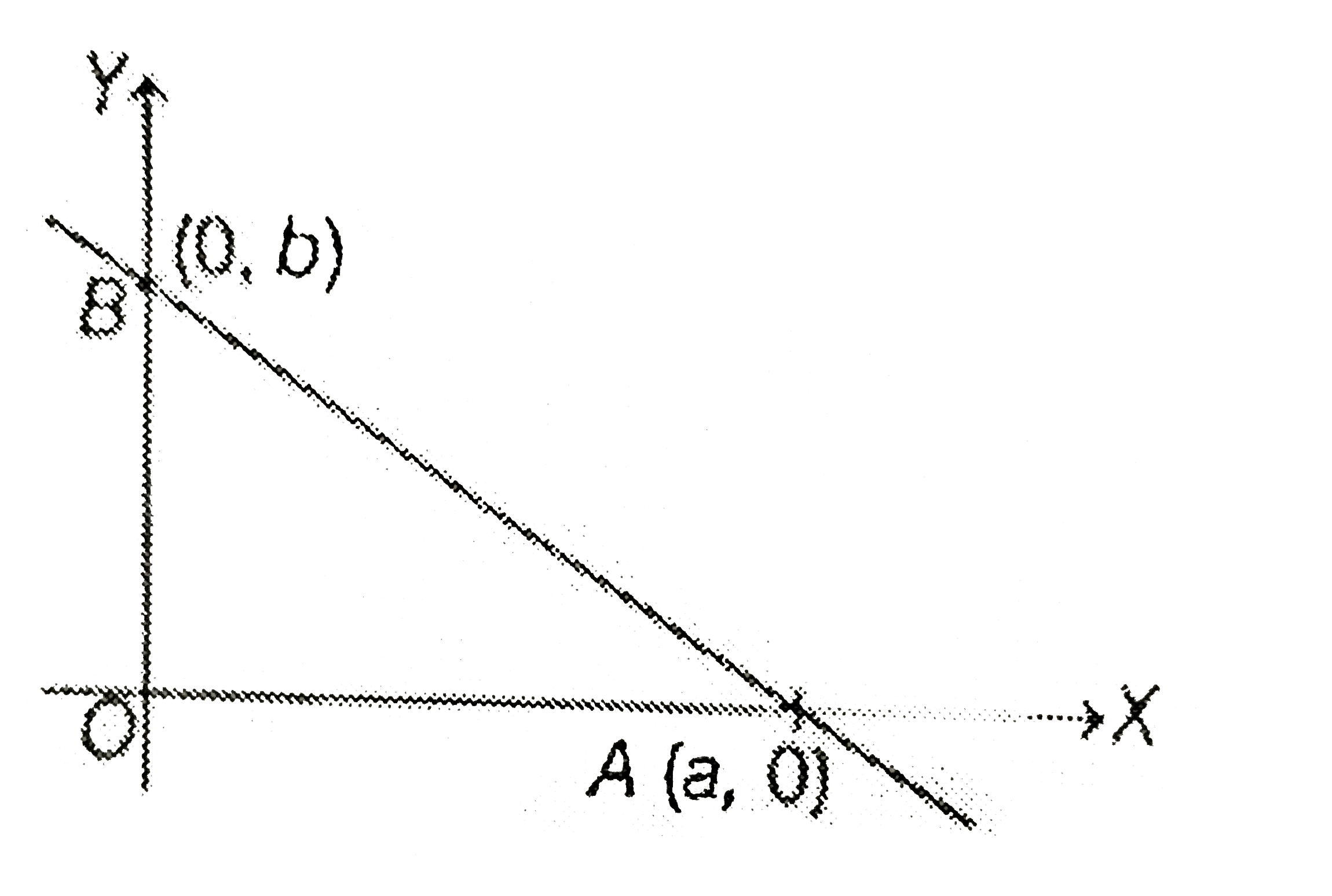
(Note that as a and b are integers so they can be negative also). Here O(0, 0), A(a, 0) and B(0, b) are the three vertices of the triangle.
Clearly, OA=|a| and OB=|b|.
∴ Area of ΔOAB=12|a||b|.
But area of such triangles is given as 50 sq units.
∴ 12|a||b|=50
⇒ |a||b|=100=22⋅52
Number of ways of distributing two 2's in |a| and |b|=3
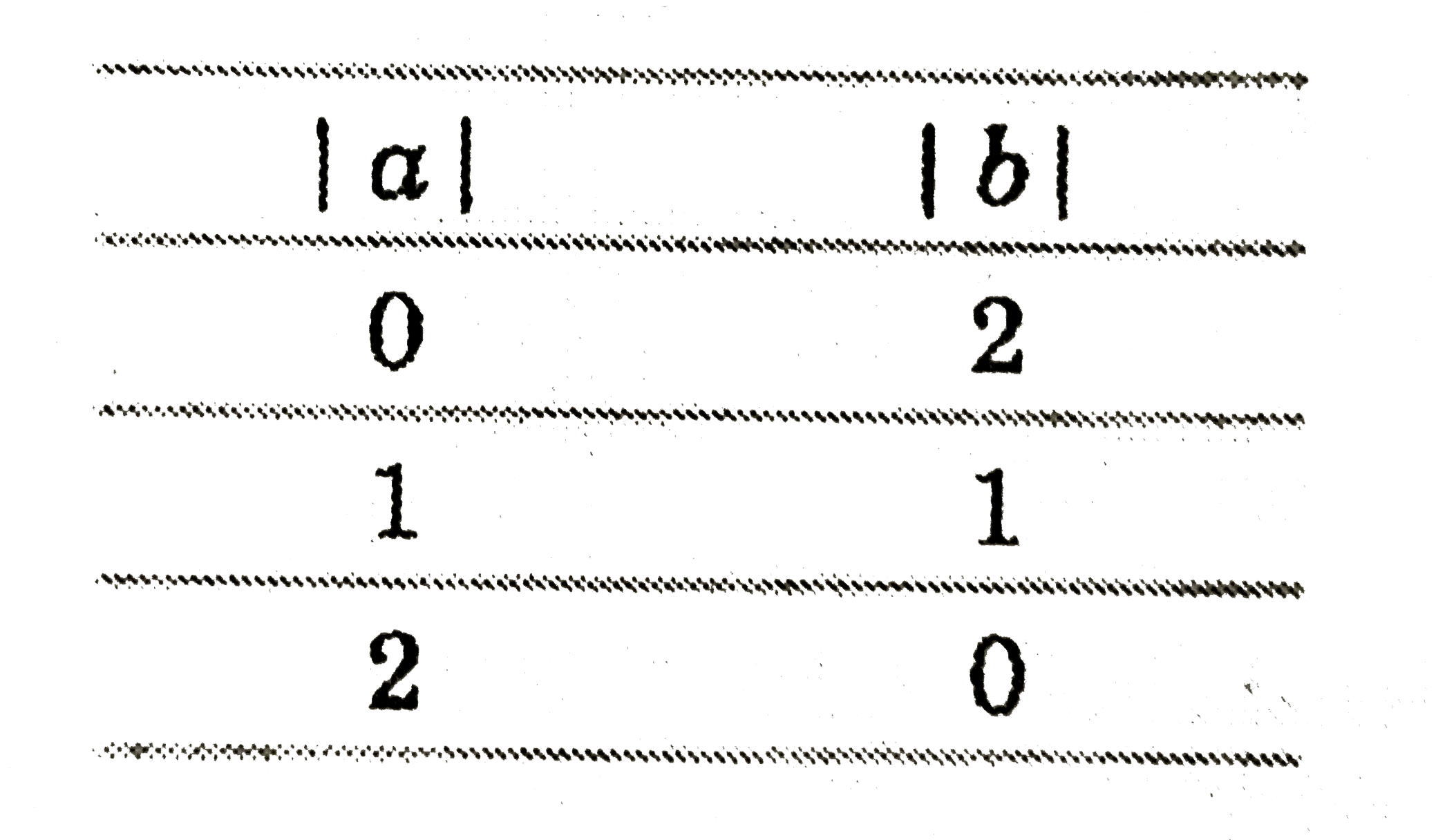
⇒3 ways
Similarly, number of ways of distributing two 5's in |a| and |b| = 3 ways.
∴ Total number of ways of distributing 2's and 5's =3×3=9 ways
Note that for one value of |a|, there are 2 possible values of a and for one value of |b| there are 2 possible values of b.
∴ Number of such triangles possible =2×2×9=36.
So, number of elements in S is 36.
Topper's Solved these Questions
- PERMUTATIONS AND COMBINATIONSBOOK - IIT JEE PREVIOUS YEARCHAPTER - PERMUTATIONS AND COMBINATIONSEXERCISE - Distribution of Object into Group (Analytical & Descriptive )
2 Videos
- PERMUTATIONS AND COMBINATIONSBOOK - IIT JEE PREVIOUS YEARCHAPTER - PERMUTATIONS AND COMBINATIONSEXERCISE - Dearrangement and Number of Divisors (Ojective Question I Only one correct option )
1 Videos
- PERMUTATIONS AND COMBINATIONSBOOK - IIT JEE PREVIOUS YEARCHAPTER - PERMUTATIONS AND COMBINATIONSEXERCISE - Multinomial, Repeated Arrangement and Selection (Analytical & Descriptive )
1 Videos
- PARABOLABOOK - IIT JEE PREVIOUS YEARCHAPTER - PARABOLAEXERCISE - TOPIC 4 DIAMETER, CHORD OF CONTACT, CHORD BISECTED AND PRODUCT OF PAIR OF TANGENTS OBJECTIVES QUESTION II (ANALYTICAL & DESCRIPTIVE )
5 Videos
- PROBABILITYBOOK - IIT JEE PREVIOUS YEARCHAPTER - PROBABILITYEXERCISE - TOPIC 5 PROBABILITY DISTRIBUTION AND BINOMIAL DISTRIBUTTION OBJECTIVE QUESTIONS ONLY ONE CORRECT OPTION (INTEGER )
1 Videos
Similar Questions
Let "S" be the set of all triangles in the xy-plane,each having one vertex at the origin and the other two vertices lie on coordinate axes with integral coordinates .If each triangle in "S" has area "60" sq.units,then the number of elements in the set "S" are
View SolutionIf the area of the triangle whose one vertex is at the vertex of the parabola, y2+4(x−a2)=0 and the other two vertices are the points of intersection of the parabola and Y-axis, is 250 sq units, then a value of 'a' is
View Solution
Knowledge Check
Let S be the set of all triangles in the xy-plane, each having one vertex at the origin and the other two vertices lie on coordinate axes with integral coordinates. If each triangle in S has area 50 eq. units, then the number of elements in the set S is
A9B18C32D36The centroid of a triangle lies at the origin and the coordinates of its two vertices are (−8,0) and (9, 11), the area of the triangle in sq. units is
A11/8B8/11C88Dnone of theseThe centroid of a triangle lies at the origin and the coordinates of its two vertices are (−8,7) and (9, 4). The area of the triangle is
A95/6B285/2C190/3D285
Two sides of a triangle are along the coordinate axes and the medians through the vertices (other than the origin) are mutually perpendicular. The number of such triangles is/are zero (b) two (c) four (d) infinite
View SolutionIf a plane cuts off intercepts OA=a,OB=b,OC=c from the coordinate axes 9where 'O' is the origin). then the area of the triangle ABC is equal to
View SolutionTwo isosceles triangles have equal vertical angles and their corresponding sides are in the ratio 3:5. What is the ratio of their areas?
(a)3:5
(b) 6:10
(c) 9:25
(d) None of these
View SolutionThe area of a triangle is 3 square units. Two of its vertices are A(3,1),B(1,−3) and the centroid of the triangle lies on x-axis. Find the coordinates of the third vertex C.
View SolutionTwo vertices of triangle are (-2,-1) and (3,2) and third vertex lies on the line x+y=5. If the area of the triangle is 4 square units, then the third vertex is a. (-0,50 or (4,1) b. (5,0) of (1,4) c. (5,0) or (4,1) d. (o,5) or (1,4)
View Solution
