A pair of dice is thrown 4 times. If getting a doublet is considered a success, find the probability distribution of number of successes. Also, find the mean and variance of number of successes.

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $(# # R S A_{M} A T H_{X} I I_{C} 31_{E} 01_{006}_A 01 # #)$
$M e a n = \frac{2}{3}, v a r i a n c e = \frac{5}{9}$

In a single throw, P(doublet) $= \frac{6}{36} = \frac{1}{6},$ and P(non-doublet) $= (1 - \frac{1}{6} = \frac{5}{6} .$
Let X be the number of doublets. Then, X = 0, 1, 2 or 3.
P(X=0)= P (non-doublet in each case)
$= P ({\bar{D}}_{1} {\bar{D}}_{2} {\bar{D}}_{3} {\bar{D}}_{4}) = (\frac{5}{6} \times \frac{5}{6} \times \frac{5}{6} \times \frac{5}{6}) = \frac{625}{1296} .$
P(X=1)=P(one doublet)
$= P (D_{1} {\bar{D}}_{2} {\bar{D}}_{3} {\bar{D}}_{4}) or P ({\bar{D}}_{1} D_{2} {\bar{D}}_{3} {\bar{D}}_{4})$
$or P ({\bar{D}}_{1} {\bar{D}}_{2} D_{3} {\bar{D}}_{4}) or P ({\bar{D}}_{1} D_{2} {\bar{D}}_{3} D_{4})$
$= (\frac{1}{6} \times \frac{5}{6} \times \frac{5}{6} \times \frac{5}{6}) + (\frac{5}{6} \times \frac{1}{6} \times \frac{5}{6} \times \frac{5}{6}) + (\frac{5}{6} \times \frac{5}{6} \times \frac{1}{6} \times \frac{5}{6}) + (\frac{5}{6} \times \frac{5}{6} \times \frac{5}{6} \times \frac{1}{6})$
$= (4 \times \frac{125}{1296}) = \frac{125}{324} .$
P(X=2)=P(two doublets)
$= P (D_{1} D_{2} {\bar{D}}_{3} {\bar{D}}_{4}) or P (D_{1} {\bar{D}}_{2} D_{3} {\bar{D}}_{4}) or P (D_{1} {\bar{D}}_{2} {\bar{D}}_{3} D_{4}) or P ({\bar{D}}_{1} D_{2} D_{3} {\bar{D}}_{4}) or P ({\bar{D}}_{1} D_{2} {\bar{D}}_{3} D_{4}) or P ({\bar{D}}_{1} {\bar{D}}_{2} D_{3} D_{4})$
$= (\frac{1}{6} \times \frac{1}{6} \times \frac{5}{6} \times \frac{5}{6}) + (\frac{1}{6} \times \frac{5}{6} \times \frac{1}{6} \times \frac{5}{6}) + (\frac{1}{6} \times \frac{5}{6} \times \frac{5}{6} \times \frac{1}{6}) + (\frac{5}{6} \times \frac{1}{6} \times \frac{1}{6} \times \frac{5}{6}) + (\frac{5}{6} \times \frac{1}{6} \times \frac{5}{6} \times \frac{1}{6}) + (\frac{5}{6} \times \frac{5}{6} \times \frac{1}{6} \times \frac{1}{6})$
$(6 \times \frac{25}{1296}) = \frac{25}{216} .$
P(X=3)=P(three doublets)
$P (D_{1} D_{2} D_{3} {\bar{D}}_{4} or P (D_{1} D_{2} {\bar{D}}_{3} D_{4}) or P (D_{1} {\bar{D}}_{2} D_{3} D_{4}) or P ({\bar{D}}_{1} D_{2} D_{3} D_{4})$
$= (\frac{1}{6} \times \frac{1}{6} \times \frac{1}{6} \times \frac{5}{5}) + (\frac{1}{6} \times \frac{1}{6} \times \frac{5}{6} \times \frac{1}{6}) + (\frac{1}{6} \times \frac{5}{6} \times \frac{1}{6} \times \frac{1}{6}) + (\frac{5}{6} \times \frac{1}{6} \times \frac{1}{6} \times \frac{1}{6})$
$= (4 \times \frac{5}{1296}) = \frac{5}{324} .$
$P (X = 4) = P (four doublets) = P (D_{1} D_{2} D_{3} D_{4})$
$= (\frac{1}{6} \times \frac{1}{6} \times \frac{1}{6} \times \frac{1}{6}) = \frac{1}{1296} .$
Thus, we have

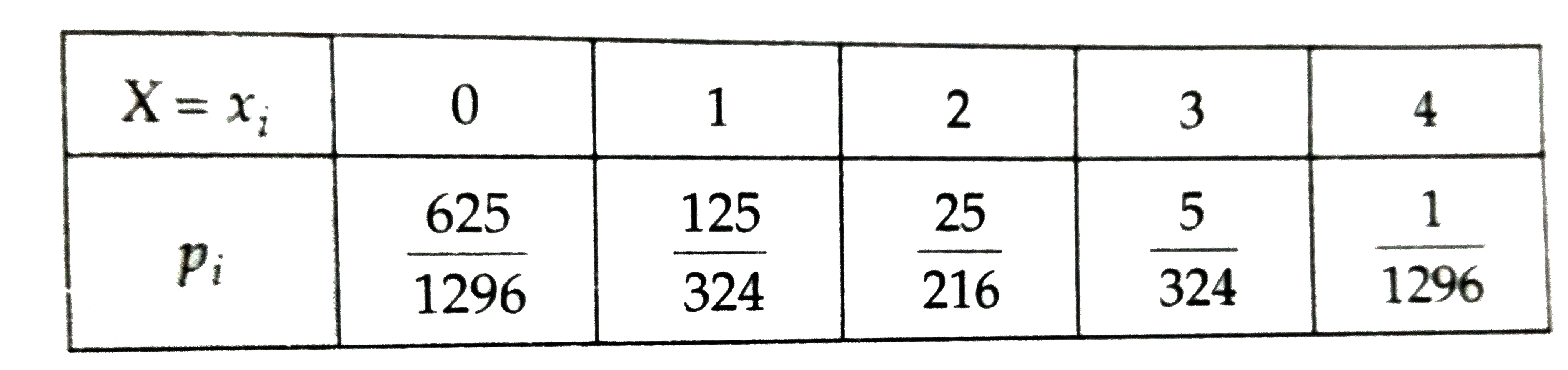
Now, find $μ and σ^{2} .$

|

Updated on:21/07/2023

Class 12 MATHS PROBABILITY

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A die is thrown 2 times. If getting an odd number is considered as a success, then the probability of 2 successes is
A $\frac{1}{2}$
B $\frac{3}{4}$
C $\frac{2}{3}$
D $\frac{1}{4}$
Question 2 - Select One
A die is thrown 10 times. If getting an even number is considered as a success, then the probability of four successes is
A $^{10} C_{4} {(\frac{1}{2})}^{4}$
B $^{10} C_{4} {(\frac{1}{2})}^{6}$
C $^{10} C_{4} {(\frac{1}{2})}^{8}$
D $^{10} C_{6} {(\frac{1}{2})}^{10}$

RS AGGARWAL-PROBABILITY DISTRIBUTION-Exercise 31

Home
Ask Doubt
Profile