Show that the angular momentum about any point of a single particle moving with constant velocity remains constant throughout the motion.Solution in Telugu
Let the particle with velocity v be at point P at some instant t. We want to calculate the angular momentum of the particle about an arbitrary point O.
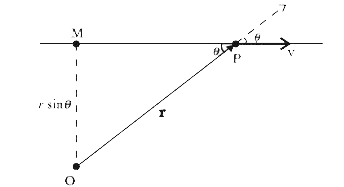
The angular momentum is l=r×mv. Its magnitude is mvrsinθ , where θ is the angle between r and v as shown in Fig. 7.19. Although the particle changes position with time, the line of direction of v remains the same and hence OM=rsinθ . is a constant.
Further, the direction of l is perpendicular to the plane of r and v. It is into the page of the figure.This direction does not change with time.
Thus, l remains the same in magnitude and direction and is therefore conserved. Is there any external torque on the particle?
Topper's Solved these Questions
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - NCERT TELUGUCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - EXERCISES
29 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - NCERT TELUGUCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - EXERCISES (TRUE OR FALSE)
5 Videos
- OSCILLATIONSBOOK - NCERT TELUGUCHAPTER - OSCILLATIONSEXERCISE - Additional Exercises
6 Videos
- THERMAL PROPERTIME OF MATTERBOOK - NCERT TELUGUCHAPTER - THERMAL PROPERTIME OF MATTEREXERCISE - EXERCISES
30 Videos
Similar Questions
Give the location of the centre of mass of a (i) sphere, (ii) cylinder, (iii) ring, and (iv) cube, each of uniform mass density. Does the centre of mass of a body necessarily lie inside the body ?
View SolutionDuring rolling, the force of friction acts in the same direction as the direction of motion of the CM of the body.
View SolutionAn air chamber of volume V has a neck area of cross section a into which a ball of mass m just fits and can move up and down without any friction (Fig.14.27). Show that when the ball is pressed down a little and released , it executes SHM. Obtain an expression for the time period of oscillations assuming pressure-volume variations of air to be isothermal [see Fig. 14.27].

View SolutionThe triple points of neon and carbon dioxide are 24.57K and 216.55K respectively. Express these temperatures on the Celsius and Fahrenheit scales.
View SolutionAssertion: If linear momentum of a particle is constant, then its angular momentum about any point will also remain constant. Reason: Linear momentum remains constant if F_(n et)=0 and angular momentum remains constant if tau_(n et)=0
View SolutionShow that the angular momentum about any point of a single particle moving with constant velocity remains constant throughout the motion.
View SolutionAssertion : If a particle moves with a constant velocity, then angular momentum of this particle about any point remains constant. Reason : Angular momentum has the units of Plank's constant.
View Solutionदर्शाइये, कि अचर -वेग से चलते एकल कण का किसी बिंदु के परितः कोणीय संवेग उसकी समस्त गति के दौरान अचर रहता है ।
View Solutionदर्शाइए कि अचर वेग से चलते हुए एकल कण का किसी बिंदु के परितः कोणीय संवेग उसकी समस्त गति के दौरान अचर रहता है ।
View SolutionA particle of mass (m) is moving with constant velocity (v). Show that its angular momentum about any point renains constant throughout the motion.
View SolutionShow that the angular momentum about any point of a single particle moving with constant velocity remains constant throughout the motion.
View SolutionShow that the angular momentum about any point of a single particle moving with constant velocity remains constant throughout the motion.
View SolutionShow that the angular momentum about any point of a single particle moving with constant velocity remains constant throughout the motion.
View SolutionShow that the angular momentum about any point of a single particle moving with constant velocity remains constant throughout the motion.
View SolutionShow that the angular momentum about any point of a single particle moving with constant velocity remains constant throughout the motion. Is there any external torque on the particle ?
View Solution
