The probabilities of four cricketers A, B, C and D scoring more than 50 runs in a match are 12,13,14 and 110. It is known that exactly two of the players more than 50 runs in a particular match. The probability that these players were A and B is
2765
56
16
None of these
The correct Answer is:A
To solve the problem, we need to find the probability that cricketers A and B are the ones who scored more than 50 runs, given that exactly two players scored more than 50 runs.
1. Identify the Probabilities:
- Let P(A)=12 (Probability that A scores more than 50 runs)
- Let P(B)=13 (Probability that B scores more than 50 runs)
- Let P(C)=14 (Probability that C scores more than 50 runs)
- Let P(D)=110 (Probability that D scores more than 50 runs)
2. Calculate the Probabilities of Not Scoring More than 50 Runs:
- P(A′)=1−P(A)=1−12=12
- P(B′)=1−P(B)=1−13=23
- P(C′)=1−P(C)=1−14=34
- P(D′)=1−P(D)=1−110=910
3. Identify the Possible Pairs of Players:
The pairs of players who can score more than 50 runs are:
- (A, B)
- (A, C)
- (A, D)
- (B, C)
- (B, D)
- (C, D)
4. Calculate the Probability for Each Pair:
- For A and B:
P(A and B)=P(A)⋅P(B)⋅P(C′)⋅P(D′)=12⋅13⋅34⋅910
=1⋅1⋅3⋅92⋅3⋅4⋅10=27240
- For A and C:
P(A and C)=P(A)⋅P(C)⋅P(B′)⋅P(D′)=12⋅14⋅23⋅910
=1⋅1⋅2⋅92⋅4⋅3⋅10=18240
- For A and D:
P(A and D)=P(A)⋅P(D)⋅P(B′)⋅P(C′)=12⋅110⋅23⋅34
=1⋅1⋅2⋅32⋅10⋅3⋅4=6240
- For B and C:
P(B and C)=P(B)⋅P(C)⋅P(A′)⋅P(D′)=13⋅14⋅12⋅910
=1⋅1⋅1⋅93⋅4⋅2⋅10=9240
- For B and D:
P(B and D)=P(B)⋅P(D)⋅P(A′)⋅P(C′)=13⋅110⋅12⋅34
=1⋅1⋅1⋅33⋅10⋅2⋅4=3240
- For C and D:
P(C and D)=P(C)⋅P(D)⋅P(A′)⋅P(B′)=14⋅110⋅12⋅23
=1⋅1⋅1⋅24⋅10⋅2⋅3=2240
5. Total Probability of Exactly Two Players Scoring More than 50 Runs:
P(exactly 2 players)=P(A and B)+P(A and C)+P(A and D)+P(B and C)+P(B and D)+P(C and D)
=27240+18240+6240+9240+3240+2240=65240
6. Calculate the Required Probability:
The probability that the two players who scored more than 50 runs are A and B is given by:
P(A and B|exactly 2 players)=P(A and B)P(exactly 2 players)
=2724065240=2765
Final Answer:
The probability that the players who scored more than 50 runs were A and B is 2765.
- Let P(A)=12 (Probability that A scores more than 50 runs)
- Let P(B)=13 (Probability that B scores more than 50 runs)
- Let P(C)=14 (Probability that C scores more than 50 runs)
- Let P(D)=110 (Probability that D scores more than 50 runs)
- P(A′)=1−P(A)=1−12=12
- P(B′)=1−P(B)=1−13=23
- P(C′)=1−P(C)=1−14=34
- P(D′)=1−P(D)=1−110=910
The pairs of players who can score more than 50 runs are:
- (A, B)
- (A, C)
- (A, D)
- (B, C)
- (B, D)
- (C, D)
- For A and B:
P(A and B)=P(A)⋅P(B)⋅P(C′)⋅P(D′)=12⋅13⋅34⋅910
=1⋅1⋅3⋅92⋅3⋅4⋅10=27240
P(A and C)=P(A)⋅P(C)⋅P(B′)⋅P(D′)=12⋅14⋅23⋅910
=1⋅1⋅2⋅92⋅4⋅3⋅10=18240
P(A and D)=P(A)⋅P(D)⋅P(B′)⋅P(C′)=12⋅110⋅23⋅34
=1⋅1⋅2⋅32⋅10⋅3⋅4=6240
P(B and C)=P(B)⋅P(C)⋅P(A′)⋅P(D′)=13⋅14⋅12⋅910
=1⋅1⋅1⋅93⋅4⋅2⋅10=9240
P(B and D)=P(B)⋅P(D)⋅P(A′)⋅P(C′)=13⋅110⋅12⋅34
=1⋅1⋅1⋅33⋅10⋅2⋅4=3240
P(C and D)=P(C)⋅P(D)⋅P(A′)⋅P(B′)=14⋅110⋅12⋅23
=1⋅1⋅1⋅24⋅10⋅2⋅3=2240
P(exactly 2 players)=P(A and B)+P(A and C)+P(A and D)+P(B and C)+P(B and D)+P(C and D)
=27240+18240+6240+9240+3240+2240=65240
The probability that the two players who scored more than 50 runs are A and B is given by:
P(A and B|exactly 2 players)=P(A and B)P(exactly 2 players)
=2724065240=2765
The probability that the players who scored more than 50 runs were A and B is 2765.
Topper's Solved these Questions
- PROBABILITY -2BOOK - DISHA PUBLICATIONCHAPTER - PROBABILITY -2EXERCISE - EXERCISE - 1 : CONCEPT BUILDER
60 Videos
- PRINCIPLE OF MATHEMATICAL INDUCTIONBOOK - DISHA PUBLICATIONCHAPTER - PRINCIPLE OF MATHEMATICAL INDUCTIONEXERCISE - Exercise-2 Concept Applicator
20 Videos
- PROBABILITY-1BOOK - DISHA PUBLICATIONCHAPTER - PROBABILITY-1EXERCISE - EXERCISE-1 : CONCEPT BUILDER
180 Videos
Similar Questions
The probability of scoring a century in a cricket match by a player is 3/4 if he practices before the match and it is 1/3 if he doesn't practice before the match. The probability that a player attends practice session before the match is 1/3. If it is known that a player scored century in a particular match, the probability that he didn't go for match practice before the match, is.
(A) 3/17 (B) 4/17 (C) 6/17 (D) 8/17
View SolutionThe probability an event of a trial is 1 (b) 0 (c) less than 1 (d) more than 1
View Solution
Knowledge Check
In a one day match, a player played 40 balls. The runs scored are as follows :
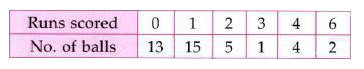
Find the probability that player hits a four or a six.A3/20B1/2C1/4D9/20The probability of occurrence of two events A and B are 1/4 and 1/2 respectively . The probability of their simultaneous occurrence is 750., find the probability that neither A nor B occurs .
A2599B39100C61100D17100Which of the following cricket players has surpassed Don Bradman as the record for the most runs scored as a captain in international cricket by more than 150 runs?
AVirat KohliBFaf du PlessisCJoe RootDKen Williamson
The probability of a certain event is 0 (b) 1 (c) greater than 1 (d) less than 0
View SolutionTwo players A and B play a match. Which consists of a series of games (independent). Whoever first wins two games not necessarily consecutive, wins the match. The probability of A's winning, drawing, losing a game against B are 12,13,16 respectively. It is known that A won the match at the end of 11th game, the probability that B wins only one game is
(A) 311 (B) 811 (C) 911 (D) 1011
View SolutionThe probability of an impossible event is 1 (b) 0 (c) less than 0 (d) greater than 1
View SolutionTwo dice are thrown. If it is known that the sum of the numbers on the dice was less than 6, the probability of getting a sum 3 is
View SolutionThe probability of A to fail in an examination is 15 and that of B is 310. If the probability that both of them fails is 350, then the probability that only one of A and B fails, is
View Solution
