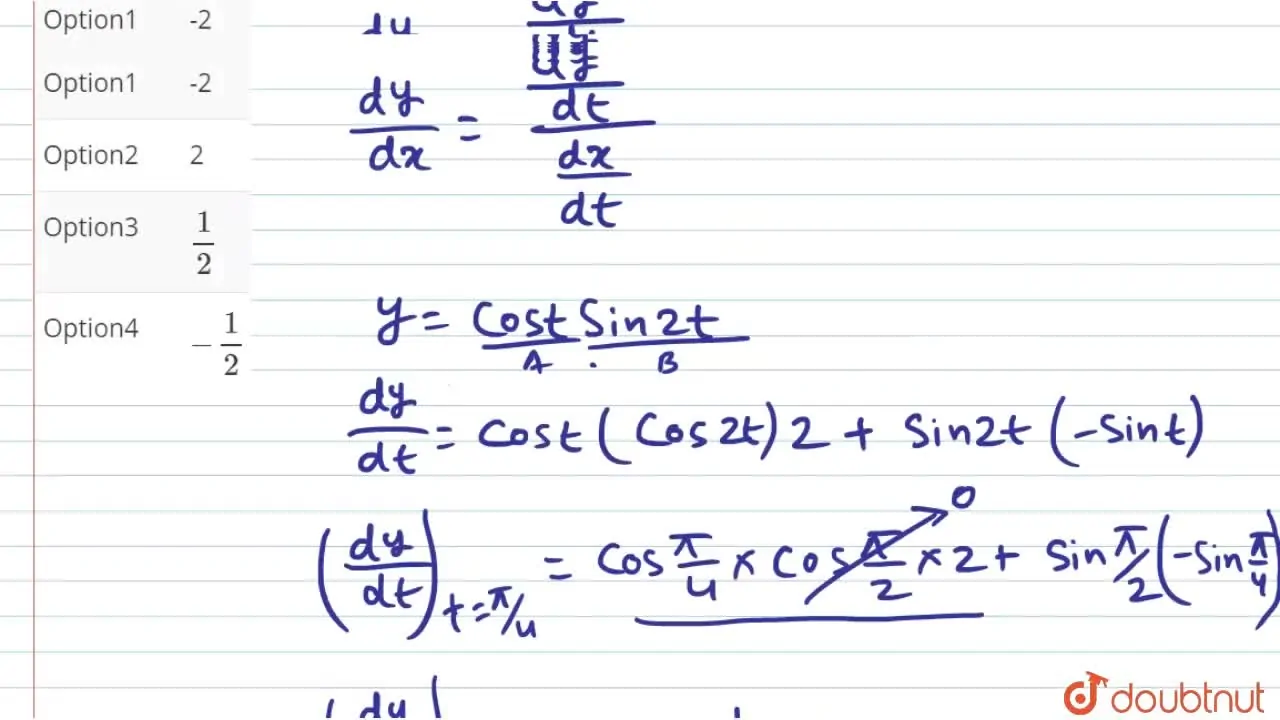If x=sintcos2t and y=costsin2t , then at t=π4, the value of dydx is equal to:
-2
2
12
−12
The correct Answer is:C
Step by step video, text & image solution for If x = sint cos2t and y = cost sin2t , then at t = pi/4, the value of dy/dx is equal to: by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - DISHA PUBLICATIONCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Exercise-2 : Concept Applicator30videos
CONTINUITY AND DIFFERENTIABILITY
- BOOK - DISHA PUBLICATIONCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Exercise-2 : Concept Applicator30videos
CONTINUITY AND DIFFERENTIABILITY
- BOOK - DISHA PUBLICATIONCHAPTER - CONIC SECTIONSEXERCISE - Exercise-2 : Concept Applicator30videos
CONIC SECTIONS
- BOOK - DISHA PUBLICATIONCHAPTER - DETERMINANTSEXERCISE - EXERCISE -2 CONCEPT APPLICATOR30videos
DETERMINANTS
Similar Questions
If y = cos t and x = sin t, then what is the value of dy/dx ?
View SolutionIf x=sintcos2t,y=costsin2t,then at t=π4,dydx
View SolutionIf x=2cost−cos2t,y=2sint−sin2t,then at t=π4,dydx=
View SolutionIf t(1+x2)=xandx2+t2=y, then at x=2 the value of dydx is equal to
View SolutionIf x=sint and y=sin3t, then d2ydx2 at t=π2 is
View SolutionIf x = sint - t cost and y = t sin t + cos t, then what is dy/dx at point t=π2 ?
View SolutionIf x=asin2t(1+cos2t) and y=bcos2t(1−cos2t), find the values of dydx at t=π4 and t=π3.
View SolutionIf y=sint−cost and x=sint+cost, then dydx at t=π6 is :
View SolutionIf x=sint and y=cos2t, then dydx =
View SolutionIf x=2sint−sin2t,y=2cost−cos2t, then the value of d2ydx2 at t=π2 is
View Solution