The area of ΔABC is 44 cm2 . If D is the midpoint of BC and E is the midpoint of AB, then the area ( in cm2) of ΔBDE is :
5.5
22
11
44
The correct Answer is:C
To find the area of triangle BDE given that the area of triangle ABC is 44 cm², and D and E are midpoints of sides BC and AB respectively, we can follow these steps:
Step 1: Understand the triangle and midpoints
We have triangle ABC with area 44 cm². D is the midpoint of side BC, and E is the midpoint of side AB.
Hint: Remember that midpoints divide the segments into equal halves.
Step 2: Divide triangle ABC into two smaller triangles
Since D is the midpoint of BC, line AD divides triangle ABC into two smaller triangles: ABD and ACD. Because D is the midpoint, the areas of triangles ABD and ACD are equal.
Calculation:
Area of triangle ABD = Area of triangle ACD = Total area of triangle ABC / 2
Area of triangle ABD = 44 cm² / 2 = 22 cm²
Hint: When a line is drawn from a vertex to the midpoint of the opposite side, it divides the triangle into two triangles of equal area.
Step 3: Analyze triangle ABD
Now, we focus on triangle ABD. E is the midpoint of side AB. Therefore, line segment DE divides triangle ABD into two smaller triangles: ADE and BDE. Since E is the midpoint, triangles ADE and BDE also have equal areas.
Calculation:
Area of triangle ADE = Area of triangle BDE = Area of triangle ABD / 2
Area of triangle BDE = 22 cm² / 2 = 11 cm²
Hint: Midpoints also divide the area of triangles into equal parts.
Step 4: Conclusion
Thus, the area of triangle BDE is 11 cm².
Final Answer: The area of triangle BDE is 11 cm².
We have triangle ABC with area 44 cm². D is the midpoint of side BC, and E is the midpoint of side AB.
Since D is the midpoint of BC, line AD divides triangle ABC into two smaller triangles: ABD and ACD. Because D is the midpoint, the areas of triangles ABD and ACD are equal.
Area of triangle ABD = Area of triangle ACD = Total area of triangle ABC / 2
Area of triangle ABD = 44 cm² / 2 = 22 cm²
Now, we focus on triangle ABD. E is the midpoint of side AB. Therefore, line segment DE divides triangle ABD into two smaller triangles: ADE and BDE. Since E is the midpoint, triangles ADE and BDE also have equal areas.
Area of triangle ADE = Area of triangle BDE = Area of triangle ABD / 2
Area of triangle BDE = 22 cm² / 2 = 11 cm²
Thus, the area of triangle BDE is 11 cm².
Similar Questions
The area of △ABC is 44 cm2. If D is the midpoint of BC and E is the midpoint of AB, then the area (in cm2) of △BDE is:
△ABC का क्षेत्रफल 44 वर्ग सेमी है | यदि D, BC का मध्य बिंदु है तथा E, AB का मध्य बिंदु है, तो △BDE का क्षेत्रफल ( वर्ग सेमी में ) होगा :
View SolutionIn an equilateral ΔABC,D is the midpoint of AB and E is the midpoint of AC. Then, ar(ΔABC):ar(ΔADE)=?
View Solution
Knowledge Check
The area of △ABC is 88cm2. If D is the midpoint of BC and E is the midpoint of AB, then the area (in cm2) of △BDE is:
A44B22C11D5.5In triangle ABC,D is the midpoint of AB, E is the midpoint of DB and F is the midpoint of BC. If the area of ΔABC is 96, the area of ΔAEF is
A16B24C32D36In ΔABC,∠A=90∘,AB=16 cm and AC=12 cm=12 cm. D is the midpoint of AC and DE⊥CB at E. What is the area (in cm2) of Δ CDE ?
A8.64B7.68C5.76D6.25
ABC is a triangle in which D is the midpoint of BC and E is the midpoint of AD.
Prove that ar(△BED)=14ar(△ABC).
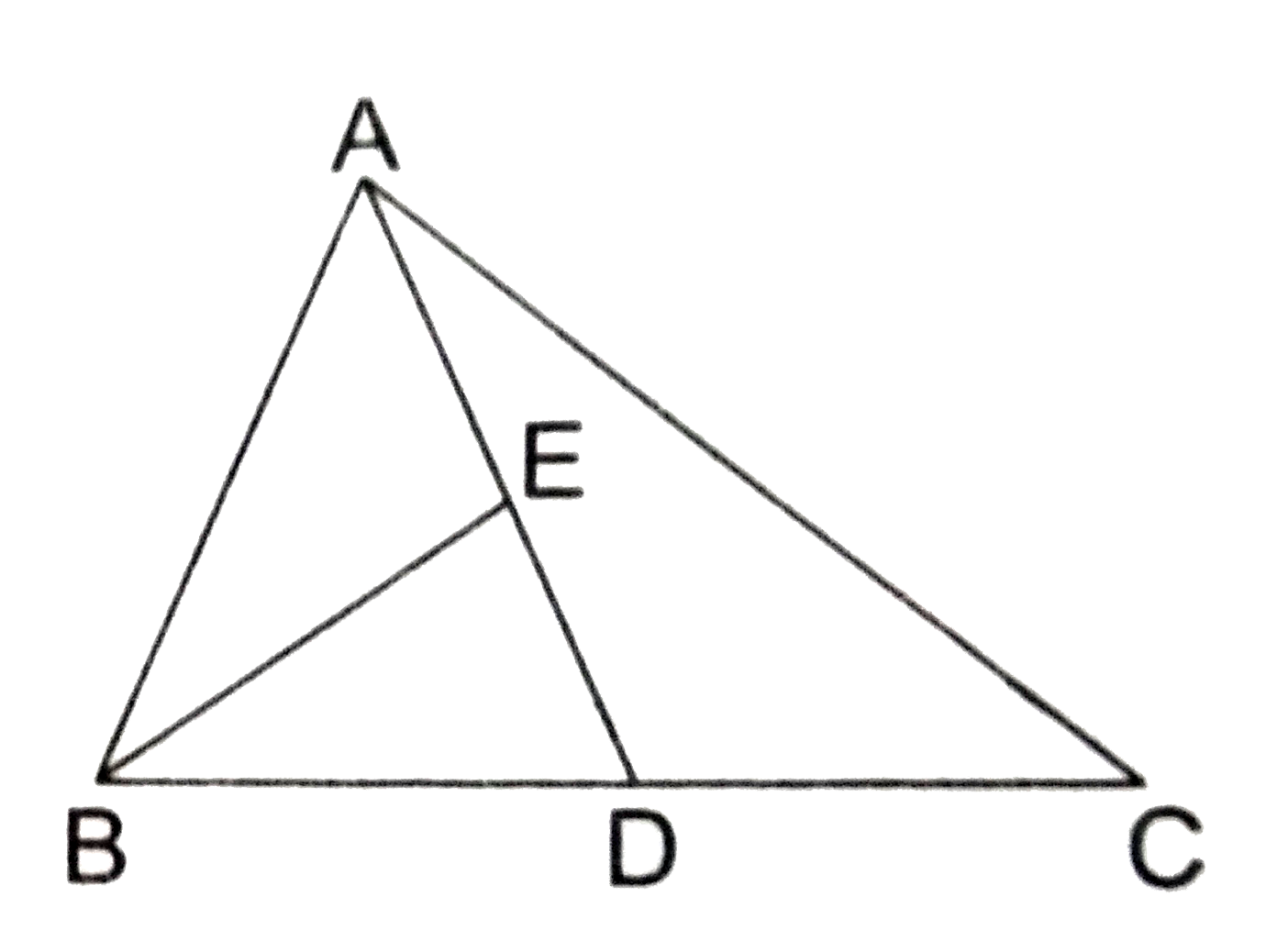
View SolutionABCD is a square. F is the midpoint of AB and BE is one fourth of BC. If the area of DFBE is 36 sq. cm. Find the length of AC.
View SolutionABC is a triangle in which D is the midpoint of BC and E is the midpoint of AD. Which of the following statements is/are correct?
1. The area of triangle ABC is equal to four times the area of triangle BED.
2. The area of triangle ADC is twice the area of triangle BED.
Select the correct answer using the code given below.
View SolutionABCD is a square of area 1 m2. P and Q are the midpoints of AB and BC respectively. What is the area of Δ DPQ ?
View SolutionArea of ΔABC=30cm2. D and E are the mid - points of BC and AB respectively. Find A(ΔBDE) :
View Solution
