1. Let PQR be a right angled isosceles triangle, right angled at P (2,1). If the equation of the line QR is 2x + y 3, then the equation representing the pair of (1999, 2M) lines P and PR is

$3 x^{2} - 3 y^{2} + 8 x y + 20 x + 10 y + 25 = 0$

$3 x^{2} - 3 y^{2} + 8 x y - 20 x - 10 y + 25 = 0$

$3 x^{2} - 3 y^{2} + 8 x y + 10 x + 15 y + 20 = 0$

$3 x^{2} - 3 y^{2} - 8 x y - 10 x - 15 y - 20 = 0$

Video Solution

Text Solution

Verified by Experts

Let $S$ be the mid-point of $Q R$ and given $Δ P Q R$ is an isosceles.
Therefore, $P S ⊥ Q R$ and $S$ is mid-point of hypotenuse, therefore $S$ is equidistant from $P$ , $Q$ , $R$ .
$∴ P S = Q S = R S$
Since, $∠ P = 90^{\circ}$ and $∠ Q = ∠ R$
But $∠ P + ∠ Q + ∠ R = 180^{\circ}$
$∴ 90^{\circ} + ∠ Q + ∠ R = 180^{\circ}$
$\Rightarrow ∠ Q = ∠ R = 45^{\circ}$

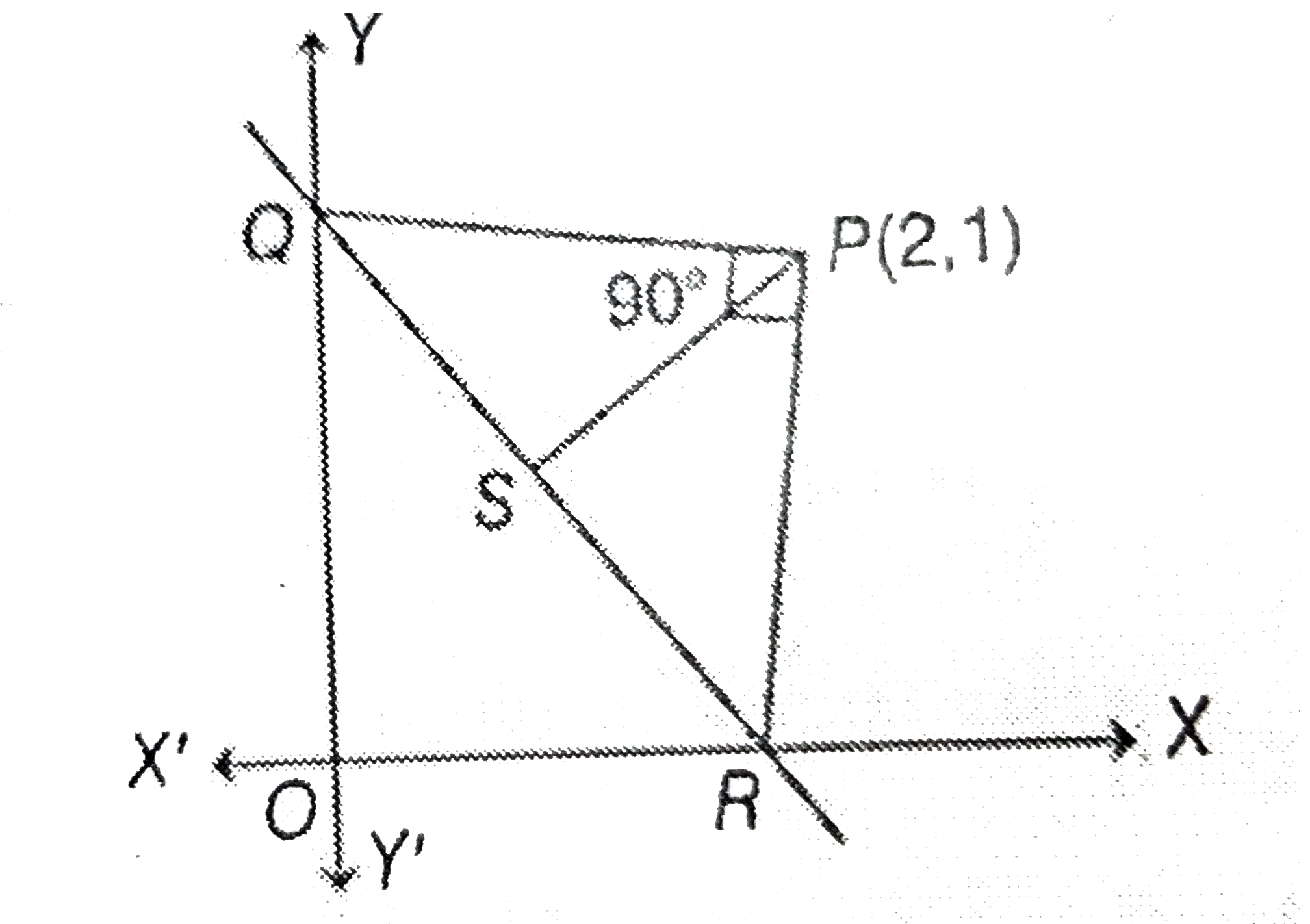
Now, slope of $Q R$ is $- 2$ [given]
But $Q R ⊥ P S$ .
$∴$ Slope of $P S$ is $1 / 2$ .
Let $m$ be the slope of $P Q$ .
$∴ tan (\pm 45^{\circ}) = \frac{m - 1 / 2}{1 - m (- 1 / 2)}$
$\Rightarrow \pm 1 = \frac{2 m - 1}{2 + m}$
$\Rightarrow m = 3, - 1 / 3$
$∴$ Equations of $P Q$ and $P R$ are
$y - 1 = 3 (x - 2)$
and $y - 1 = - \frac{1}{3} (x - 2)$
or $3 (y - 1) + (x - 2) = 0$
Therefore, joint equation of $P Q$ and $P R$ is
$[3 (x - 2) - (y - 1)] [(x - 2) + 3 (y - 1)] = 0$
$\Rightarrow 3 {(x - 2)}^{2} - 3 {(y - 1)}^{2} + 8 (x - 2) (y - 1) = 0$
$\Rightarrow 3 x^{2} - 3 y^{2} + 8 x y - 20 x - 10 y + 25 = 0$

Updated on:21/07/2023