Area of the parallelogram formed by the lines y = mx, y = mx + 1,y = nx and y =nx+1 equals to

A

$\frac{| m + n |}{{(m - n)}^{2}}$

B

$\frac{2}{| m + n |}$

C

$\frac{1}{| m + n |}$

D

$\frac{1}{| m - n |}$

Video Solution

Text Solution

Verified by Experts

Let lines $O B : y = m x$
$C A : y = m x + 1$
$B A : y = n x + 1$
and $O C : y = n x$
The point of intersection $B$ of $O B$ and $A B$ has $x$ coordinate $\frac{1}{m - n}$ .

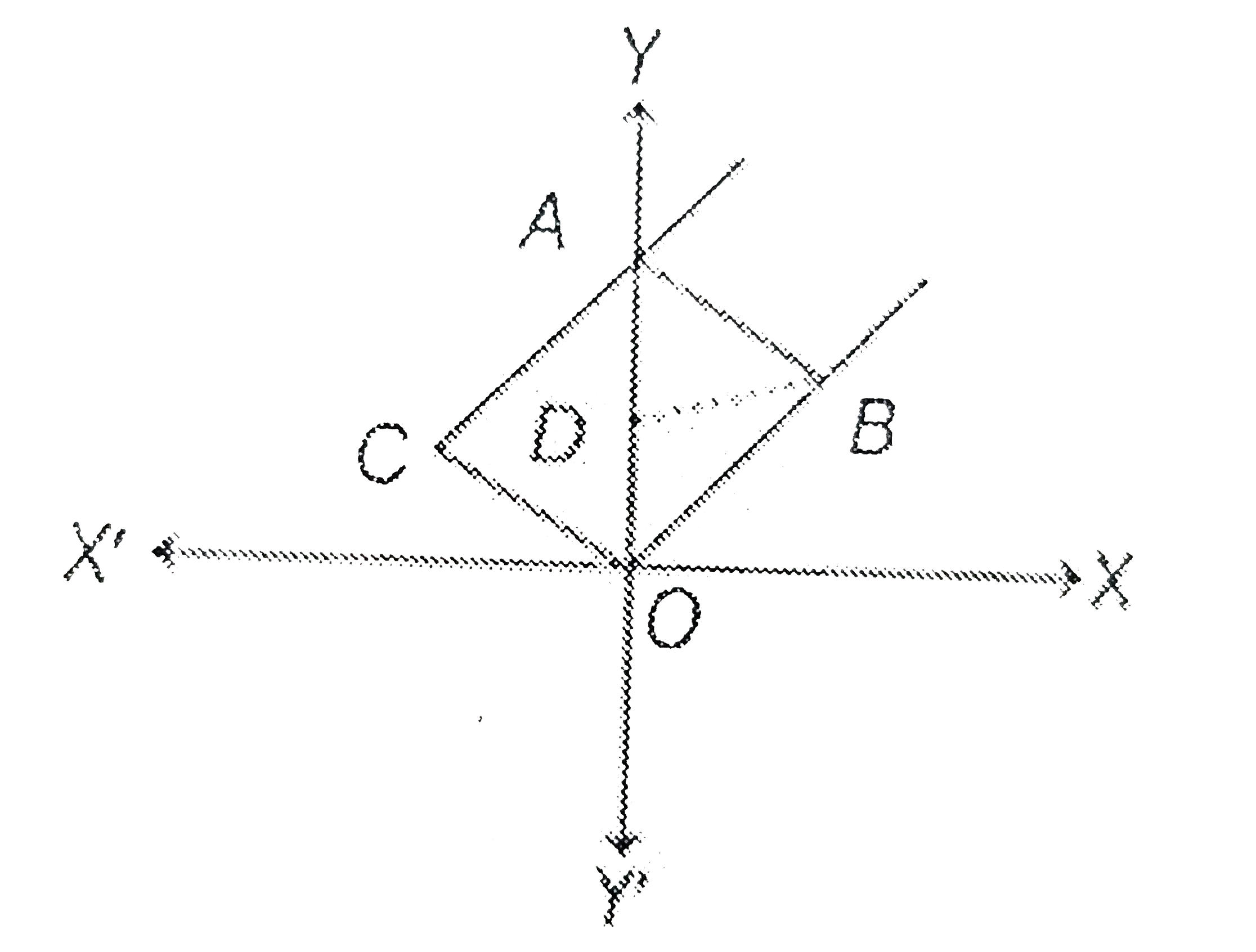
Now, area of a parallelogram $O B A C$
$= 2 \times area Of Δ O B A$
$= 2 \times \frac{1}{2} \times O A \times O B = 2 \times \frac{1}{2} \times \frac{1}{m - n}$
$= \frac{1}{m - n} = \frac{1}{| m - n |}$
depending upon whether $m > n$ or $m < n$ .

Updated on:21/07/2023

|

Class 12 MATHS STRAIGHT LINE AND PAIR OF STRAIGHT LINES

Topper's Solved these Questions

Similar Questions

Knowledge Check

The area of the region bounded by the lines y = mx, x = 1, x = 2 and X-axis is 6 sq units, then m is equal to
A3
B1
C2
D4
The lines y = mx , $y + 2 x = 0, y = 2 x + k and y + m x = k$ form a rhombus if m equals
A $- 1$
B $\frac{1}{2}$
C1
D2
Area of triangle formed by straight line $y = m x + c$ with coordinate area
A $\frac{c^{2}}{m}$
B $\frac{m}{c^{2}}$
C $\frac{c^{2}}{2 m}$
D $\frac{2 m}{c^{2}}$

IIT JEE PREVIOUS YEAR-STRAIGHT LINE AND PAIR OF STRAIGHT LINES-AREA AND FAMILY OF CONCURRENT LINES (Objective Questions I) (Only one correct option)

Home
Ask Doubt
Profile