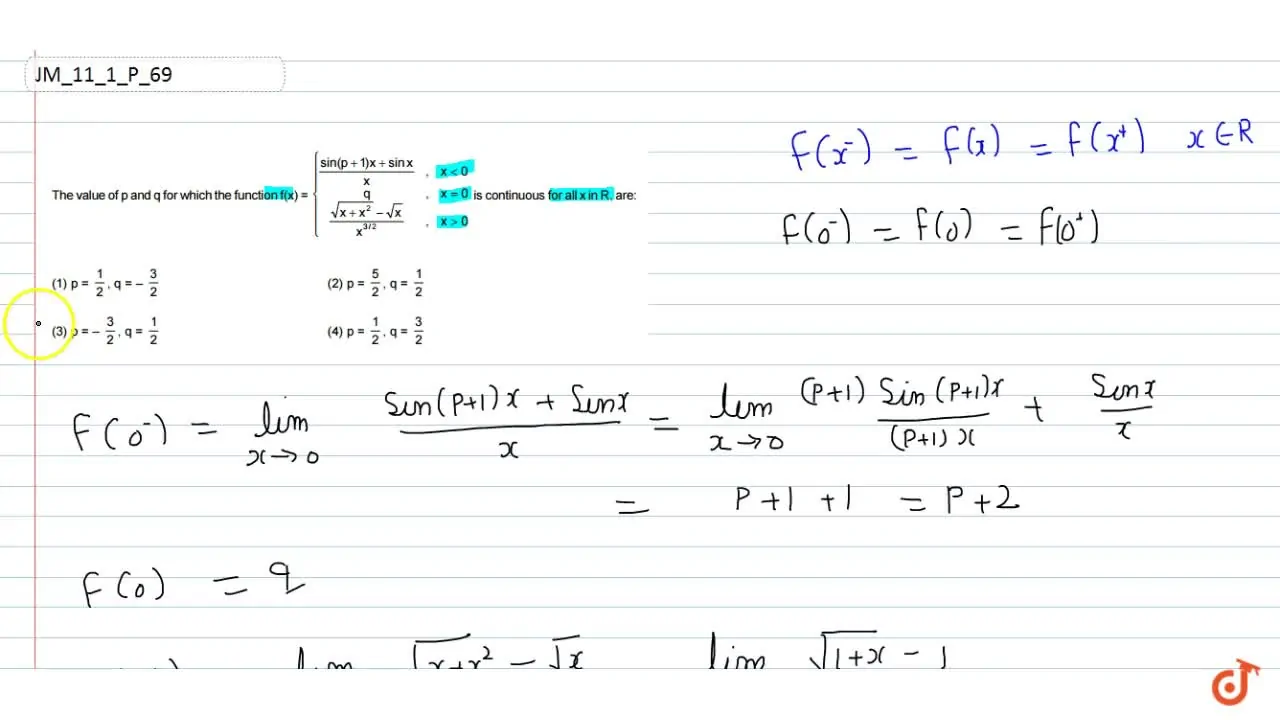
The value of p and q for which the function f(x)={sin(p+1)x+sinxx,x<0q,x=0√x+x2−√xx3/2,x>0} is continuous for all x in R, are: (1) p=12,q=−32 (2) p=52,q=−12 (2) p=−32,q=12 (4) p=12,q=32

A

(−32,−12)

B

(−12,32)

C

(52,12)

D

(−32,12)

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:D

Given function
f(x)=⎧⎪ ⎪ ⎪ ⎪⎨⎪ ⎪ ⎪ ⎪⎩sin(p+1)x+sinxx , x<0 , x=0√x+x2−√xx3/2 , x>0
is continuous at x = '0, then
f(0)=limx→0−f(x)=limx→0+f(x)....(i)
limx→0−f(x)=limx→0−sin(p+1)x+sinxx
=p+1+1+=p+2[∵limx→0sin(ax)x=a]andlimx→0+f(x)=limx→0+√x+x2−√xx3/2
=limx→0+√x[(1+x)1/2−1]x√x
=limx→0+(1+12x+12(12−1)2!x2+...−1)x
[∵(1+x)n
=1+nx+n(n−1)1⋅2x2+n(n−1(n−2))1⋅2⋅3x3+...,|x|<1]
=limx→0+⎛⎜ ⎜⎝12+12(12−1)2!x+...⎞⎟ ⎟⎠=12
From Eq. (i), we get
f(0)=q=12andlimx→0−f(x)=p+2=12
⇒ p=−32
So, (p,q)=(−32,12)

Updated on:21/07/2023
|
Class 12MATHSLIMITS AND DERIVATIVES

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • The values of p and q for which the function
    f(x)=⎧⎪ ⎪ ⎪ ⎪⎨⎪ ⎪ ⎪ ⎪⎩sin(p+1)x+sinxxx<0qx=0√x+x2−√xx3/2x>0 is continuous for all x in R are

    Ap=5/2 and q=1/2
    Bp=-3/2 and q=1/2
    Cp=1/2 and q=3/2
    Dp=1/2 and q=-3/2

  • The value of p and q for which the function
    f(x)=⎧⎪ ⎪ ⎪ ⎪⎨⎪ ⎪ ⎪ ⎪⎩sin(p+1)x+sinxx,x<0q,x=0√x+x2−√xx1/2,x>0
    is continuous for all x in R, are

    Ap=52,q=12
    Bp=−32,q=12
    Cp=12,q=32
    Dp=12,q=−32

  • The value of p and q for which the function
    f(x)=⎧⎪ ⎪ ⎪ ⎪⎨⎪ ⎪ ⎪ ⎪⎩sin(p+1)x+sinxx,x<0q,x=0√x+x2−√xx3/2,x>0
    is continuous for all x in R, are

    Ap=12,q=−32
    Bp=52,q=12
    Cp=−32,q=12
    Dp=12,q=32