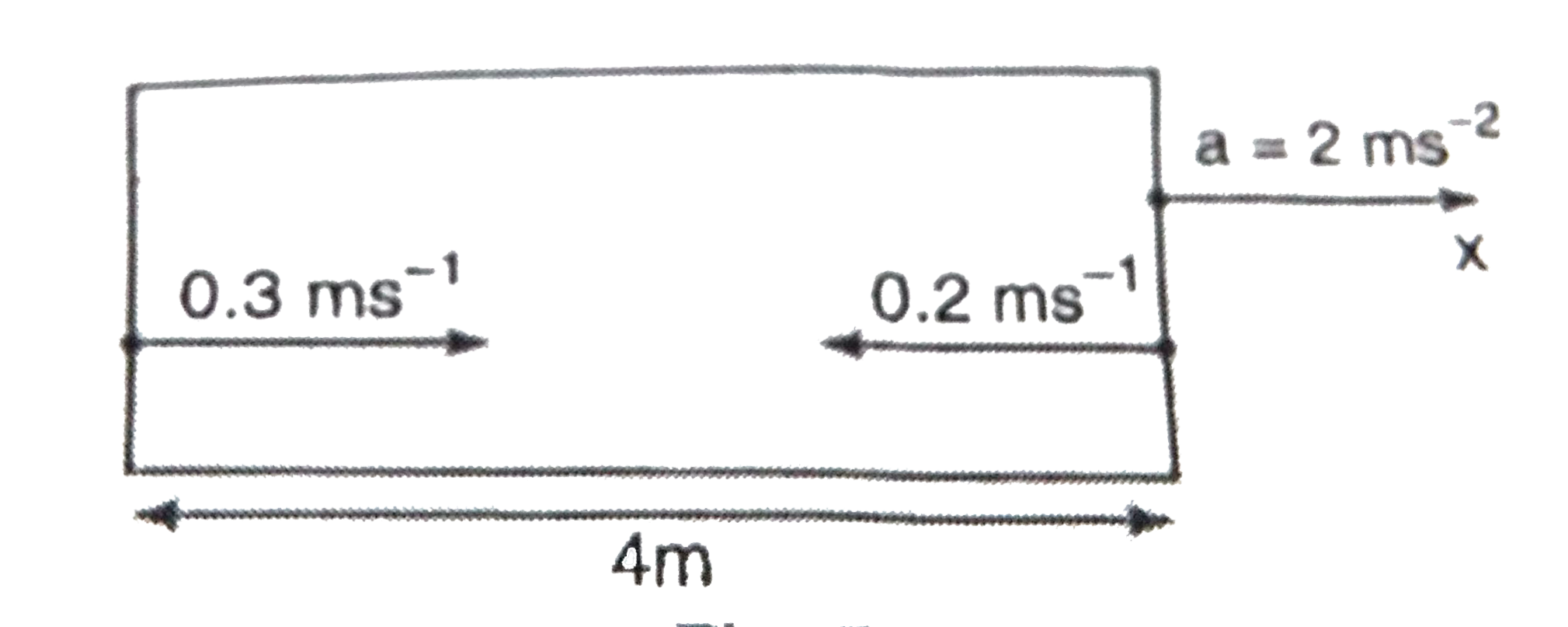
A rocket is moving in a gravity free space with a constnat acceleration of 2ms−1 along +x direction (see Fig.5.126). The length of a chamber inside the rocket is 4m. A ball is thrown from th left end of the chamber in +x direction with a speed of 0.3 ms−1 relaitve to the rocket. At the same time , another ball is thrown in -x direction with a speed of 0.2ms^(-1) from its right and relative to the rocket. the time in seconds when the two balls hit each other is:
The correct Answer is:2
Step by step video, text & image solution for A rocket is moving in a gravity free space with a constnat acceleration of 2ms^(-1) along +x direction (see Fig.5.126). The length of a chamber inside the rocket is 4m. A ball is thrown from th left end of the chamber in +x direction with a speed of 0.3 ms^(-1) relaitve to the rocket. At the same time , another ball is thrown in -x direction with a speed of 0.2ms^(-1) from its right and relative to the rocket. the time in seconds when the two balls hit each other is: by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - CHAPTER PRACTICE TEST
16 Videos
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - COMPETITION FILE (MATRIX MATCH TYPE QUESTIONS )
1 Videos
- MOTION IN A PLANE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - Chapter Practice Test
15 Videos
- OSCILLATIONS BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Practice Test (For Board Examination)
12 Videos
Similar Questions
A rocket is moving in a gravity free space with a constant acceleration of 2ms−1 along +x direction (see Fig.5.126). The length of a chamber inside the rocket is 4m. A ball is thrown from the left end of the chamber in +x direction with a speed of 0.3 ms−1 relative to the rocket. At the same time , another ball is thrown in -x direction with a speed of 0.2ms−1 from its right and relative to the rocket. the time in seconds when the two balls hit each other is:

View SolutionA ball is dropped from a top of a 100 m high building. At the ssame time another ball is thrown up from the ground with a speed of 20ms−1. At what time will the balls meet?
View Solution
Knowledge Check
A ball is dropped from top of a tower 250 m high. At the same time, another ball is thrown upwards with a velocity of 50ms−1 from the ground. The time at which they cross each other is
A3 secondB5 secondC10 secondD12 secondA particle (A) is dropped from a height and another particles (B) is thrown into horizontal direction with speed of 5m/s sec from the same height. The correct statement is
ABoth partiles will reaches at ground simultaneouslyBBoth particles will reaches at ground with the same speedCParticle (A) will reach at ground at first with respect to particle (B)DParticle (B) will reach at ground at first with respect to particle (A)A ball is thrown in upwards direction then the force(s) acting on it is (are)
Agravitational force.Bmechanical force.Cfictional force.DBoth (a) and (c).
A ball is dropped from the top of a tower. After 2 s another ball is thrown vertically downwards with a speed of 40ms−1. After how much time and at what distance below the top of tower the balls meet ?
View SolutionA cricket ball is thrown at a speed of 28ms−1 in a direction 30∘ above the horizontal. Calculate the time taken by the ball to return to the same level.
View SolutionA ball is thrown downwards with a speed of 20ms–1 from top of a building 150m high and simultaneously another ball is thrown vertically upwards with a speed of 30ms–1 from the foot of the building. Find the time after which both the balls will meet - (g=10ms–2)
View SolutionA ball is thrown horizontally from the top a tower with a speed of 50ms−1 . Find the velocity and position at the end of 3 second. g=9.8ms−2.
View SolutionA 1 kg ball moving with a speed of 6 ms−1 collides head - on with a 0.5 kg ball moving in the opposite direction with a speed of 9 ms−1. If the coefficient of restitution is 13, the energy lost in the collision is
View Solution
