The velocity-time graph of a particle in one-dimensional motion is shown in Fig 3.29:

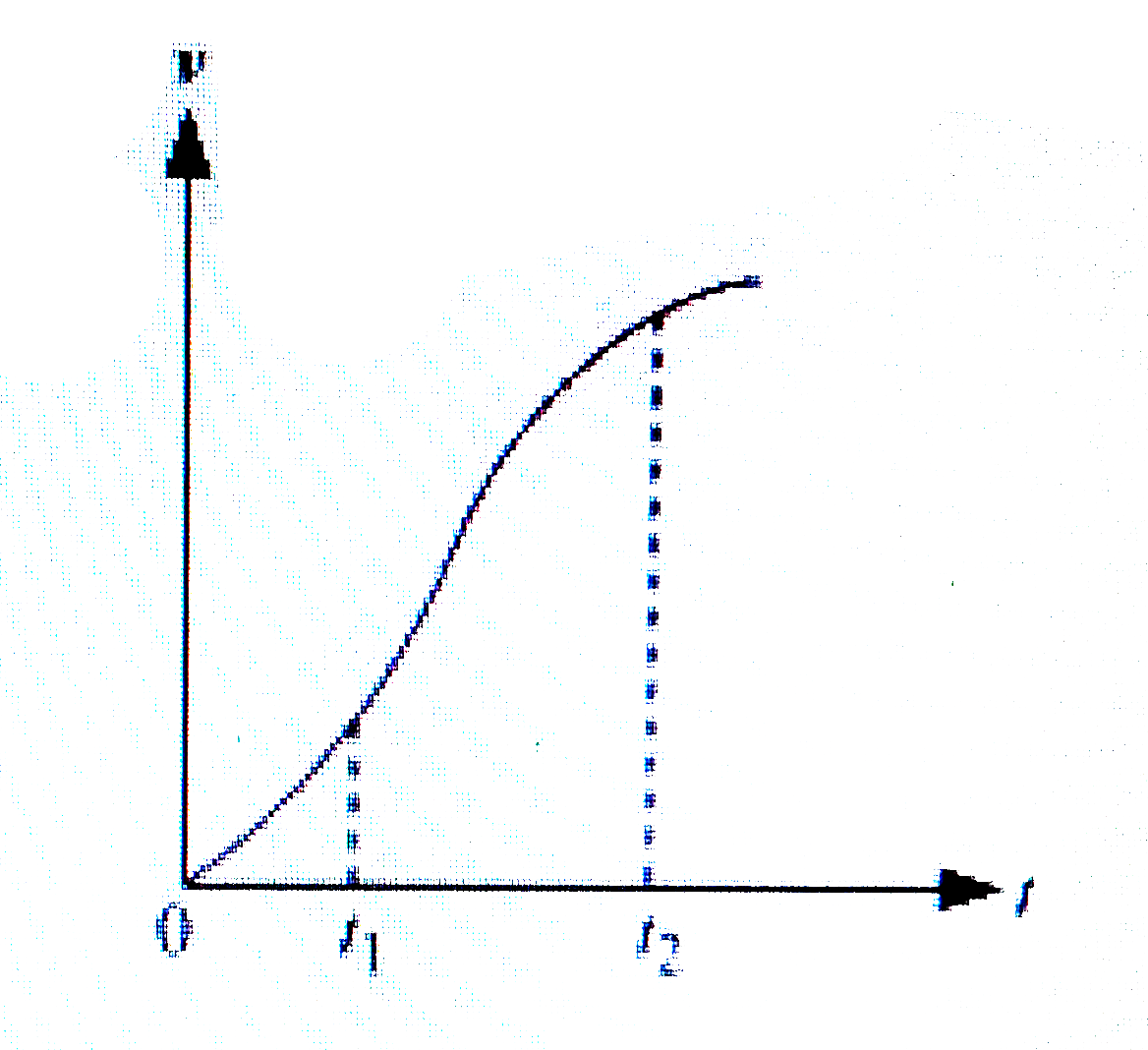
(a) Which of the following formylae are correct for describing the motion of the particle over the time -interval $t_{1}$ to $t_{2}$ :
(a) $x (t_{2}) = x (t_{1}) + v (t_{1}) (t_{2} - t_{1}) + (1 / 2) a {(t_{2} - t_{1})}_{2}^{2}$
(b) $v (t_{2}) = v (t_{1}) + a (t_{2} - t_{1})$
(c) $v_{average} = (x (t_{2}) - x (t_{1})) / (t_{2} - t_{1})$
(d) $a_{average} = (v (t_{2}) - v (t_{1})) / (t_{2} - t_{1})$
(e) $x (t_{2}) = x (t_{1}) + v_{average} (t_{2} - t_{1}) + (1 / 2) a_{average} {(t_{2} - t_{1})}_{2}^{2}$
(f) $x (t_{2}) - x (t_{1})$ = area under the $v - t$ curve bounded by the t-axis and the dotted line shown.

Video Solution

Text Solution

Verified by Experts

As the motion of the particle over the interval $t_{1}$ and $t_{2}$ is non-unifom. Therefore the relation c,d,f are correct but a,b,e represent uniform acceleration.

|

Updated on:21/07/2023

Class 11 PHYSICS RECTILINEAR MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The velocity-time graph of a particle in one-dimensional motion is shown in the figure. Which of the following formulae is correct for describing the motion of the particle over the time interval $t_{1}$ to $t_{2}$ ?

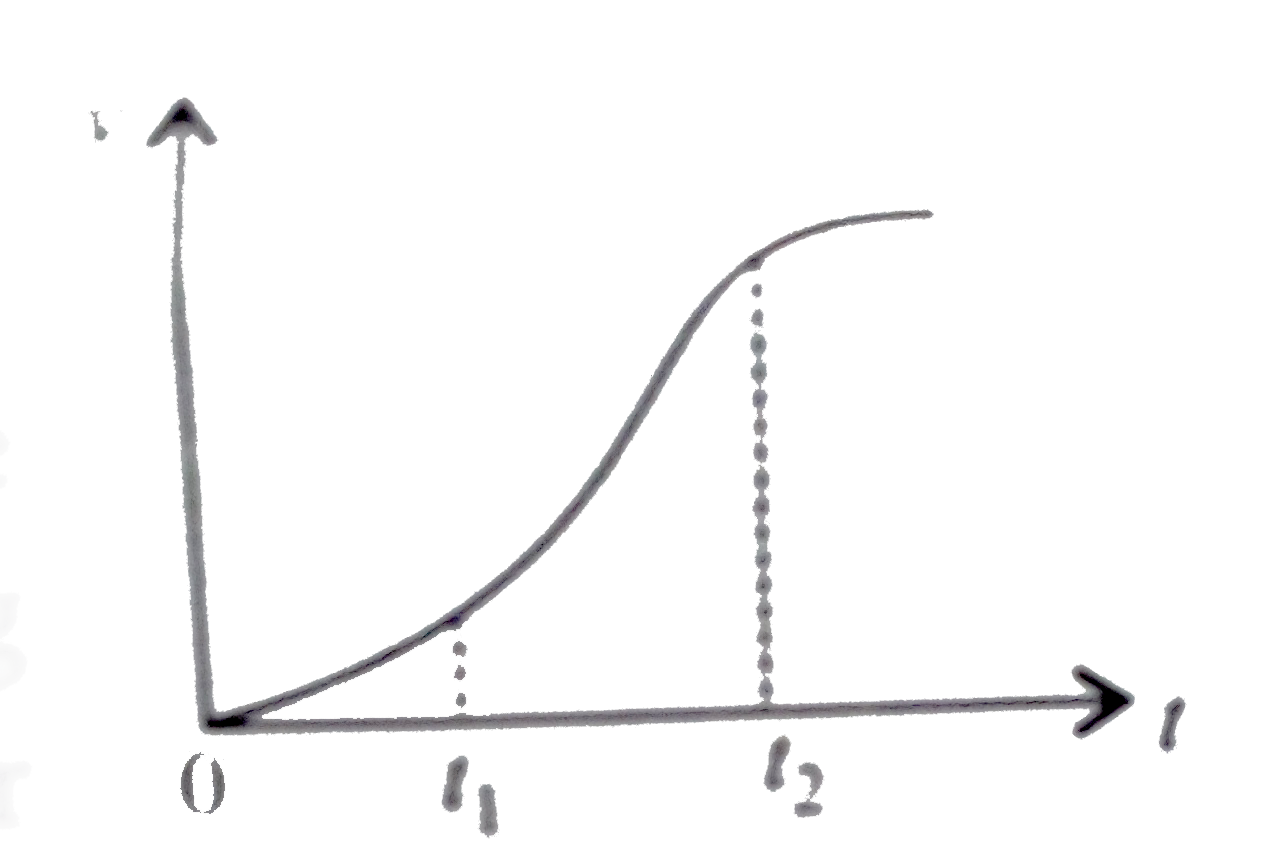A $x (t_{2}) = x (t_{1}) + v (t_{1}) (t_{2} - t_{1}) + (\frac{1}{2}) a {(t_{2} - t_{1})}_{2}^{2}$
B $v (t_{2}) = v (t_{1}) + a (t_{2} - t_{1})$
C $v_{average} = \frac{x (t_{2}) + x (t_{1})}{t_{2} - t_{1}}$
D $a_{average} = \frac{v (t_{2}) - v (t_{1})}{t_{2} - t_{1}}$
Question 2 - Select One
If $(1^{2} - t_{1}) + (2^{2} - t_{2}) + + (n^{2} - t_{n}) + = \frac{n (n^{2} - 1)}{3}$ , then $t_{n}$ is equal to $n^{2}$ b. $2 n$ c. $n^{2} - 2 n$ d. none of these
A $n / 2$
Bn - 1
Cn + 1
Dn
Question 3 - Select One
In any progression, if $\frac{t_{2} t_{3}}{t_{1} t_{4}} = \frac{t_{2} + t_{3}}{t_{1} + t_{4}} = 3 (\frac{t_{2} - t_{3}}{t_{1} - t_{4}})$ , then $t_{1}, t_{2}, t_{3}, t_{4}$ are in
AA.P.
BG.P.
CH.P.
Dnone

MODERN PUBLICATION-MOTION IN A STRAIGHT LINE -NCERT (ADDITIONAL EXERCISE)

Home
Ask Doubt
Profile