A circular hoop rolls without slipping on a flat horizontal surface. Which one of the following is necessarily true?
All points on the rim of the hoop have the same speed.
All points on the rim of the hoop have the same velocity
Every point on the rim of the wheel has a different velocity
All of these
The correct Answer is:C
To solve the problem, we need to analyze the motion of a circular hoop that rolls without slipping on a flat horizontal surface.
1. Understanding Rolling Without Slipping:
- When a circular hoop rolls without slipping, it means that the point of contact with the ground does not slide. This condition implies a specific relationship between the translational and rotational motion of the hoop.
2. Defining Motion Parameters:
- Let the radius of the hoop be r.
- Let the translational speed of the center of the hoop be v.
- Let the angular velocity of the hoop be ω.
3. Condition for Pure Rolling:
- The condition for rolling without slipping can be expressed as:
v=rω
- This means that the linear speed of the center of the hoop is equal to the tangential speed at the rim due to rotation.
4. Analyzing Points on the Hoop:
- Consider different points on the rim of the hoop:
- Point C (the point in contact with the ground):
- The tangential speed at this point due to rotation is rω (downward), and the translational speed is v (forward). Since v=rω, the net speed at point C is zero. Hence, point C is momentarily at rest.
- Point A (the topmost point):
- The tangential speed is rω (upward), and the translational speed is v (forward). The net speed at point A is v+rω=2v.
- Point B (any point above the center):
- The net speed will be a combination of the translational and rotational speeds, leading to different magnitudes and directions.
5. Conclusion on Speeds and Velocities:
- Since point C has a speed of 0, point A has a speed of 2v, and other points have speeds that vary between these two extremes, it is clear that:
- All points on the rim do not have the same speed.
- All points on the rim do not have the same velocity (as velocity includes direction).
- Every point on the rim has a different velocity due to the different magnitudes and directions of motion.
6. Final Answer:
- Therefore, the correct statement is that every point on the rim of the wheel has a different velocity. The correct answer is option 3.
- When a circular hoop rolls without slipping, it means that the point of contact with the ground does not slide. This condition implies a specific relationship between the translational and rotational motion of the hoop.
- Let the radius of the hoop be r.
- Let the translational speed of the center of the hoop be v.
- Let the angular velocity of the hoop be ω.
- The condition for rolling without slipping can be expressed as:
v=rω
- This means that the linear speed of the center of the hoop is equal to the tangential speed at the rim due to rotation.
- Consider different points on the rim of the hoop:
- Point C (the point in contact with the ground):
- The tangential speed at this point due to rotation is rω (downward), and the translational speed is v (forward). Since v=rω, the net speed at point C is zero. Hence, point C is momentarily at rest.
- Point A (the topmost point):
- The tangential speed is rω (upward), and the translational speed is v (forward). The net speed at point A is v+rω=2v.
- Point B (any point above the center):
- The net speed will be a combination of the translational and rotational speeds, leading to different magnitudes and directions.
- Since point C has a speed of 0, point A has a speed of 2v, and other points have speeds that vary between these two extremes, it is clear that:
- All points on the rim do not have the same speed.
- All points on the rim do not have the same velocity (as velocity includes direction).
- Every point on the rim has a different velocity due to the different magnitudes and directions of motion.
- Therefore, the correct statement is that every point on the rim of the wheel has a different velocity. The correct answer is option 3.
Topper's Solved these Questions
- RIGID BODY DYNAMICS-IIBOOK - RESNICK AND HALLIDAYCHAPTER - RIGID BODY DYNAMICS-IIEXERCISE - PRACTICE QUESTIONS (More than One Option Correct Type)
2 Videos
- RIGID BODY DYNAMICS-IIBOOK - RESNICK AND HALLIDAYCHAPTER - RIGID BODY DYNAMICS-IIEXERCISE - PRACTICE QUESTIONS(Linked Comprehension)
3 Videos
- RIGID BODY DYNAMICS-IIBOOK - RESNICK AND HALLIDAYCHAPTER - RIGID BODY DYNAMICS-IIEXERCISE - PROBLEMS
41 Videos
- RIGID BODY DYNAMICS - IBOOK - RESNICK AND HALLIDAYCHAPTER - RIGID BODY DYNAMICS - IEXERCISE - PRACTICE QUESTIONS (INTEGER TYPE))
3 Videos
- TEMPERATURE, ZEROTH LAW OF THERMODYNAMICS AND THERMAL EXPANSION BOOK - RESNICK AND HALLIDAYCHAPTER - TEMPERATURE, ZEROTH LAW OF THERMODYNAMICS AND THERMAL EXPANSION EXERCISE - PRACTICE QUETIONS (Integer Type)
4 Videos
Similar Questions
A circular disc of radius r is rolling without slipping on a horizontal surface. What is the ratio of the translational KE and rotational KE of disc ?
View SolutionA circular disc of radius R rolls without slipping along the horizontal surface with constant velocity v0. We consider a point A on the surface of the disc. Then the acceleration of the point A is:
View Solution
Knowledge Check
A rigid ball rolls without slipping on a surface shown below.

Which one of the following is the most likely representation of the distance traveled by the ball vs time graph?A B
B C
C D
D
If |b|≥1 and x=−|a|b, then which one of the following is necessarily true ?
Aa−xb<0Ba−xb≥0Ca−xb>0Da−xb≤0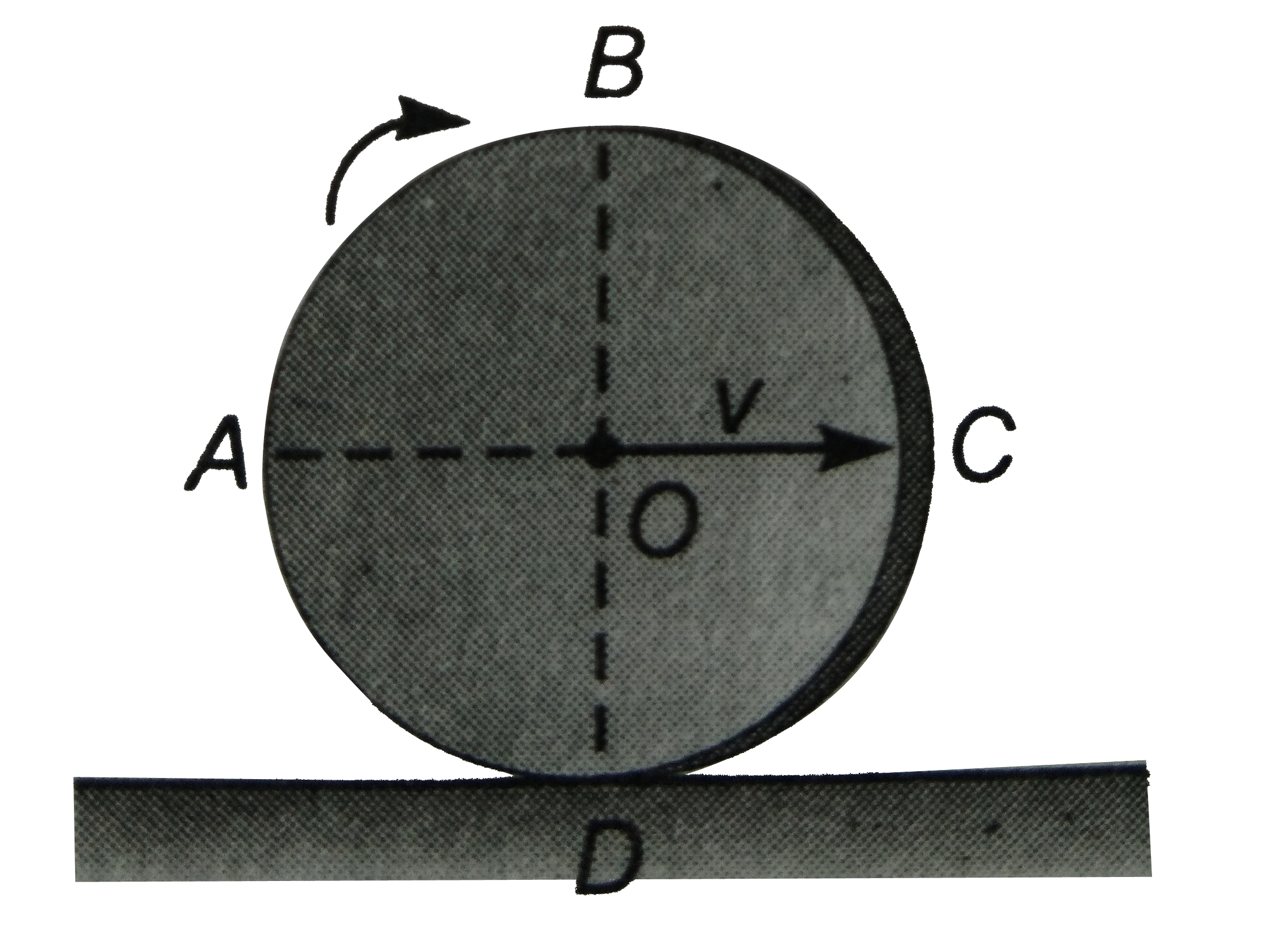
A uniform circular righ rolls without slipping on a horizontal surface. At any instant, its position is as shown in the figure. ThenAsection ABC has greater kinetic energy than section ADCBsection BC has greater kinetic energy than section CD.Csection BC has the same kinetic energy as section DADthe section CD and DA have the same kinetic energy.
A circular disc of radius R rolls without slipping along the horizontal surface with constant velocity v0. We consider a point A on the surface of the disc. Then the acceleration of the point A is:
View SolutionA solid cylinder of mass M and radius R rolls without slipping on a flat horizontal surface. Its moment of inertia about the line of contact is ?
View SolutionIf the roots of a quadratic equation are real and unequal, which one of the following is necessarily true?
View SolutionA solid sphere rolls without slipping along a horizontal surface. What percentage of its total kinetic energy is rotational kinetic energy?
View SolutionWhen a body is rolling without slipping on a rough horizontal surface, the work done by friction is :
View Solution
