Three boxes of capacity 24 kg. 36 kg and 84 kg are completely filled with three varieties of wheat A, B and C respectively. All the three boxes were emptied and the three types of wheat were thoroughly mixed and the mixture was put back in the three boxes. How many kg of type A wheat would be there in the third box (in kg)?
10
12
14
16
The correct Answer is:3
To solve the problem step by step, we will follow these instructions:
Step 1: Identify the capacities of the boxes and the types of wheat.
- The capacities of the boxes are:
- Box 1: 24 kg (filled with wheat A)
- Box 2: 36 kg (filled with wheat B)
- Box 3: 84 kg (filled with wheat C)
Step 2: Calculate the total capacity of the boxes.
- Total capacity = Capacity of Box 1 + Capacity of Box 2 + Capacity of Box 3
- Total capacity = 24 kg + 36 kg + 84 kg = 144 kg
Step 3: Determine the ratio of the capacities of the boxes.
- The ratio of the capacities of the boxes is:
- Box 1 : Box 2 : Box 3 = 24 : 36 : 84
- To simplify this ratio, we can divide each term by 12:
- 24 ÷ 12 = 2
- 36 ÷ 12 = 3
- 84 ÷ 12 = 7
- Thus, the simplified ratio is 2 : 3 : 7.
Step 4: Calculate the total parts in the ratio.
- Total parts = 2 + 3 + 7 = 12 parts.
Step 5: Determine the amount of type A wheat in the total mixture.
- The amount of type A wheat in the total mixture is based on the ratio:
- Since type A corresponds to 2 parts out of a total of 12 parts, we can calculate the amount of type A wheat:
- Amount of type A wheat = (2/12) * Total capacity
- Amount of type A wheat = (2/12) * 144 kg = 24 kg.
Step 6: Distribute the type A wheat back into the boxes based on their capacities.
- We need to find out how much type A wheat goes into Box 3 (which has a capacity of 84 kg).
- The ratio of the boxes is 2 : 3 : 7, which means:
- Box 1 (2 parts) will get (2/12) of the total type A wheat,
- Box 2 (3 parts) will get (3/12) of the total type A wheat,
- Box 3 (7 parts) will get (7/12) of the total type A wheat.
Step 7: Calculate the amount of type A wheat in Box 3.
- Amount of type A wheat in Box 3 = (7/12) * Total amount of type A wheat
- Amount of type A wheat in Box 3 = (7/12) * 24 kg = 14 kg.
Final Answer:
- Therefore, the amount of type A wheat in the third box is 14 kg.
---
- The capacities of the boxes are:
- Box 1: 24 kg (filled with wheat A)
- Box 2: 36 kg (filled with wheat B)
- Box 3: 84 kg (filled with wheat C)
- Total capacity = Capacity of Box 1 + Capacity of Box 2 + Capacity of Box 3
- Total capacity = 24 kg + 36 kg + 84 kg = 144 kg
- The ratio of the capacities of the boxes is:
- Box 1 : Box 2 : Box 3 = 24 : 36 : 84
- To simplify this ratio, we can divide each term by 12:
- 24 ÷ 12 = 2
- 36 ÷ 12 = 3
- 84 ÷ 12 = 7
- Thus, the simplified ratio is 2 : 3 : 7.
- Total parts = 2 + 3 + 7 = 12 parts.
- The amount of type A wheat in the total mixture is based on the ratio:
- Since type A corresponds to 2 parts out of a total of 12 parts, we can calculate the amount of type A wheat:
- Amount of type A wheat = (2/12) * Total capacity
- Amount of type A wheat = (2/12) * 144 kg = 24 kg.
- We need to find out how much type A wheat goes into Box 3 (which has a capacity of 84 kg).
- The ratio of the boxes is 2 : 3 : 7, which means:
- Box 1 (2 parts) will get (2/12) of the total type A wheat,
- Box 2 (3 parts) will get (3/12) of the total type A wheat,
- Box 3 (7 parts) will get (7/12) of the total type A wheat.
- Amount of type A wheat in Box 3 = (7/12) * Total amount of type A wheat
- Amount of type A wheat in Box 3 = (7/12) * 24 kg = 14 kg.
- Therefore, the amount of type A wheat in the third box is 14 kg.
Similar Questions
Three particles of msses 1.0 kg 2.0 kg and 3.0 kg are placed at the corners A,B and C respectively of an equilateral triangle ABC of edge 1m. Locate the centre ofmass of the system.
View SolutionThree particles of masses 1kg, 2kg and 3kg are placed at the corners A, B and C respectively of an equilateral triangle ABC of edge 1m. Find the distance of their centre of mass from A.
View Solution
Knowledge Check
Find the proportion in which three types of sugar at 12, 14 and 20 may be mixed so as to obtain a mixture worth 15 per kg?
A15:5:6B5:15:6C3:12:16D4:12:15Three taps A, B and C can fill a tank in 27, 36 and 54 minutes respectively. If all the three taps are opened, then how much time (in minutes) it will take to completely fill the tank?
A16B12C10D15A 100 kg block is suspended with the help of three strings A, B and C. The tension in the string A is
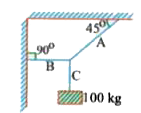 A50 g NB100 g NC20 g ND100√2gN
A50 g NB100 g NC20 g ND100√2gN
Three particles of msses 1.0 kg , , 2.0 kg and 3.0 kg are placed at the corners A,B and C respectively of an equilateral triangle ABC of edge 1m. Locate the centre of mass of the system.
View SolutionThe weights of three boxes are 3 kg, 8 kg and 12 kg. Which of the following CANNOT be the total weight, in kg, of any combination of these boxes?
View SolutionWheat costing Rs 30/kg, Rs 35/kg and a third variety of wheat are mixed in the ratio of 3 : 4 : 2. If the mixture is costs Rs 34/kg, then what will be the cost (in Rs/kg) of the third variety of wheat?
View SolutionVenu mixed two varieties of wheat costing Rs 20 and Rs 25 per kg in the ratio 5:4 and sold the mixture at 24 per Kg Find the profit or loss in the whole transaction.
View SolutionVeenu purchased two types of wheat, one costing Rs. 20 per kg and the other Rs. 25 per kg in quantities having ratio 5:4 He mixed the two and sold the mixture at Rs. 24 per kg. In the transaction he got
View Solution
