A man rows down a river 15 km in 3 hrs. with the stream and returns in 712 hrs, The rate at which he rows in still water is
2.5 km/hr
1.5 km/hr
3.5 km/hr
4.5 km/hr
The correct Answer is:C
To solve the problem of finding the rate at which a man rows in still water, we can break down the solution into clear steps.
1. Define Variables:
Let:
- x = speed of the boat in still water (in km/hr)
- y = speed of the stream (in km/hr)
2. Determine Speeds:
- When rowing downstream (with the stream), the effective speed is x+y.
- When rowing upstream (against the stream), the effective speed is x−y.
3. Use Given Information:
- The man rows 15 km downstream in 3 hours. Therefore:
Speed downstream=DistanceTime=15 km3 hr=5 km/hr
This gives us the equation:
x+y=5(1)
- The man returns 15 km upstream in 712 hours. Convert 712 hours to an improper fraction:
712=152 hours
Thus, the speed upstream is:
Speed upstream=15 km152 hr=2 km/hr
This gives us the equation:
x−y=2(2)
4. Solve the System of Equations:
Now we have a system of two equations:
x+y=5(1)x−y=2(2)
To eliminate y, we can add both equations:
(x+y)+(x−y)=5+2
This simplifies to:
2x=7⟹x=72=3.5 km/hr
5. Find y:
Substitute x=3.5 back into equation (1):
3.5+y=5⟹y=5−3.5=1.5 km/hr
6. Conclusion:
The speed at which the man rows in still water is:
3.5 km/hr
Let:
- x = speed of the boat in still water (in km/hr)
- y = speed of the stream (in km/hr)
- When rowing downstream (with the stream), the effective speed is x+y.
- When rowing upstream (against the stream), the effective speed is x−y.
- The man rows 15 km downstream in 3 hours. Therefore:
Speed downstream=DistanceTime=15 km3 hr=5 km/hr
This gives us the equation:
x+y=5(1)
712=152 hours
Thus, the speed upstream is:
Speed upstream=15 km152 hr=2 km/hr
This gives us the equation:
x−y=2(2)
Now we have a system of two equations:
x+y=5(1)x−y=2(2)
To eliminate y, we can add both equations:
(x+y)+(x−y)=5+2
This simplifies to:
2x=7⟹x=72=3.5 km/hr
Substitute x=3.5 back into equation (1):
3.5+y=5⟹y=5−3.5=1.5 km/hr
The speed at which the man rows in still water is:
3.5 km/hr
Similar Questions
Direction: Study the data carefully and answer the following questions. Following is the production data by 4 persons during 2 months.
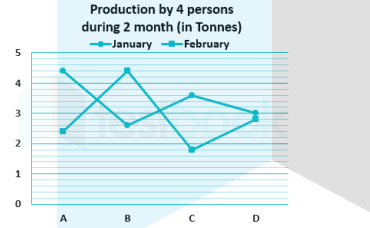
A man rows down a river 30 km in 6 hrs with the stream and returns in 10 hrs. The rate at which he rows in still water is
View SolutionA man rows down a river 18 km in 4 hours with the stream and returns in 10 hours. Consider the following statements :
1. The speed of the man against the stream is 1.8 km/hr.
2. The speed of the man in still water is 3.15 km/hr.
3. The speed of the stream is 1.35 km/hr.
Which of the above statements are correct ?
View Solution
Knowledge Check
A man rows 750 m in 675 seconds against the stream and returns in712 minutes .Find his rowing speed in still water .
A3 kmphB4 kmphC5 kmphD6kmphA man rows 750 m in 675 sec - onds against the stream and returns in712 minutes .Find his rowing speed in still water .
A5.5B5.75C5D5.25A man rows 750 m in 775 seconds against the stream and return in 712 minutes . What is rowing speed in still water ?
A4.7 km/hrB4 km/hrC3.5 km/hrD6 km/hr
A man can row 34 of a km against the stream in 1114 minutes and returns in 712 minutes. Find the speed of the man in still water. a. 3 km/hr b. 4 km/hr c. 5 km/hr d. 6 km/hr
View SolutionIf a man goes 18/ km downstream in 4 hours and returns against the stream in 12 hours then the speed of the stream is km/hr is a. 1 b. 3 c. 1.5 d. 1.75
View SolutionA man can row three quarters of a km against the stream in 11 1/2 minutes and return in 7 1/3 minutes. Find the speed of the man in still water. What is the speed of the stream ?
View SolutionA man can row three quarters of a km against the stream in 11 minutes 15 seconds and return in 7 minutes 30 second. Find the speed of the man in still water and also the speed of the stream ______.
View SolutionA man can row three-quarters of a kilometre against the stream in 1114 minutes and return in 0712 minutes. The speed (in km/hr) of the man in still water is:
View Solution
