Given . If then find the value of KE?
The correct Answer is:125 J
Step by step video, text & image solution for Given KE=(1)/(2)mv^(2). If m=10kg, v=5m//s then find the value of KE? by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (VECTORS)
57 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Units, Dimensions & Measurements)
33 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Graphs))
30 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
Similar Questions
Relation between kinetic energy and momentum
Let us consider a body of mass 'm' having a velocity 'v', then
momentum of the body P = mass × velocity
P=m×v⇒v=Pm ...(1)
From definition, kinetic energy (K.E) of the body
K.E=12mv2 ...(2)
Now putting the value of (1) in (2) we have
K.E=12m(Pm)2
K.E.=12mP2m2=12P2m=P22m ...(3)
Thus we can write
P2=2m×K.E
⇒P=√2m×K.E
Thus momentum = √2×mass×kinetic energy
The kinetic energy of a given body is doubled. Its momentum will
View SolutionRelation between kinetic energy and momentum
Let us consider a body of mass 'm' having a velocity 'v', then
momentum of the body P = mass × velocity
P=m×v⇒v=Pm ...(1)
From definition, kinetic energy (K.E) of the body
K.E=12mv2 ...(2)
Now putting the value of (1) in (2) we have
K.E=12m(Pm)2
K.E.=12mP2m2=12P2m=P22m ...(3)
Thus we can write
P2=2m×K.E
⇒P=√2m×K.E
Thus momentum = √2×mass×kinetic energy
Two bodies masses 1 kg and 4 kg having equal kinetic energies. The ratio of their momentum is
View Solution
Knowledge Check
Moment of inertia of a body about a given axis is the rotational inertia of the body about that axis. It is respresented by I=MK2, where M is mass of body and K is radius of gyration of the body about that axis. It is a scalar quantity, which is measured in kgm2. when a body rotates about a given axis, and teh axis of rotates also moves, then total K.E. of body =K.E. of translation +K.E. of rotation
E=12mv2+12Iω2
Which the help of the compreshension given above, choose the most apporpriate altermative for each of the following questions :
Kinetic energy of rotation of the flywheel in the above case isA20JB20JC395JD80JMoment of inertia of a body about a given axis is the rotational inertia of the body about that axis. It is respresented by I=MK2, where M is mass of body and K is radius of gyration of the body about that axis. It is a scalar quantity, which is measured in kgm2. when a body rotates about a given axis, and teh axis of rotates also moves, then total K.E. of body =K.E. of translation +K.E. of rotation
E=12mv2+12Iω2
Which the help of the compreshension given above, choose the most apporpriate altermative for each of the following questions :
A 40kg flywheel in the from of a unifrom circular disc of diameter 1m is making 120r±. Its moment of inertia about a transverse axis through its centre isA40kgm2B5kgm2C10kgm2D20kgm2
Relation between kinetic energy and momentum
Let us consider a body of mass 'm' having a velocity 'v', then
momentum of the body P = mass × velocity
P=m×v⇒v=Pm ...(1)
From definition, kinetic energy (K.E) of the body
K.E=12mv2 ...(2)
Now putting the value of (1) in (2) we have
K.E=12m(Pm)2
K.E.=12mP2m2=12P2m=P22m ...(3)
Thus we can write
P2=2m×K.E
⇒P=√2m×K.E
Thus momentum = √2×mass×kinetic energy
Two bodies of mass 1 kg and 4 kg possess equal momentum. The ratio of their kinetic energies is
View SolutionRelation between kinetic energy and momentum
Let us consider a body of mass 'm' having a velocity 'v', then
momentum of the body P = mass × velocity
P=m×v⇒v=Pm ...(1)
From definition, kinetic energy (K.E) of the body
K.E=12mv2 ...(2)
Now putting the value of (1) in (2) we have
K.E=12m(Pm)2
K.E.=12mP2m2=12P2m=P22m ...(3)
Thus we can write
P2=2m×K.E
⇒P=√2m×K.E
Thus momentum = √2×mass×kinetic energy
Two bodies A and B of unequal masses having same momentum have masses in the ratio 1 : 2 then their K.E are in the ratio
View SolutionRelation between kinetic energy and momentum
Let us consider a body of mass 'm' having a velocity 'v', then
momentum of the body P = mass × velocity
P=m×v⇒v=Pm ...(1)
From definition, kinetic energy (K.E) of the body
K.E=12mv2 ...(2)
Now putting the value of (1) in (2) we have
K.E=12m(Pm)2
K.E.=12mP2m2=12P2m=P22m ...(3)
Thus we can write
P2=2m×K.E
⇒P=√2m×K.E
Thus momentum = √2×mass×kinetic energy
What will be the momentum of a body of mass 100 g having kinetic energy of 20 J ?
View SolutionIf in the previous problem , the final K.E. is 3/5 of the initial K.E. , find the value of e.
View SolutionIf the acceleration of the block B in the following system is a ( in m/s2) then find out value of 2a/5(g=10m/s2):
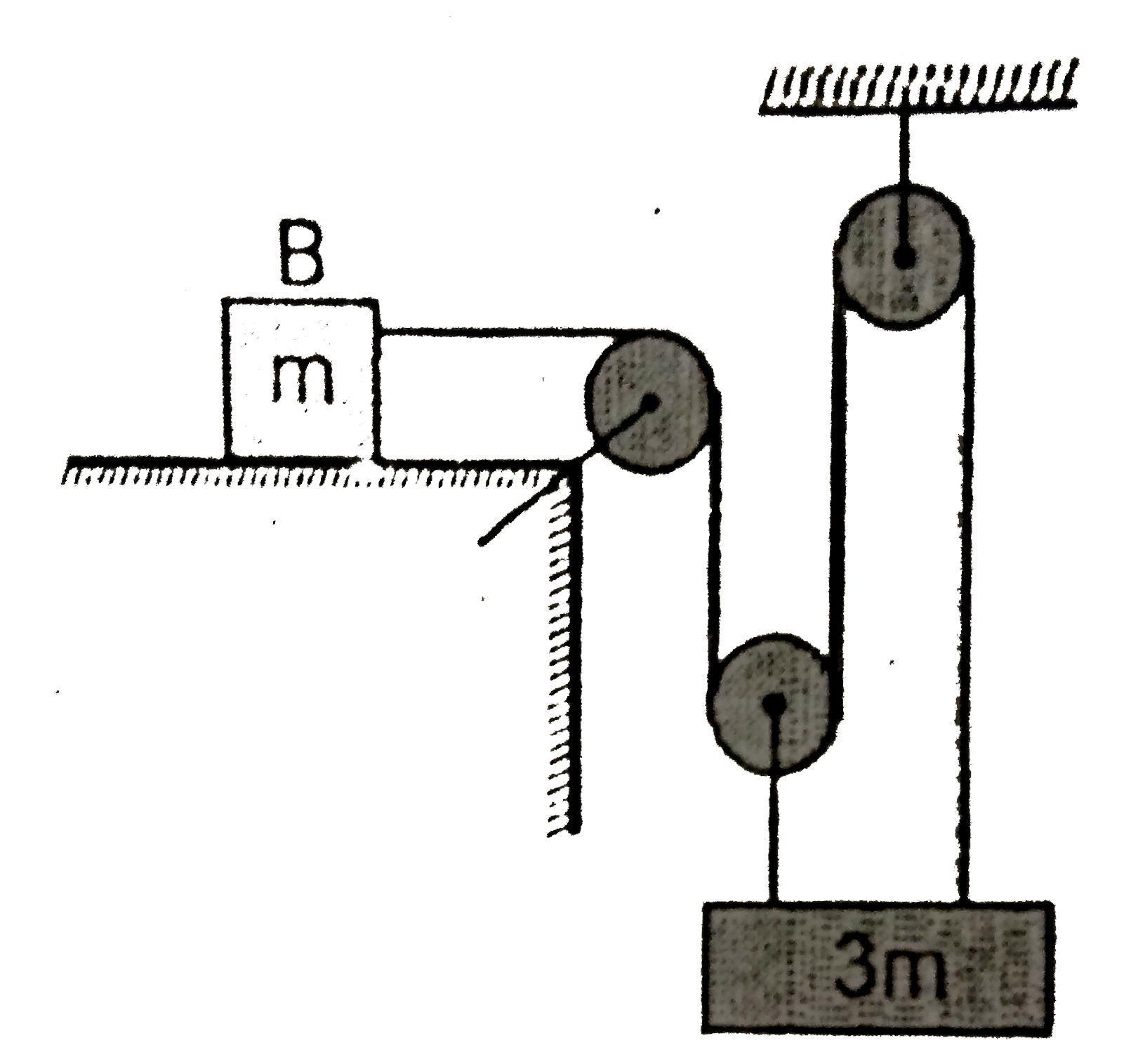
View SolutionA block of mass 2kg is sliding on a smooth surface. At t=0, its speed is v0=2m/s. At t=0, a time varying force starts acting on the block in the direction opposite to v0 if the speed of object is 38Nm/s at t=10s. Find the value of N

View Solution
