The slope of straight line √3y=3x+4 is
3
√3
1√3
13
The correct Answer is:B
To find the slope of the straight line given by the equation √3y=3x+4, we will follow these steps:
Step 1: Rewrite the equation in slope-intercept form
The slope-intercept form of a line is given by the equation:
y=mx+c
where m is the slope and c is the y-intercept. We need to isolate y in the given equation.
Starting with:
√3y=3x+4
Step 2: Divide both sides by √3
To isolate y, we divide both sides of the equation by √3:
y=3√3x+4√3
Step 3: Simplify the fraction
Now, we simplify 3√3:
3√3=√3
Thus, the equation becomes:
y=√3x+4√3
Step 4: Identify the slope
From the equation y=√3x+4√3, we can identify the slope m:
m=√3
Final Answer
The slope of the straight line √3y=3x+4 is √3.
---
The slope-intercept form of a line is given by the equation:
y=mx+c
where m is the slope and c is the y-intercept. We need to isolate y in the given equation.
√3y=3x+4
To isolate y, we divide both sides of the equation by √3:
y=3√3x+4√3
Now, we simplify 3√3:
3√3=√3
Thus, the equation becomes:
y=√3x+4√3
From the equation y=√3x+4√3, we can identify the slope m:
m=√3
The slope of the straight line √3y=3x+4 is √3.
Topper's Solved these Questions
- RACEBook:ALLENChapter:RACEExercise:Basic Maths (Co-ordinate & Algebra)
Explore 39 Videos
- RACEBook:ALLENChapter:RACEExercise:Basic Maths (VECTORS)
Explore 57 Videos
- RACEBook:ALLENChapter:RACEExercise:Basic Maths (Integration)
Explore 12 Videos
- NEWTONS LAWS OF MOTIONBook:ALLENChapter:NEWTONS LAWS OF MOTIONExercise:EXERCISE-III
Explore 28 Videos
- SIMPLE HARMONIC MOTIONBook:ALLENChapter:SIMPLE HARMONIC MOTIONExercise:Example
Explore 1 Video
Similar Questions
Knowledge Check
The graph of straight line y=√3x+2√3 is :
A B
B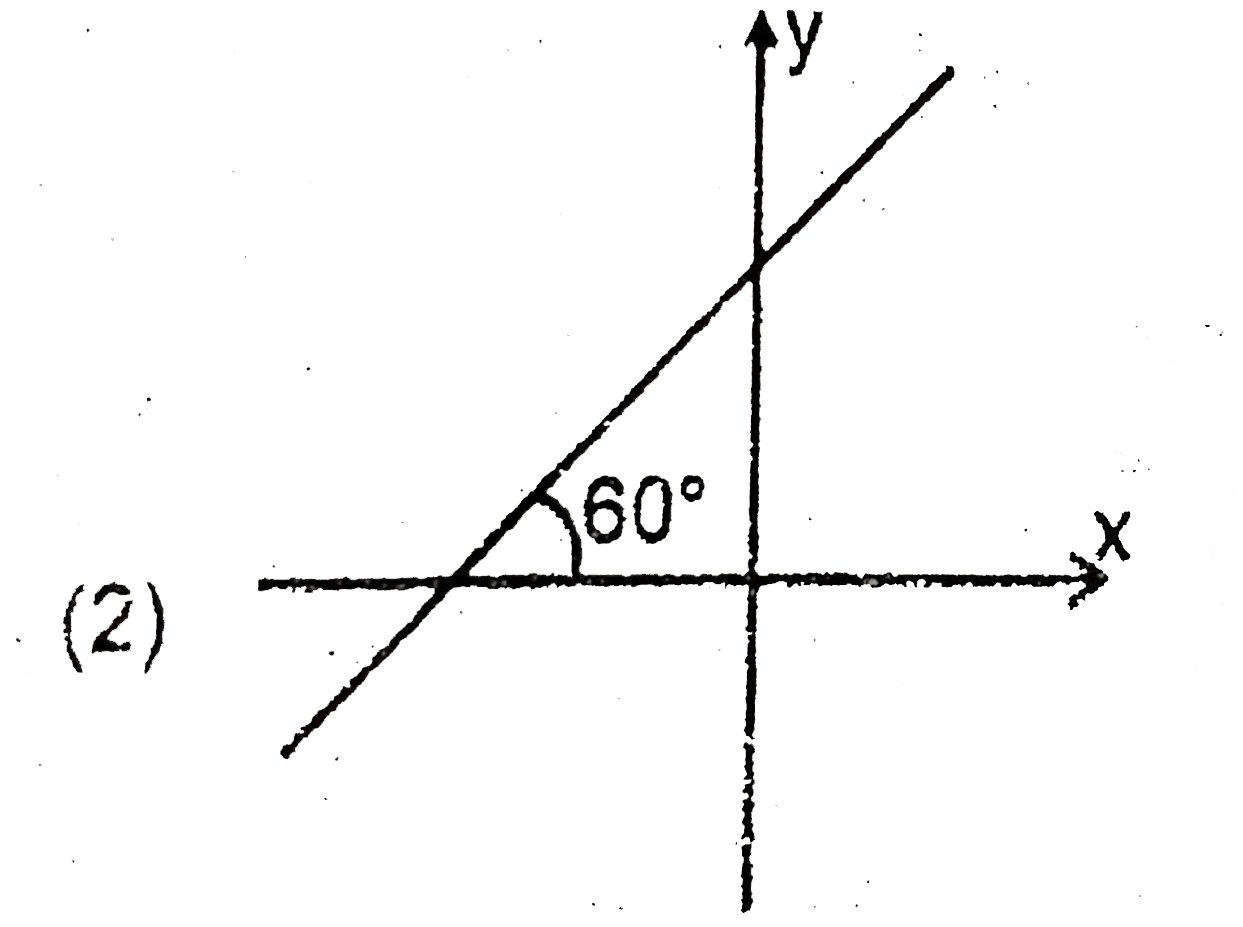 C
C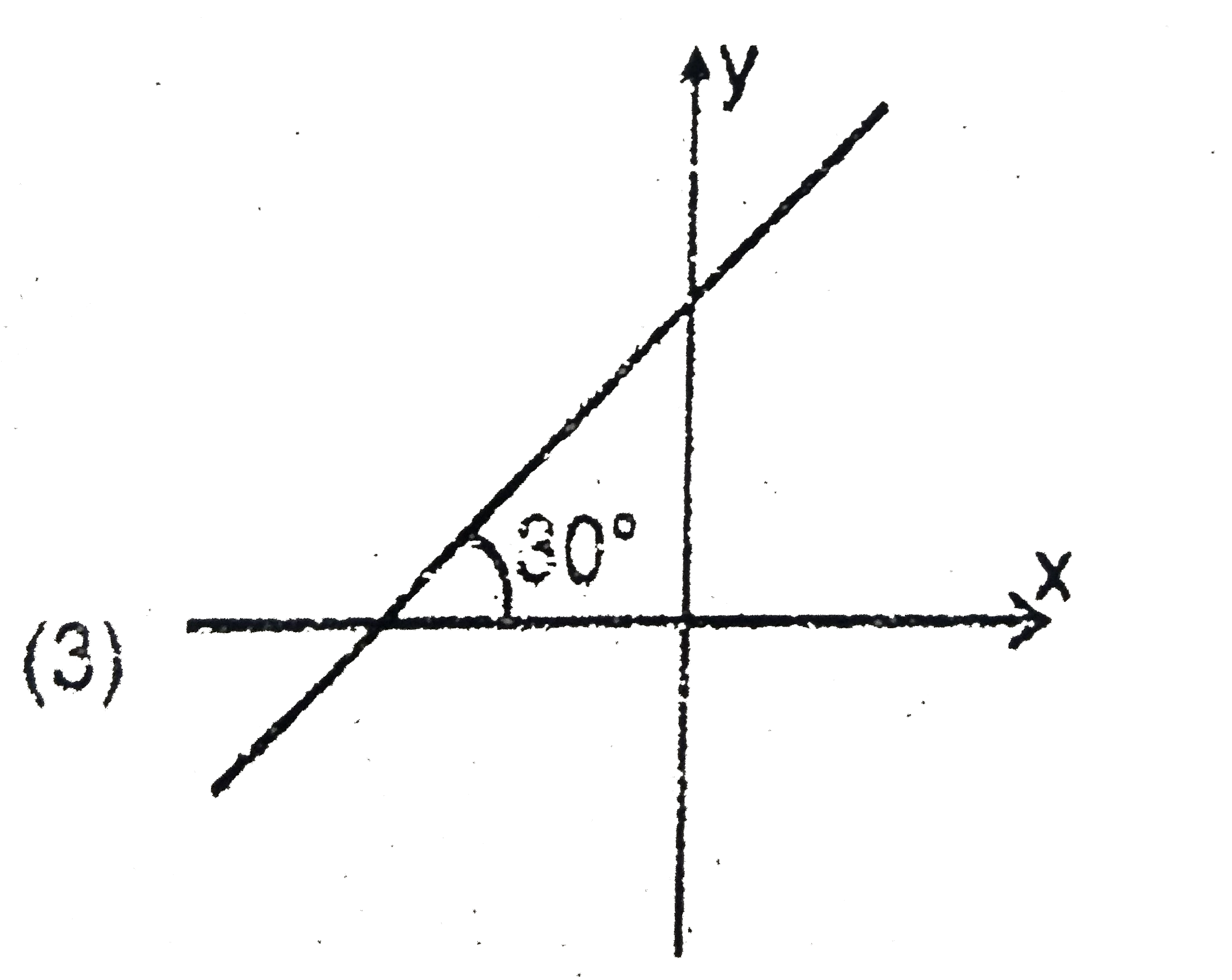 D
D
If the slope of a straight line through A(3,2) is 3/4. Then, the coordinates of the points on the line that are 5 units away from A is
A(7,5) and (-1,-1)B(-7,-5) and (1,1)C(7,1) and (5,1)DNone of these
The slope of a straight line through A(3,2)i s 3/4 Find the coordinates of the points on the line that are 5units away fromA.
View SolutionFind the angles between the pairs of straight line x−y√3=5 and √3x+y=7
View SolutionFind the slopes of straight lines (i) parallel (ii) perpendicular to the straight line 3x+4y=11.
View SolutionFind the angles between the pairs of straight lines: x+√3y−5=0 and √3x+y−7=0
View SolutionThe slope of a line 3x−y+10=0
View SolutionFor the straight line √3y−3x=3, find the intercepts on the x-axis and y-axis.
View Solution