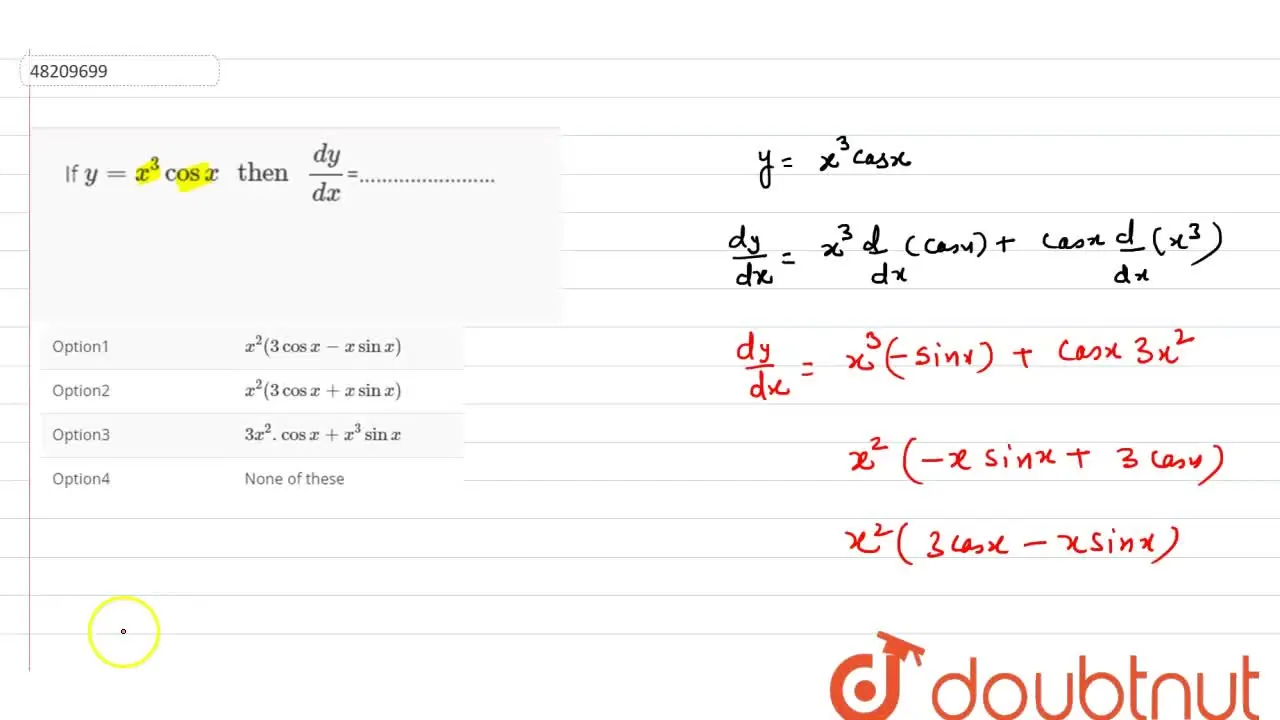
If y=x3cosx then dydx=……………………
A
x2(3cosx−xsinx)
B
x2(3cosx+xsinx)
C
3x2.cosx+x3sinx
D
None of these
Video Solution
Text Solution
The correct Answer is:A
|
Answer
Step by step video, text & image solution for If y=x^(3)cosx" then "(dy)/(dx)=…………………… by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023
Related Playlists
- BOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Integration)12videos
RACE
- BOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Graphs))30videos
RACE
- BOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Trigonometry)22videos
RACE
- BOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III28videos
NEWTONS LAWS OF MOTION
- BOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example1videos
SIMPLE HARMONIC MOTION
Similar Questions
If y=xcosx then find dydx
View SolutionIf y=sinx1+cosx then dydx=
View SolutionIf y=x3, then dydx=
View SolutionIf y=xcosx then find dydx
View SolutionIf y=cosx3, then find dydx.
View SolutionIf y=1−cosx1+cosx, then: dydx=
View SolutionIf xy=3x−y,thendydx=
View SolutionIf y=f(cosx) and f'(x)=cos−1x, then dydx=
View SolutionIf 2x+3y=cosx then find dydx
View SolutionIf y=(cosx)(cosx) then dydx is equal to :
View Solution