△XYZ is right angled at y and ∠Z=30∘ what is the length of yz if zx=9 cm
6√3
9√32
3√3
18
The correct Answer is:2
To solve the problem, we will use the properties of a right-angled triangle. Given that triangle XYZ is right-angled at Y and angle Z is 30 degrees, we need to find the length of side YZ when the hypotenuse ZX is 9 cm.
1. Identify the triangle and given values:
We have triangle XYZ where:
- Angle Y is 90 degrees (right angle)
- Angle Z is 30 degrees
- Hypotenuse ZX = 9 cm
2. Determine the angles:
Since the sum of angles in a triangle is 180 degrees:
- Angle X = 180° - Angle Y - Angle Z
- Angle X = 180° - 90° - 30° = 60°
3. Use trigonometric ratios:
In a right-angled triangle, we can use the sine and cosine functions to find the lengths of the sides. We know:
- The sine of an angle is defined as the opposite side over the hypotenuse.
For angle Z (30 degrees):
sin(Z)=YZZX
Substituting the known values:
sin(30°)=YZ9
4. Calculate sin(30°):
We know that:
sin(30°)=12
5. Set up the equation:
Now we can substitute this value into our equation:
12=YZ9
6. Solve for YZ:
To find YZ, we can multiply both sides by 9:
YZ=9×12=92=4.5 cm
Final Answer:
The length of YZ is 4.5 cm.
We have triangle XYZ where:
- Angle Y is 90 degrees (right angle)
- Angle Z is 30 degrees
- Hypotenuse ZX = 9 cm
Since the sum of angles in a triangle is 180 degrees:
- Angle X = 180° - Angle Y - Angle Z
- Angle X = 180° - 90° - 30° = 60°
In a right-angled triangle, we can use the sine and cosine functions to find the lengths of the sides. We know:
- The sine of an angle is defined as the opposite side over the hypotenuse.
sin(Z)=YZZX
sin(30°)=YZ9
We know that:
sin(30°)=12
Now we can substitute this value into our equation:
12=YZ9
To find YZ, we can multiply both sides by 9:
YZ=9×12=92=4.5 cm
The length of YZ is 4.5 cm.
Similar Questions
Knowledge Check
ΔXYZ is right angled at Y.If tan X =158,then what is the value of sinZ?
A1517B17/8C17/15D8/17△XYZis right angled at Y. If tan X = 24/7, then what is the value of cot Z?
A25/7B24/25C7/24D24/7△XYZ is right angled at Y. If ∠Z=60∘ , then find the value of (cotX - 13).
A(3√3−1)/3B(2√3−√6)/2√3C−5/3D(2−√3)/2√3
ΔXYZ is right angled at Y. If m∠Z=30∘, then find the value of (cosX−1/√3)
View SolutionΔLMNis right angled at M. If M∠N=45∘, what is the length of MN (in cm), if NL=9√2cm?
View SolutionIf the length of the sides which make the right angle of a right angled triangle are 6 cm and 8 cm, what is the length of hypotenuse?
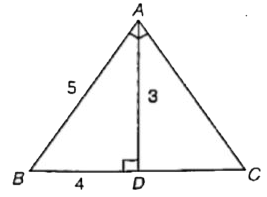
View SolutionIn the ajoining figure the angle BAC and ∠ADB are right angles .
BA=5cm,AD=3cmandBD=4cm, what is the length of DC ?
View SolutionThe length of the two sides forming the right angle of a right-angled triangle are 6 cm and 8 cm. The length of its circumradius is :
View Solution
