A common hydrometer reads specific gravity of liquids Compared to the 1.6 mark of the stem the mark 1.5 will be
upwards
downwards
in the same place
may be upward or downward depending upon the hydrometer
The correct Answer is:A
To solve the problem, we need to understand how a hydrometer works and how it indicates the specific gravity of liquids.
1. Understanding the Hydrometer: A hydrometer is an instrument used to measure the specific gravity (density relative to water) of liquids. It consists of a weighted bulb and a graduated scale.
2. Principle of Operation: The hydrometer floats in the liquid, and the level at which it floats indicates the specific gravity. According to Archimedes' principle, the buoyant force acting on the hydrometer is equal to the weight of the liquid displaced by it.
3. Specific Gravity Reading: The specific gravity is read from the scale on the hydrometer. If the liquid is less dense than water, the hydrometer will sink lower, causing the reading to be higher on the scale (closer to zero). Conversely, if the liquid is denser than water, the hydrometer will float higher, resulting in a lower reading on the scale.
4. Comparing the Marks: In the question, we are asked to compare the mark at 1.6 with the mark at 1.5. If the hydrometer is floating in a liquid that has a specific gravity less than 1.6, the hydrometer will sink lower, causing the reading to rise (the mark at 1.5 will be above the surface of the liquid).
5. Conclusion: Since the question asks where the mark 1.5 will be compared to the mark 1.6 when the hydrometer is in a liquid, and we know that a lower specific gravity causes the hydrometer to float higher, we conclude that the mark 1.5 will be upwards compared to the mark 1.6.
Thus, the answer is A. Upwards.
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF FLUIDS BOOK - DISHA PUBLICATIONCHAPTER - MECHANICAL PROPERTIES OF FLUIDS EXERCISE - EXERCISE-2 CONCEPT APPLICATOR
24 Videos
- MECHANICAL PROPERTIES OF FLUIDS BOOK - DISHA PUBLICATIONCHAPTER - MECHANICAL PROPERTIES OF FLUIDS EXERCISE - EXERCISE-2 CONCEPT APPLICATOR
24 Videos
- MAGNETISM AND MATTERBOOK - DISHA PUBLICATIONCHAPTER - MAGNETISM AND MATTEREXERCISE - EXERCISE-2:CONCEPT APPLICATOR
31 Videos
- MOTION IN A PLANE BOOK - DISHA PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - Exercise -2 : CONCEPT APPLICATOR
28 Videos
Similar Questions
A variable immersion hydrometer is used to measure the specific gravity of two liquids When the hydrometer reads 0.8, half of the total length of the hydrometer stem is immersed in the liquid and when the hydrometer reads 0.6,3/4 th of the total length of the hydrometer stem is immersed in the liquid. What is the maximum and minimum reading that can be measured by using this hydrometer ?
View SolutionA device used to measure the specific gravity of a liquid is called a hydrogmeter. In a simple hydrometer there is a cylindrical galss tube with some lead-weight at its bottom. The device floats in liquid while remaining vertical. The top part of the tube extends above the liquid and the divisions marked on the tube allows one to directly read the specific gravity of the liquid.
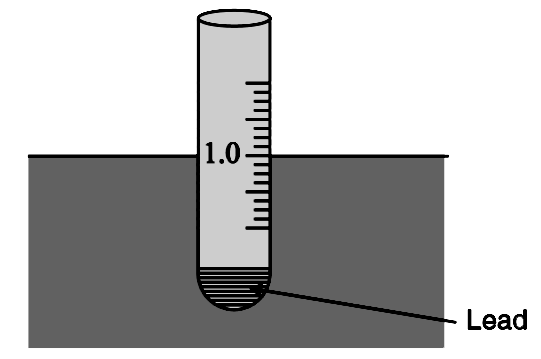
The scale on the tube is calibrated such that in pure water it reads 1.0 at the water surface and a lenght z0 of the tube is submerged. calculate the specific gravity of the liquid if the liquid level is ΔZ above the 1.0 mark. Disregard the curvature of the tube bottom.
View Solution
Knowledge Check
If S1 is the specific gravity of a solid with respect to a liqid and S2 is the specific gravity of the liquid with respect to water, then the specific gravity of the solid with respect to water is
AS1+S2BS1×S2CS1−S2DS1S2If a liquid of specific fravity 1 , rises to a height of 2.5 cm in a capillary tube , then another liquid of specific gravity 1.25 will rise to the height in the same tube will be
A1 cmB2 cmC1.25 cmD1.5 cmA solid uniform ball of volume V floats on the interface of two immiscible liquids (see the figure). The specific gravity of the upper liquid is ρ1 and that of lower one is ρ2 and the specific gravity of ball is ρ(ρ1>ρ>ρ2) The fraction of the volume the ball in the u of upper liquid is
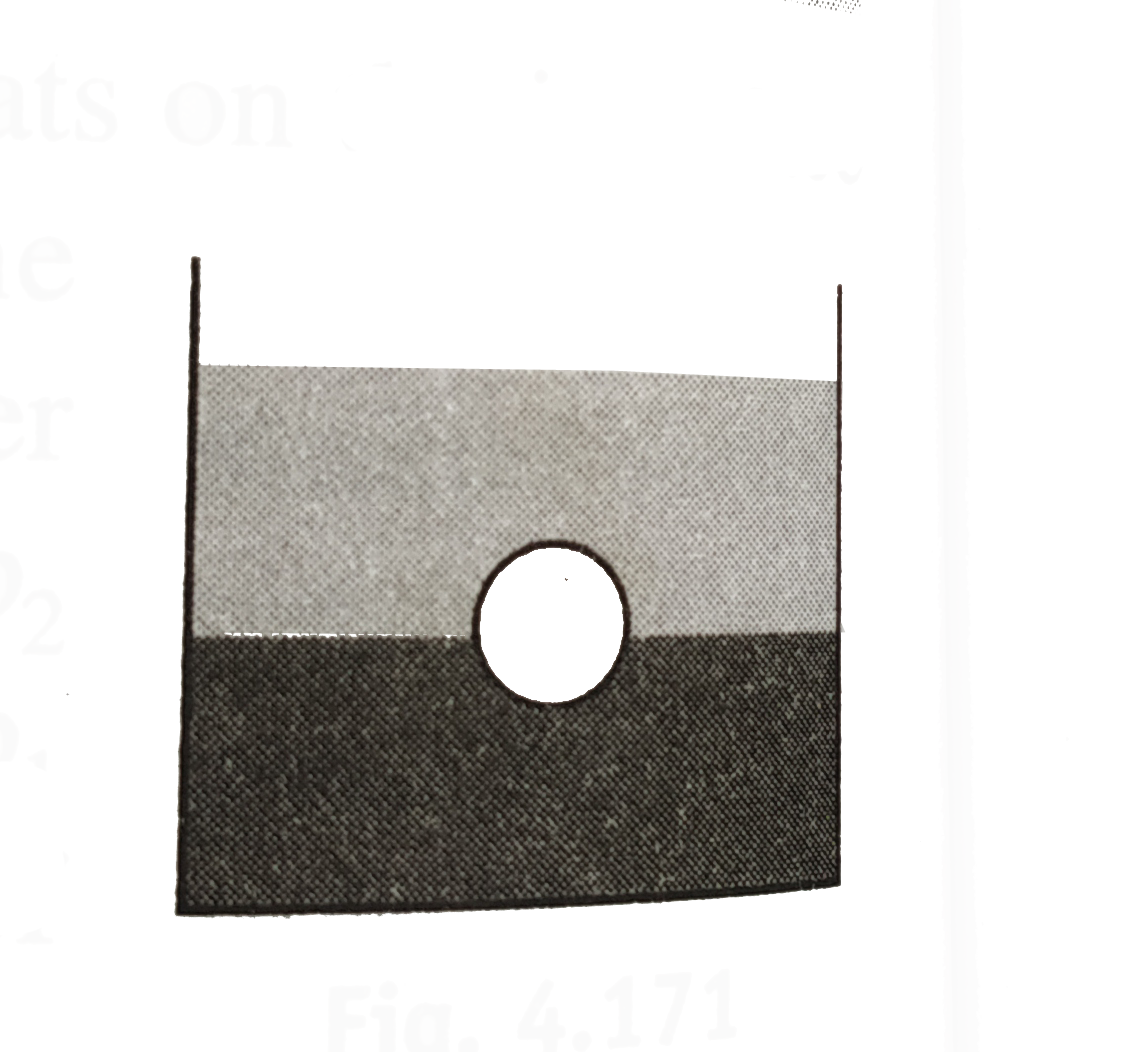 Aρ2ρ1Bρ2−ρρ2−ρ1Cρ−ρ1ρ2−ρ1Dρ1ρ2
Aρ2ρ1Bρ2−ρρ2−ρ1Cρ−ρ1ρ2−ρ1Dρ1ρ2
A hydrometer stem has a length 30 cm. If the hydrometer is immersed in water , its floatation bulb just sinks. If the same hydrometer is immersed in a liquid having density of 500kgm−3, two-third of the stem is immersed. Find the least specific gravity of a liquid that can be measured using the hydrometer.
View SolutionA hydrometer stem has a length 30 cm. If the hydrometer is immersed in water , its floatation bulb just sinks. If the same hydrometer is immersed in a liquid having density of 500kgm−3, two-third of the stem is immersed. Find the least specific gravity of a liquid that can be measured using the hydrometer.
View SolutionA hydrometer stem has a length 30 cm. If the hydrometer is immersed in water , its floatation bulb just sinks. If the same hydrometer is immersed in a liquid having density of 500kgm−3, two-third of the stem is immersed. Find the least specific gravity of a liquid that can be measured using the hydrometer.
View SolutionA camera lens is marked f/1.8. What is the meaning of this mark?
View SolutionA glass hydrometer reads 9200 in a liquid at 45∘C. What would be the reading at 15∘C? (Cubical expansivity of the liquid =52.×10−5/K and that of glass =2.4×10−5/K)
View Solution
