Question

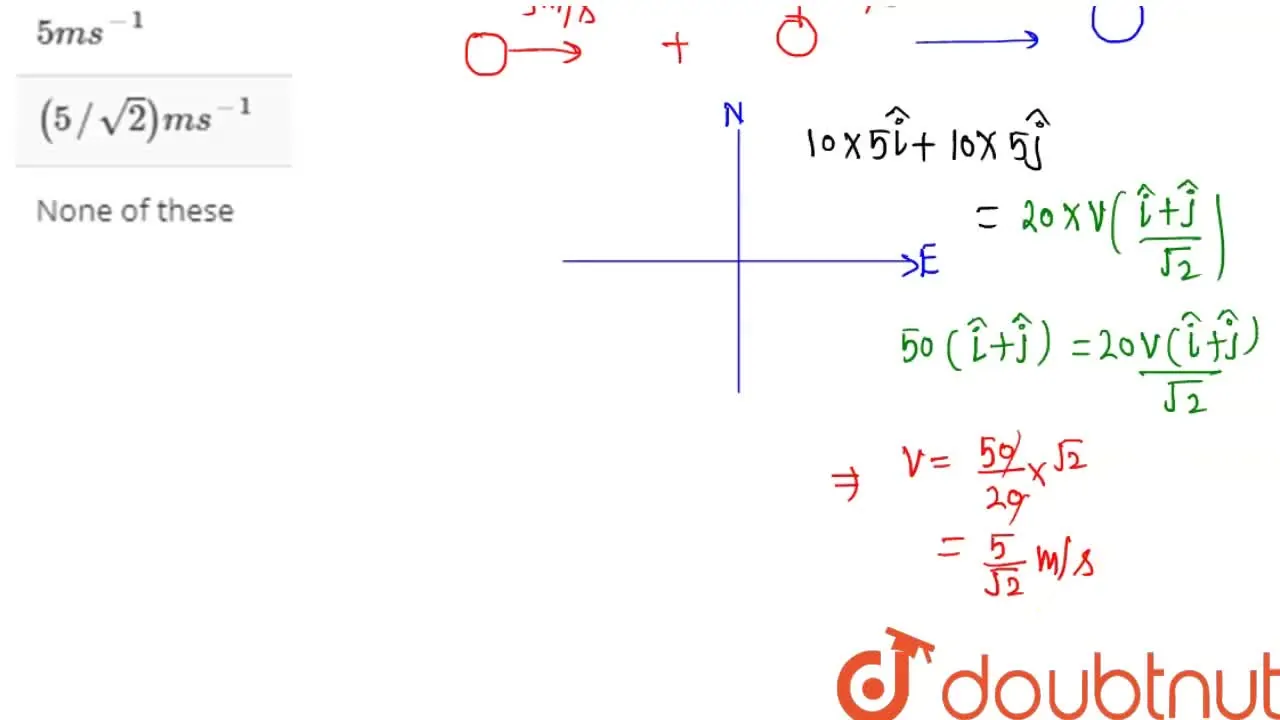
A particle of mass 10 kg moving eastwards with a speed 5 $m{s}^{-1}$ collides with another particle of the same mass moving north-wards with the same speed 5$m{s}^{-1}$ .The two particles coalesce on collision.The new particle of mass 20 kg will move in the north-east direction with velocity

Options

A. `10 ms^(-1)`
B. `5 ms^(-1)`
C. `(5//sqrt(2))ms^(-1)`
D. None of these

Answer

C

Explanation

To solve the problem, we need to determine the velocity of the new particle formed after the collision of the two particles. Let's break down the steps:

Step 1: Identify the initial conditions
- Mass of particle 1 (m1) = 10 kg, moving east with speed (v1) = 5 m/s.
- Mass of particle 2 (m2) = 10 kg, moving north with speed (v2) = 5 m/s.

Step 2: Determine the momentum of each particle
- Momentum of particle 1 (P1) = m1 * v1 = 10 kg * 5 m/s = 50 kg·m/s (east direction).
- Momentum of particle 2 (P2) = m2 * v2 = 10 kg * 5 m/s = 50 kg·m/s (north direction).

Step 3: Calculate the total momentum before collision
Since the two particles are moving at right angles to each other, we can treat their momenta as vectors:
- Total momentum in the east direction (x-direction) = P1 = 50 kg·m/s.
- Total momentum in the north direction (y-direction) = P2 = 50 kg·m/s.

Step 4: Combine the momenta to find the resultant momentum
The total momentum vector (P_total) can be found using the Pythagorean theorem:
\( P_{\text{total}} = \sqrt{(P1)^2 + (P2)^2} = \sqrt{(50)^2 + (50)^2} = \sqrt{2500 + 2500} = \sqrt{5000} = 50\sqrt{2} \text{ kg·m/s}. \)

Step 5: Determine the mass of the new particle
After the collision, the two particles coalesce, so the total mass (M) of the new particle is:
\( M = m1 + m2 = 10 \text{ kg} + 10 \text{ kg} = 20 \text{ kg}. \)

Step 6: Calculate the velocity of the new particle
Using the conservation of momentum, the velocity (v) of the new particle can be calculated as:
\( P_{\text{total}} = M \cdot v \)
\( 50\sqrt{2} = 20 \cdot v \)
\( v = \frac{50\sqrt{2}}{20} = \frac{5\sqrt{2}}{2} \text{ m/s}. \)

Step 7: Determine the direction of the new particle
The new particle moves in the northeast direction, which is at a 45-degree angle to both the east and north directions.

Final Answer
The new particle will move in the northeast direction with a velocity of \( \frac{5\sqrt{2}}{2} \text{ m/s} \).

---