The solution set of the inequality 37−(3x+5)≥9x−8(x−3) is
(−∞,2)
(−∞,−2)
(−∞,2]
(−∞,−2]
The correct Answer is:(c )
To solve the inequality 37−(3x+5)≥9x−8(x−3), we will follow these steps:
Step 1: Simplify both sides of the inequality
Start by distributing and simplifying the left and right sides of the inequality.
Left side:
37−(3x+5)=37−3x−5=32−3x
Right side:
9x−8(x−3)=9x−8x+24=x+24
Now, the inequality becomes:
32−3x≥x+24
Step 2: Rearrange the inequality
Next, we will move all terms involving x to one side and constant terms to the other side.
Add 3x to both sides:
32≥x+3x+24
32≥4x+24
Now, subtract 24 from both sides:
32−24≥4x
8≥4x
Step 3: Solve for x
Now, divide both sides by 4:
84≥x
2≥x
or
x≤2
Step 4: Write the solution set
The solution set for the inequality x≤2 can be expressed in interval notation as:
(−∞,2)
Final Answer
Thus, the solution set of the inequality is:
x∈(−∞,2)
Start by distributing and simplifying the left and right sides of the inequality.
37−(3x+5)=37−3x−5=32−3x
9x−8(x−3)=9x−8x+24=x+24
32−3x≥x+24
Next, we will move all terms involving x to one side and constant terms to the other side.
32≥x+3x+24
32≥4x+24
32−24≥4x
8≥4x
Now, divide both sides by 4:
84≥x
2≥x
or
x≤2
The solution set for the inequality x≤2 can be expressed in interval notation as:
(−∞,2)
Thus, the solution set of the inequality is:
x∈(−∞,2)
Topper's Solved these Questions
- LINEAR INEQUALITIESBOOK - DISHA PUBLICATIONCHAPTER - LINEAR INEQUALITIESEXERCISE - Exercise -1 :Concept Builder (Topic 2)
2 Videos
- LINEAR INEQUALITIESBOOK - DISHA PUBLICATIONCHAPTER - LINEAR INEQUALITIESEXERCISE - Exercise -1 : Concept Builder (Topic 3)
7 Videos
- LINEAR INEQUALITIESBOOK - DISHA PUBLICATIONCHAPTER - LINEAR INEQUALITIESEXERCISE - Exercise -2 : Concept Applicator
30 Videos
- LIMITS AND DERIVATIVESBOOK - DISHA PUBLICATIONCHAPTER - LIMITS AND DERIVATIVESEXERCISE - Exercise -2 : Concept Applicator
30 Videos
- MATHEMATICAL REASONINGBOOK - DISHA PUBLICATIONCHAPTER - MATHEMATICAL REASONINGEXERCISE - Exercise-2 : Concept Applicator
30 Videos
Similar Questions
Knowledge Check
The solution set of the inequality 37−(3x+5)≥9x−8(x−3) is
A(−∞,2)B(−∞,−2)C(−∞,2)D(−∞,−2)The solution of inequality 37−(3x+5)≥9x−8(x−3) on number line is
A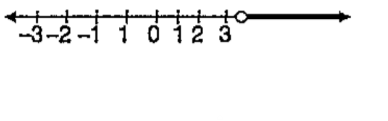 B
B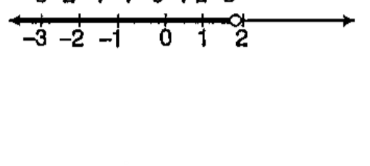 C
C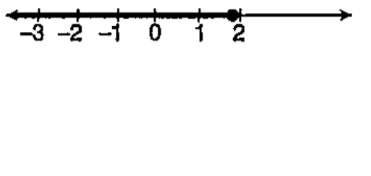 DNone of the above
DNone of the aboveThe solution set of the inequality 3(2−x)≥2(1−x) is …B…. Here, B refers to
A(−∞,4)B(−∞,4]C(−∞,−4)D(−∞,−4]
The solution set of the inequality 3(2−x)≥2(1−x) is ...B… Here, B refers to
View SolutionThe solution set of the inequation x+2y≥3 is
View SolutionThe solution set of the inequality 4x+3<6x+7 is
View SolutionThe solution set of the inequality (x+3)5−(x−1)5≥244 is
View SolutionThe solution set of the inequality log58(2x2−x−38)≥1 is-
View Solution
