Investigate for the maxima and minima of the function f(x)=∫x1[2(t−1)(t−2)3+3(t−1)2(t−2)2]dt
Video Solution
Text Solution
The correct Answer is:At x=1and75, f (x) is maximum and minimum respectively.
Given , f(x)=∫x1[2(t−1)(t−2)3+3(t−1)2(t−2)2]dt
∴f'(x)=[2(x−1)(x−2)3+3(x−1)2(x−2)]⋅1−0
=(x−1)(x−2)2[2(x−2)+3(x−1)]
=(x−1)(x−2)2(5x−7)
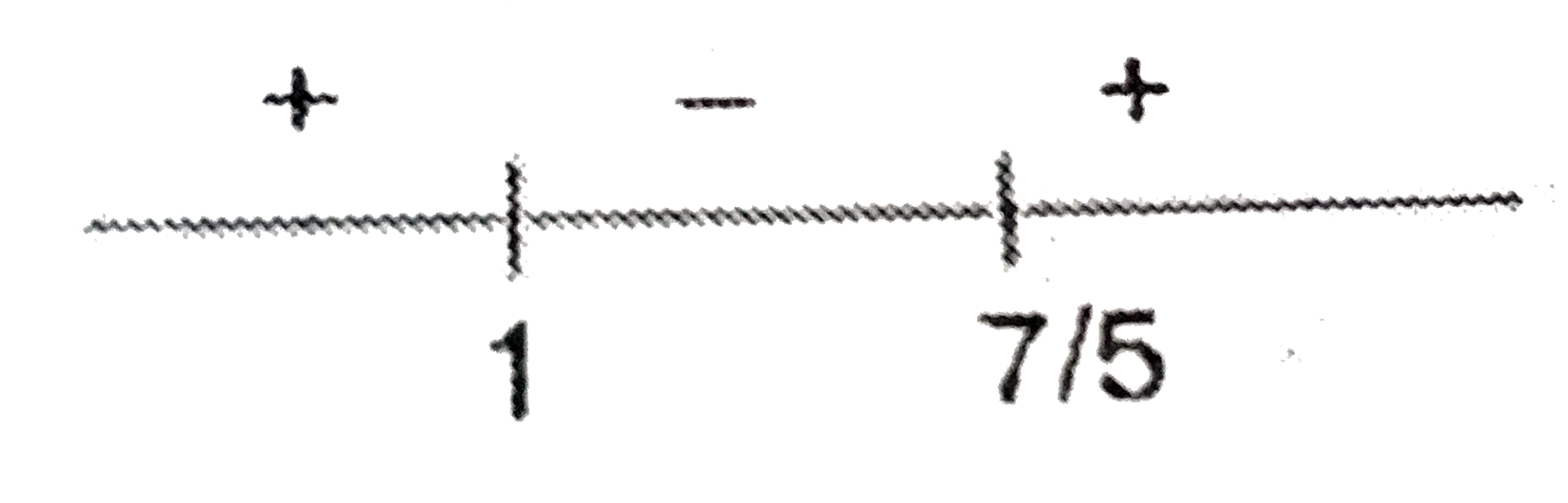
∴ f (x) attains maximum at x = 1 and f (x) attains mimimum at x=75.
|
Topper's Solved these Questions
- DEFINITE INTEGRATIONBook:IIT JEE PREVIOUS YEARChapter:DEFINITE INTEGRATIONExercise:LIMITS AS THE SUM
Explore 6 Videos
- DEFINITE INTEGRATIONBook:IIT JEE PREVIOUS YEARChapter:DEFINITE INTEGRATIONExercise:PERIODICITY OF INTEGRAL FUNCTIONS
Explore 6 Videos
- COMPLEX NUMBERSBook:IIT JEE PREVIOUS YEARChapter:COMPLEX NUMBERSExercise:TOPIC 5 DE-MOIVRES THEOREM,CUBE ROOTS AND nth ROOTS OF UNITY (INTEGER ANSWER TYPE QUESTION)
Explore 1 Video
- DIFFERENTIAL EQUATIONS Book:IIT JEE PREVIOUS YEARChapter:DIFFERENTIAL EQUATIONS Exercise:TOPIC 3 APPLICATIONS OF HOMOGENEOUS DIFFERENTIAL EQUATIONS <br> ANALYTICAL & DESCRIPTIVE QUESTIONS
Explore 7 Videos
Similar Questions
Knowledge Check
The points of extremum of the function F(x)=∫x1e−t2(1−t2)dt are
Ax=0Bx=1Cx=1/2Dx=−1The poins of extremum of ϕ(x)=x∫1e−t2/2(1−t2)dt are
Ax = 0Bx = 1Cx = 1/2Dx = -1The function F(x)=∫x0log(t+√1+t2)dt is
Aan even functionBan odd functionCa periodic functionDNone of these
The number of critical points of the functionf(x)=∫x0et(t−1)(t−2)(t−3)dt
View SolutionThe range of the function f(x)=∫x1|t|dt,x∈[−12,12] is
View SolutionLet F(x)=∫x0(t−1)(t−2)2dt, then
View SolutionFind the points of minima for f(x)=∫x0t(t−1)(t−2)dt
View SolutionThe point of extremum of f(x)=∫x0(t−2)2(t−1)dt is a
View Solution