$\int_{0}^{π} {| cos x |}^{3} d x$ is equal to (a) $\frac{4}{3}$ (b) $\frac{2}{3}$ (c) $0$ (d) $\frac{- 8}{3}$

A

$\frac{2}{3}$

B

$- \frac{4}{3}$

C

0

D

$\frac{4}{3}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

We know , graph of y = cos x is

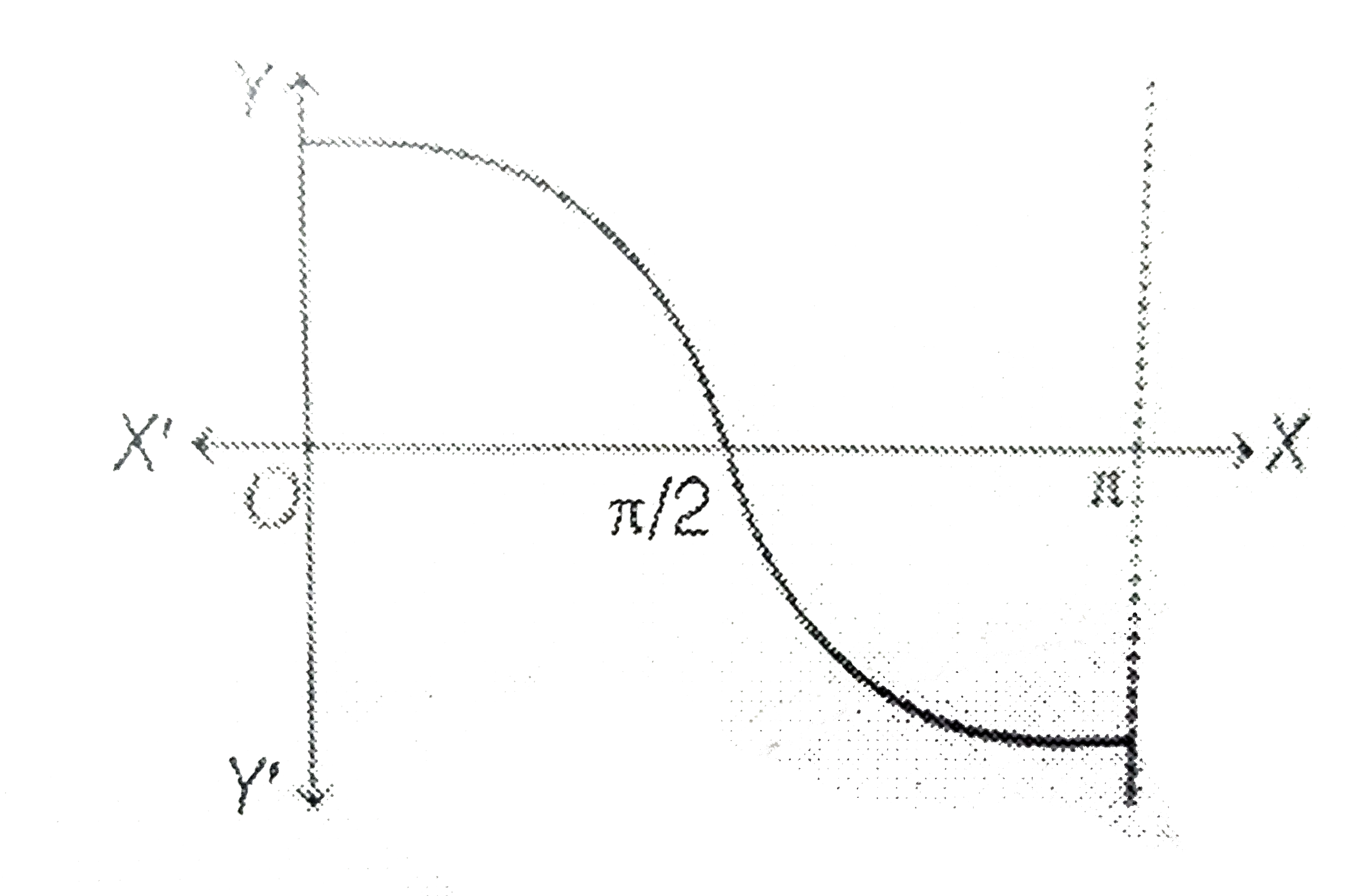
$∴$ The graph of y= | cos x | is

$I = \int_{0}^{π} {| cos x |}^{3} = 2 \int_{0}^{\frac{π}{2}} {| cos x |}^{3} d x$
( $∵ y = | cos x | is symmetric about x = \frac{π}{2})$
$= 2 \int_{0}^{\frac{π}{2}} {cos}^{3} x d x [∵ cos x \geq 0 for x \in [0, \frac{π}{2}]]$
Now , as cos $3 x = 4 {cos}^{3} x - 3 cos x$
$∴ {cos}^{3} x = \frac{1}{4} (cos 3 x + 3 cos x)$
$∴ I = \frac{2}{4} \int_{0}^{\frac{π}{2}} (cos 3 x + 3 cos x) d x$
$= \frac{1}{2} {[\frac{sin 3 x}{3} + 3 sin x]}_{0}^{\frac{π}{2}}$
$= \frac{1}{2} {[\frac{1}{2} \frac{sin (3 π)}{2} + 3 \frac{sin (π)}{2}] - [\frac{1}{3} sin 0 + 3 sin 0]}$
$= \frac{1}{2} {[\frac{1}{3} (- 1) + 3] - [0 + 0]}$
[ $∵ sin \frac{3 π}{2} sin (π + \frac{π}{2}) = - sin \frac{π}{2} = - 1]$
$= \frac{1}{2} [- \frac{1}{2} + 3] = \frac{4}{3}$

Updated on:21/07/2023

|

Class 12 MATHS DEFINITE INTEGRAL

Topper's Solved these Questions

Similar Questions

Knowledge Check

$\int_{0}^{π / 2} cos x e^{sin x} d x$ is equal to
A $e + 1$
B $e - 1$
C $e$
D $- e$
$\int_{0}^{\frac{π}{2}} | sin x - cos x | d x$ is equal to
A $0$
B $2 (\sqrt{2} - 1)$
C $2 \sqrt{2}$
D $2 (\sqrt{2} + 1)$
$\int_{0}^{π} \frac{1}{1 + 3^{cos x}}$ dx is equal to
A $π$
B0
C $π / 2$
Dnone of these

IIT JEE PREVIOUS YEAR-DEFINITE INTEGRATION-LIMITS AS THE SUM

Home
Ask Doubt
Profile