If the angles of elevation of the top of a tower from three collinear points A, B and C, on a line leading to the foot of the tower, are 300 , 450 and 600 respectively, then the ratio, AB : BC, is : (1) √3:1 (2) √3:√2 (3) 1:√3 (4) 2:3
√3:1
√3:√2
1:√3
2:3
The correct Answer is:A
According to the given information, the figure should be as follows:
Let the height of tower =h
In △EDA,tan30∘=EDAD
1√3=EDAD=hAD
⇒AD=h√3
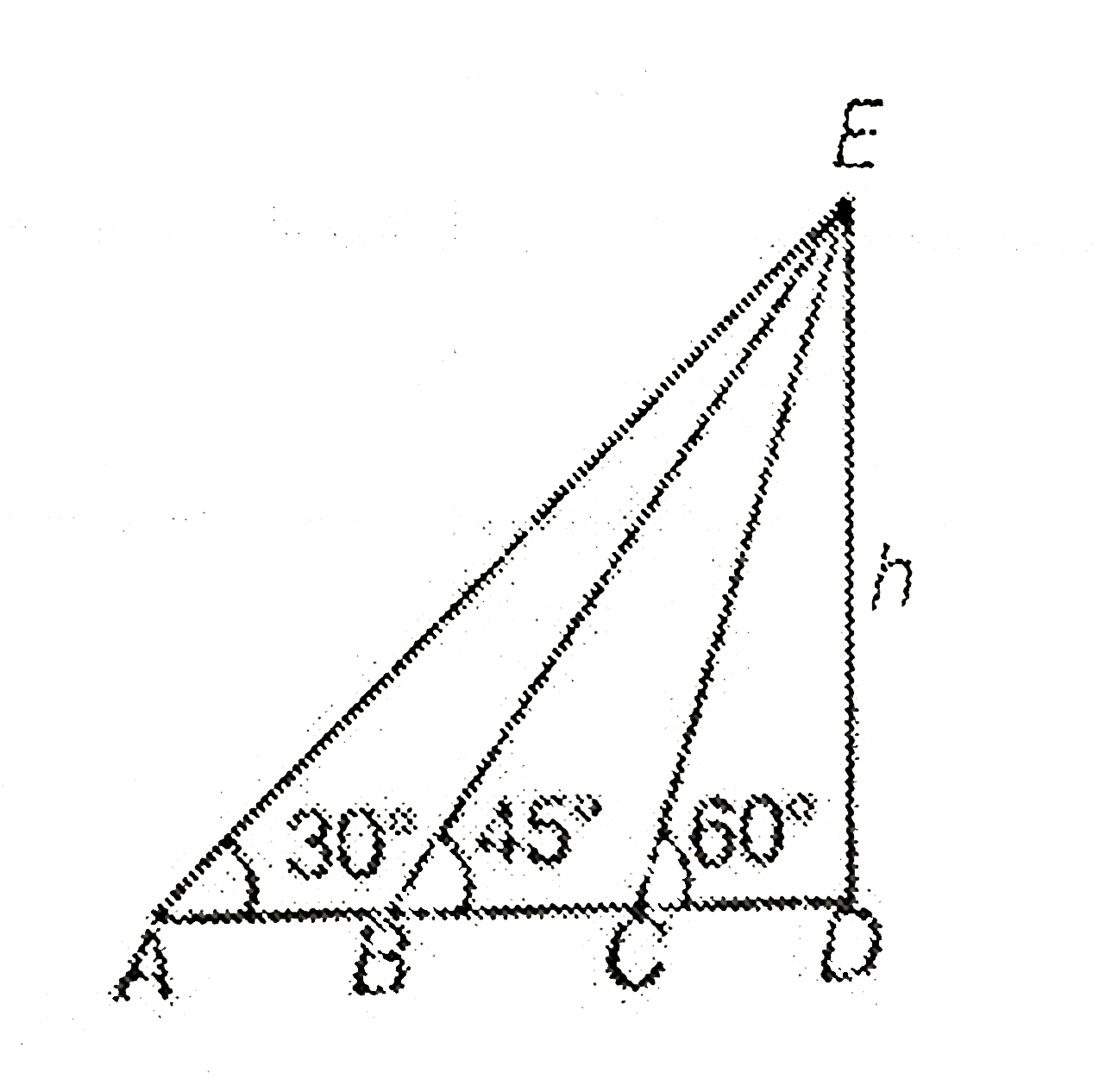
In △EDB,tan45∘=hBD⇒BD=h
In △EDC,tan60∘=hCD⇒CD=h√3
Now, ABBC=AD−BDBD−CD⇒ABBC=h√3−hh−h√3
⇒ABBC=h(√3−1)h(√3−1)√3⇒ABBC=√3−1(√3−1)×√3
⇒ABBC=√31∴AB:BC=√3:1
Topper's Solved these Questions
- MISCELLANEOUSBook:IIT JEE PREVIOUS YEARChapter:MISCELLANEOUSExercise:MISCELLANEOUS
Explore 87 Videos
- MATRICES AND DETERMINANTSBook:IIT JEE PREVIOUS YEARChapter:MATRICES AND DETERMINANTSExercise:SOLVING SYSTEM OF EQUATIONS (INTEGER ANSWER TYPE QUESTION)
Explore 2 Videos
- PARABOLABook:IIT JEE PREVIOUS YEARChapter:PARABOLAExercise:TOPIC 4 DIAMETER, CHORD OF CONTACT, CHORD BISECTED AND PRODUCT OF PAIR OF TANGENTS OBJECTIVES QUESTION II (ANALYTICAL & DESCRIPTIVE )
Explore 5 Videos
Similar Questions
The angles of elevation of the top of a TV tower from three points A, B and C in a straight line (in thehorizontal plane) through the foot of tower are α,2αand3α respectively. If AB=a, the height of tower is
View SolutionWhat is the angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of a tower of height 10√3m?
View Solution
Knowledge Check
If the angles of elevation of the top of tower from three collinear points A, B and C, on a line leading to the foot of the tower, are 30∘, 45∘ and 60∘ respectively, then the ratio , AB:BC is
A√3:1B√3:√2C1:√3D2:3Angles of elevation of the top of a tower from three point (collinear) A, B and C on a road leading to the food of the tower are 30∘,45∘ and 60∘ respectively. The ratio of AB to BC is
A√3:1B√3:2C1:2D2:√3The angle of elevation of the top of a TV tower from three points A,B and C in a straight line through the foot of the tower are α,2αand 3α, respectively. If AB−α, then height of the tower is
AatanαBasinαCasin2αDasin3α
The angle of elevation of the top of two vertical towers as seen from the middle point of the line joining the foot of the towers are 60∘and30∘ respectively. The ratio of the heights of the tower is
View SolutionThe angle of elevation of the top of a T.V. tower from three points A,B,C in a straight line in the horizontal plane through the foot of the tower are α,2α,3α respectively. If AB=a, the height of the tower is
View SolutionThe angle of elevation of the top of a T.V. tower from three points A,B,C in a straight line in the horizontal plane through the foot of the tower are α,2α,3α respectively. If AB=a, the height of the tower is
View SolutionThe angle of elevation of the top of a tower of height 100√3m from a point at a distance of 100 m from the foot of the tower on a horizontal plane is
View SolutionThe angle of elevation of the top of a tower of height 100√3m from a point at a distance of 100 m from the foot of the tower on a horizontal plane is
View Solution