Let be continuous functions which are twice differentiable on the interval (-1, 2). Let the values of f and g at the points –1, 0 and 2 be as given in the following table : In each of the intervals (-1,0) and (0, 2) the function (f – 3g)'' never vanishes. Then the correct statement(s) is(are)
has exctly three solution in
=0 has exactly one solution in (-1,0)
f'(x)-3g'(x)=0 has exactly one solution in (0,2)
f'(x)-3g'(x)=0 has exactly one solution in (-1,0) and exactly one solution in (0,2)
The correct Answer is:D
Let We have,
By Rolle's theorem, F'(x) vanishes at least once in each of the interva [-1,0] and [0,2]
Now,
It is given that never vanishes. Therefore,
is either increasing or decreasing in (-1,0( and (0,2) .
0 has exactly on solution in (-1,0) and one solution in (0,2)
has exctly one solution in (-1,0)n adn exactly one solution in (0,2)
Topper's Solved these Questions
- MEAN VALUE THEOREMSBOOK - OBJECTIVE RD SHARMACHAPTER - MEAN VALUE THEOREMSEXERCISE - Section II - Assertion Reason Type
4 Videos
- MEAN VALUE THEOREMSBOOK - OBJECTIVE RD SHARMACHAPTER - MEAN VALUE THEOREMSEXERCISE - Exercise
28 Videos
- MEAN VALUE THEOREMSBOOK - OBJECTIVE RD SHARMACHAPTER - MEAN VALUE THEOREMSEXERCISE - Exercise
28 Videos
- MATRICESBOOK - OBJECTIVE RD SHARMACHAPTER - MATRICESEXERCISE - Chapter Test
30 Videos
- PAIR OF STRAIGHT LINESBOOK - OBJECTIVE RD SHARMACHAPTER - PAIR OF STRAIGHT LINESEXERCISE - Chapter Test
19 Videos
Similar Questions
Statement-1 : The equation 3x5+15x−18=0 has exactly one real root.
Statement-2: Between any two roots of , there is a root of its derivative f'(x) .
View SolutionLet a and b be two distinct roots of a polynomial equation f(x) =0 Then there exist at least one root lying between a and b of the polynomial equation
View SolutionLet a and b be two distinct roots of a polynomial equation f(x) =0 Then there exist at least one root lying between a and b of the polynomial equation
View SolutionIf A is an invertible matrix and B is a matrix, then
View SolutionIf the lines given by ax2+2hxy+by2=0 are equally inclined to the lines given by ax2+2hxy+by2+λ(x2+y2)=0, then
View SolutionLet f:R→(0,∞) and g:R→R be twice differentiable functions such that f" and g" are continuous functions on R. suppose f′(2)=g(2)=0,f(2)≠0 and g'(2)≠0, If limx→2f(x)g(x)f'(x)g'(x)=1 then
View SolutionLet f,g:[-1,2]rarr RR be continuous functions which are twice differentiable on the interval (-1, 2). Let the values of f and g at the points –1, 0 and 2 be as given in the following table : x=-1 x=0 x=2 f(x) 3 6 0 g(x) 0 1 -1 In each of the intervals (-1,0) and (0, 2) the function (f – 3g)' never vanishes. Then the correct statement(s) is(are)
View SolutionLet f:(−5,5)→R be a differentiable function of with f(4)=1,f'(4)=1,f(0)=−1andf'(0)=Ifg(x)=(f(2f2(x)+2))2, then g'(0) equals
View SolutionLet f:(−5,5)→R be a differentiable function of with f(4)=1,f'(4)=1,f(0)=−1andf'(0)=Ifg(x)=(f(2f2(x)+2))2, then g'(0) equals
View SolutionLet f,g:[−1,2]→ be continuous functions which are twice differentiable on the interval (-1,2). Let the values of f and g at the points and 2 be as given in the following table:
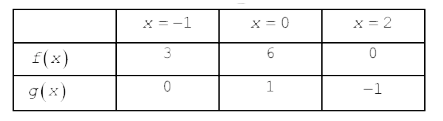
In each of the intervals (-1,0) and (0, 2) the function (f-3g) never vanishes. Then the correct statements(s) is(are) :
View Solutionमाना कि f:(−1,1)→R एक अवकलनीय फलन है जिसके लिए f(0) = - 1 और f'(0) = 1. माना कि g(x)=[f{2f(x)+2}]2, तब g'(0) =
View SolutionLet f:R→(0,∞) and g:R→R be twice differentiable functions such that f'' and g'' are continuous functions on R. Suppose f'(2)=g(2)=0,f''(2)≠0 and g'(2)≠0. If limx→2f(x)g(x)f'(x)g'(x)=1. Then
View Solutionमाना कि f, g:[−1,2]→R सतत फलन है जो कि अंतराल (−1,2) में दो बार अवकलनीय है। माना कि f और g के मान , बिन्दुओ −1, 0 और 2 पर निम्न सारणी में दर्शाए गए है-
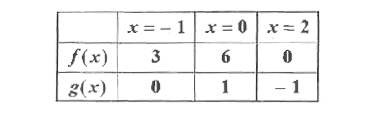
यदि प्रत्येक अंतराल (−1,0) और (0,2) में फलन (f−3g)'' कभी भी शून्य का मान नहीं लेता है, तब सही कथन है (हैं)-
View Solutionमाना f,g:[−1,2]→R सतत फलन हैं जोकि अंतराल (-1, 2) में दो बार अवकलनीय (twice differentiable) है | माना f और g के मान, बिन्दुओ -1, 0 और 2 पर निम्न सारणी में दर्शाए गए हैं
यदि प्रत्येक अंतराल (-1, 0) और (0, 2) में फलन (f - 3g)'' कभी भी शून्य का मान नहीं लेता है, तब सही कथन है (हैं)
x=−1x=0x=2f(x) 3 6 0g(x) 0 1 −1
View Solutionफलन f(x) और g(x) अन्तराल [0,1] में अवकलनीय इस प्रकार है कि f(0)=2,g(0)=0,f(1)=6,g(1)=2, तो रोले प्रमेय अन्तराल [0,1] में निम्न में से किसमें लागू होगा ?
View SolutionLet f,g:[−1,2]→R be continuous functions which are twice differentiable on the interval (-1, 2). Let the values of f and g at the points –1, 0 and 2 be as given in the following table : x=−1x=0x=2f(x)360g(x)01−1 In each of the intervals (-1,0) and (0, 2) the function (f – 3g)'' never vanishes. Then the correct statement(s) is(are)
View Solution
