A man uses a rough inclined plane of length 3 m to raise a load of 100 kgwt. If he does 2400 J of work and the inclined plane offers 300 N resistance, find the mechanical advantage (Take g=10ms−2).
Work done to raise the load = Work done against gravity + work done agianst friction,
w = mg Sinθ×1+f×1
Substituting W = 2400 J, mg = 1000 N,
1=3 m, f = 300 N, we get
2400 J = (1000×sinθ+300)×3
100sinθ+300=800
1000sinθ=500
sinθ=5001000
sinθ=12
M.A. =loadeffort=mgmgsinθ+fs
substitute mg =1000Nsinθ=12.
fs=300N
MA=10001000×12+300=1000800=54=1.25
Alternative method :
Length of the given inclined plane 1 = 3 m (given)
Work done by the effort, We=2400J (given)
Let 'E' be the effort.
∴We=El⇒2400=E(3)⇒E=800N
Mass of the load = 100 kgwt
∴ Load L = (100) (10)
[∵g=10ms−2]
=1000N
∴ Mechanical advantage (M.A.)
=LE=1000800=1.25
Topper's Solved these Questions
- SIMPLE MACHINESBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - SIMPLE MACHINESEXERCISE - Level- 3
10 Videos
- SIMPLE MACHINESBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - SIMPLE MACHINESEXERCISE - Concept Application (Level - 1 )
44 Videos
- MODERN PHYSICSBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - MODERN PHYSICSEXERCISE - Level 3
5 Videos
- WAVES MOTION AND SOUND BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - WAVES MOTION AND SOUND EXERCISE - Level 3
10 Videos
Similar Questions
Knowledge Check
An inclined plane of length 2 m is used to lift a load of 100 kgwt. The force required to lift the load is 20 kgwt. The height through which the load is lifted is ______ m
A2B2.5C1.5D0.4A Sphere pure rolls on a rough inclined plane with initial velocity 2.8 m/s. Find the maximum distance on the inclined plane
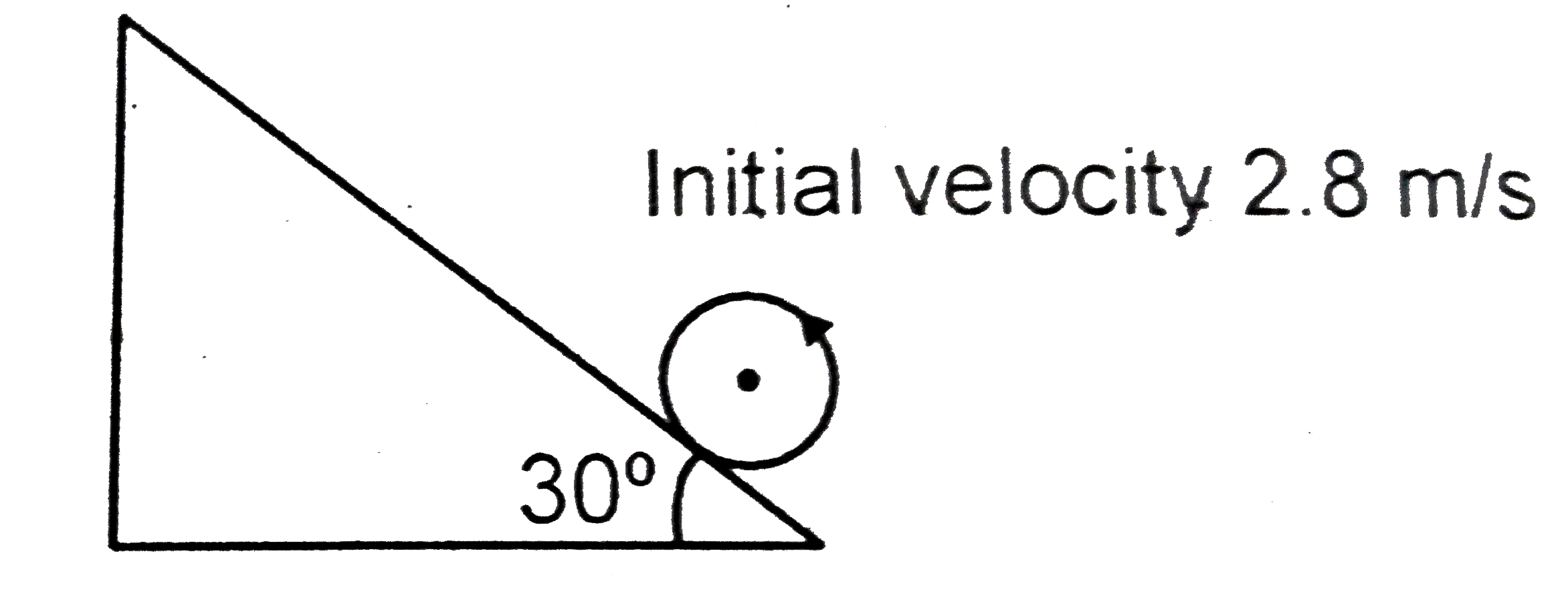 A2.74 mB5.48 mC1.38 mD3.2 m
A2.74 mB5.48 mC1.38 mD3.2 mAn inclined plane of length 2 m is used to lift a load of 100 kgwt. The force required to lift the load is 20 kgwt. The height through which the load is lifted is ______ m
A2B2.5C1.5D0.4
An inclined plane of length 6 cm is used to lift a load of 42 N, by applying an effort of 7 N. Find the height of the plane.
View SolutionIf an inclined plane of length 2 m is used to lift a load of 20 N to a height of 1 m, find the effort required to lift the load to that heiht using inclined plane.
View SolutionIf an inclined plane of length 2 m is used to lift a load of 20 N to a height of 1 m, find the effort required to lift the load to that heiht using inclined plane.

View SolutionA particle of mass 0.2 kg has an intial speed of 5ms−1 at the bottom of a rough inclined plane of inclination 30∘ and vertical height 0.5 m . What is the speed of the particle as it reaches the top of the inclined plan ? (Take μ=1/√3,g=10ms−2 ) .
View SolutionThe efficiency of a rough inclined plane is 90%. The energy spent in raising a load of 225 N through 2 m is
View Solution
