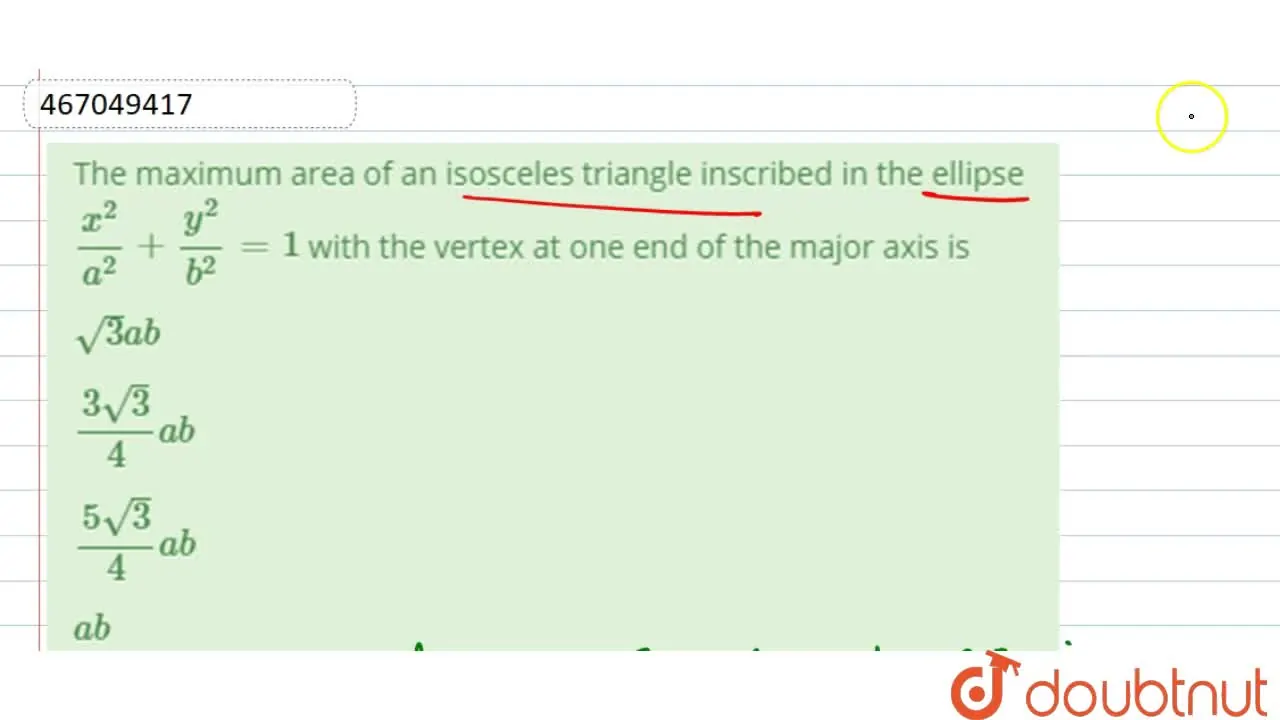
The maximum area of an isosceles triangle inscribed in the ellipse x2a2+y2b2=1 with the vertex at one end of the major axis is Solution in Tamil
√3ab
3√34ab
5√34ab
ab
The correct Answer is:D
Step by step video, text & image solution for The maximum area of an isosceles triangle inscribed in the ellipse (x^2)/(a^2) + (y^2)/(b^2) = 1 with the vertex at one end of the major axis is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - NEW JOYTHI PUBLICATIONCHAPTER - CONIC SECTIONS EXERCISE - EXERCISE - HYPERBOLA9videos
CONIC SECTIONS
- BOOK - NEW JOYTHI PUBLICATIONCHAPTER - CONIC SECTIONS EXERCISE - EXERCISE - PARABOLA19videos
CONIC SECTIONS
- BOOK - NEW JOYTHI PUBLICATIONCHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - QUESTIONS FROM COMPETITIVE EXAMS194videos
COMPLEX NUMBERS AND QUADRATIC EQUATIONS
- BOOK - NEW JOYTHI PUBLICATIONCHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - OBJECTIVE TYPE QUESTION19videos
DIFFERENTIAL EQUATIONS
Similar Questions
The vertices of the hyperbola 9x2−16y2=144
10:45View SolutionThe vertex of the parabola y2+4x=0 is
05:27View SolutionIf p,q are the roots of ax2−25x+c=0, then p3q3+p2q3+p3q2=
04:07View SolutionThe degree of the differential equation [5+(dydx)2]53=x5(d2ydx2) is
06:10View SolutionFind the maximum are of the isosceles triangle inscribed in the ellipse x2a2+y2b2=1, with its vertex at one end of major axis.
07:40View SolutionFind the area of the greatest isosceles triangle that can be inscribed in the ellipse (x2a2)+(y2b2)=1 having its vertex coincident with one extremity of the major axis.
06:51View SolutionA isosceles triangle with each base angle equal to 30^(@) is inscribed in the ellipse (x^(2))/(a^(2))+(y^(2))/(b^(2))=1 If the base of the triangle coincides with the major axis of the ellipse,then find its eccentricity
09:46View SolutionFind the maximum area of an isosceles triangle incribed in the ellipse (x^(2))/(25)+(y^(2))/(16)=1, with its vertex atone end of the major axis.
09:46View SolutionAn isosceles triangle that can be inscribed in an ellipse x2a2+y2b2=1 having its vertex coincident with one extremity of major axis has the maximum area equal to m√n4ab (m,n are prime numbers) then m2−n3=
06:56View Solutionदीर्घवृत x2a2+y2b2=1 के अंतर्गत समद्विबाहु त्रिभुज का महत्तम क्षेत्रफल ज्ञात कीजिए |जिसका शीर्ष दीर्घ अक्ष का एक सिरा है |
02:23View Solutionदीर्घवृत x2a2+y2b2=1 के अंतर्गत उस समद्विबाहु त्रिभुज का महत्तम क्षेत्रफल ज्ञात कीजिए जिसका शीर्ष दीर्घ अक्ष का एक सिरा है।
03:38View Solutionमहत्तम समद्विबाहु त्रिभुज का क्षेत्रफल ज्ञात कीजिए जिसे उस दीर्घवृत में खींचा जा सकता है दीर्घ अक्ष के एक सिरे पर त्रिभुज का शीर्ष है |
06:10View SolutionMaximum area of an isosceles triangle inscribed in the ellipse y2a2+x2b2=1 whose one vertex is at end of major axis
03:21View SolutionThe maximum area in square units of an isosceles triangle inscribed in an ellipse x2a2+y2b2=1 with its vertex at one end of the major axis is :
10:24View SolutionThe maximum area in squre units of an isosceles triangle inscribed in an ellipse x2a2+y2b2=1 with its vertex at one end of the major axis is
03:50View SolutionFind the maximum area of an isosceles triangle inścribed in the ellipse x225+y216==1, with its vertex atone end of the major axis.
06:53View Solution