A point source of light is kept on the surface of a sphere and it is found that parallel light rays emerge from the other side of the sphere. What is refractive index of material of the sphere ?
1.5
2
2.5
3
The correct Answer is:B
To find the refractive index of the material of the sphere given that parallel light rays emerge from the other side, we can follow these steps:
1. Understanding the Situation:
- We have a point source of light on the surface of a sphere.
- Light rays from this source are refracted at the surface of the sphere and emerge as parallel rays on the other side.
2. Identifying the Image Formation:
- Since the rays emerge as parallel, we can conclude that the image of the point source is formed at infinity.
3. Applying the Lens Maker's Formula:
- We can use the formula for refraction at a spherical surface:
n2v−n1u=n2−n1R
- Where:
- n1 is the refractive index of the material of the sphere (let's denote it as n).
- n2 is the refractive index of air, which is 1.
- u is the object distance (the distance from the point source to the surface of the sphere, which is −R since it's on the surface).
- v is the image distance (which is at infinity, so v=∞).
- R is the radius of the sphere.
4. Substituting Values:
- Substitute n1=n, n2=1, u=−R, and v=∞ into the formula:
1∞−n−R=1−nR
- Since 1∞=0, the equation simplifies to:
nR=1−nR
5. Simplifying the Equation:
- Multiply through by R (assuming R≠0):
n=1−n
- Rearranging gives:
n+n=1
2n=1
n=12
6. Conclusion:
- The refractive index of the material of the sphere is n=2.
Final Answer:
The refractive index of the material of the sphere is 2.
- We have a point source of light on the surface of a sphere.
- Light rays from this source are refracted at the surface of the sphere and emerge as parallel rays on the other side.
- Since the rays emerge as parallel, we can conclude that the image of the point source is formed at infinity.
- We can use the formula for refraction at a spherical surface:
n2v−n1u=n2−n1R
- Where:
- n1 is the refractive index of the material of the sphere (let's denote it as n).
- n2 is the refractive index of air, which is 1.
- u is the object distance (the distance from the point source to the surface of the sphere, which is −R since it's on the surface).
- v is the image distance (which is at infinity, so v=∞).
- R is the radius of the sphere.
- Substitute n1=n, n2=1, u=−R, and v=∞ into the formula:
1∞−n−R=1−nR
- Since 1∞=0, the equation simplifies to:
nR=1−nR
- Multiply through by R (assuming R≠0):
n=1−n
- Rearranging gives:
n+n=1
2n=1
n=12
- The refractive index of the material of the sphere is n=2.
The refractive index of the material of the sphere is 2.
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - OBJECTIVE TYPE QUESTIONS (B. MULTIPLE CHOICE QUESTIONS)
21 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - OBJECTIVE TYPE QUESTIONS (JEE (MAIN) & OTHER STATE BOARDS FOR ENGINEERING ENTRANCE)
40 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - Revision Exercises (Numerical Problems)
36 Videos
- NUCLEIBOOK - MODERN PUBLICATIONCHAPTER - NUCLEIEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
15 Videos
- SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS BOOK - MODERN PUBLICATIONCHAPTER - SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS EXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
12 Videos
Similar Questions
A ray of light falls on a transparent sphere with centre at C as shown in figure. The ray emerges from the sphere parallel to line AB. The refractive index of the sphere is
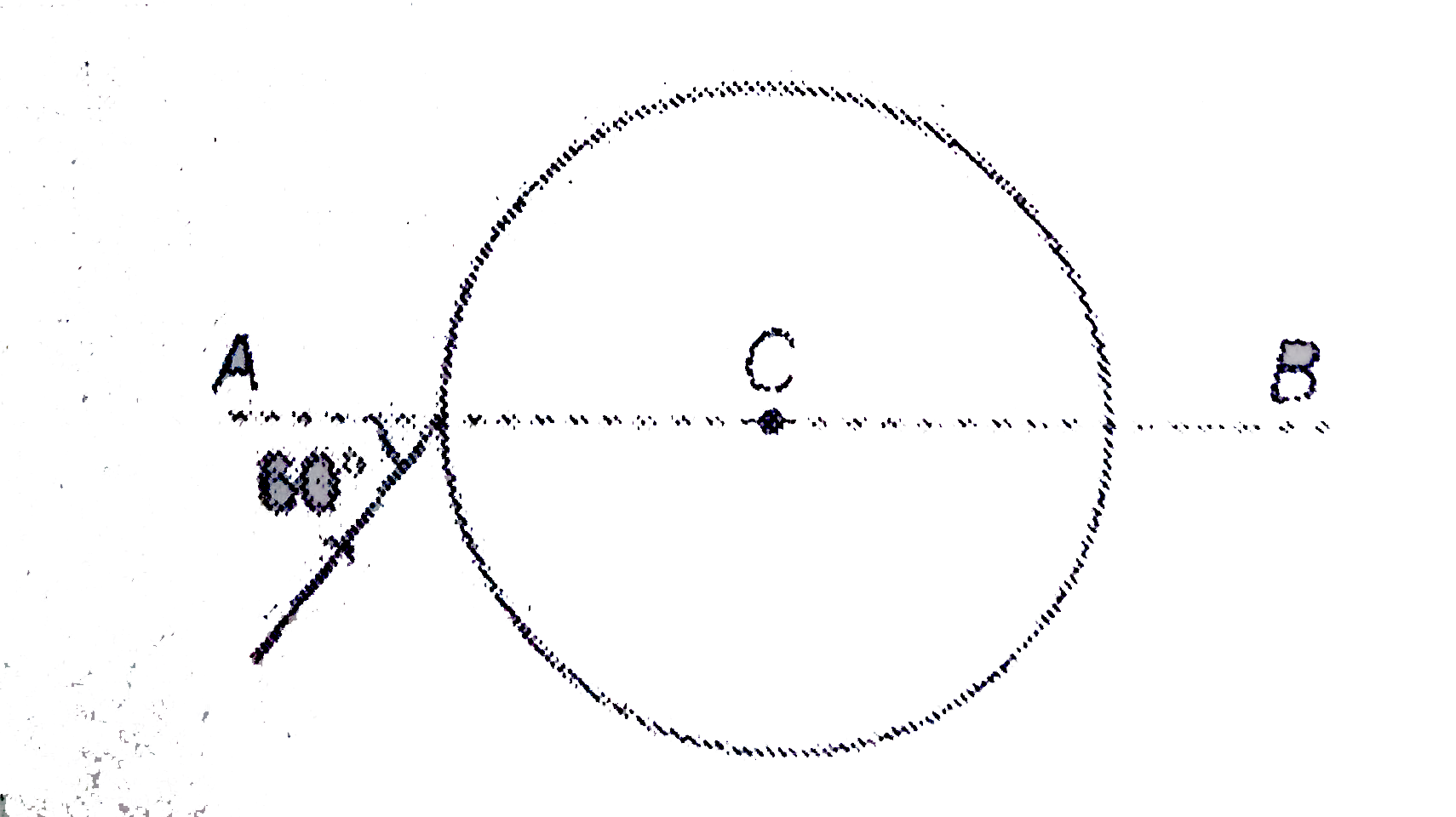
View SolutionA ray of light falls on a transparent sphere with centre at C as shown in figure. The ray emerges from the sphere parallel to line AB. The refractive index of the sphere is

View Solution
Knowledge Check
A poing source of light at the surface of a sphere causes a paralel beam of light to emerge from the opposite surface of the sphere. The refractive index of the material of the sphere is
A1.5B5/3C2D2.5A parallel beam of light emerges from the opposite surface of the sphere when a point source of light lies at the surface of the sphere. The refractive index of the sphere is
A32B53C2D52A parallel beam of light emerges from the opposite surface of the sphere when a point source of light lies at the surface of the sphere. The refractive index of the sphere is
A32B53C2D52
A ray of light is incident at an angle of 60∘ on one face of a prism of angle 30∘. They ray emerges normally from the other face of the prism. The refractive index of the prism material is
View SolutionParallel rays are incident on a transparent sphere along its one diameter. After refraction, these rays converge at the other end of this diameter. The refractive index for the material of sphere is
View SolutionA monochromatic ray of light is incident normally on one of the refracting surfaces of a prism . The emergent ray grazes the second refracting surface . What is the angle of the prism if the refractive index of the material of the prism is 1.6 ?
View SolutionA ray of light is incident normally on one of the refracting surfaces of a prism of refracting angle A,. The emergent ray grazes the other refracting surface. Find the refractive index of the material ofprism.
View SolutionThe angles of refraction of a very thin prism is 1∘. A light say is incident normally on one of the refracting surface. The ray that ultimately emerges from the first surface, after suffering from the second surface. Makes an angle of 3.32∘ with the normal. the deviation of the ray emerging from the second surface and the refractive index of the material of the prism respectivley, are
View Solution
