A concave lens and a plane mirror re placed coaxially 10 cm apart. A point object is placed at a distance of 40 cm from the concave lens. The final image formed by the combination is located 30 cm behind the mirror. Calculate the focal length of the concave lens.
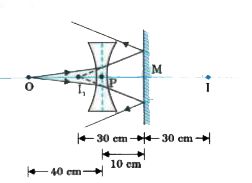
From figure. For the concave lens,
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - PRACTICE PROBLEMS (1)
13 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - PRACTICE PROBLEMS (2)
9 Videos
- NUCLEIBOOK - MODERN PUBLICATIONCHAPTER - NUCLEIEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
15 Videos
- SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS BOOK - MODERN PUBLICATIONCHAPTER - SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS EXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
12 Videos
Similar Questions
An object is placed at a distance of 4 cm from a concave lens of focal length 12 cm. Find the position and nature of the image.
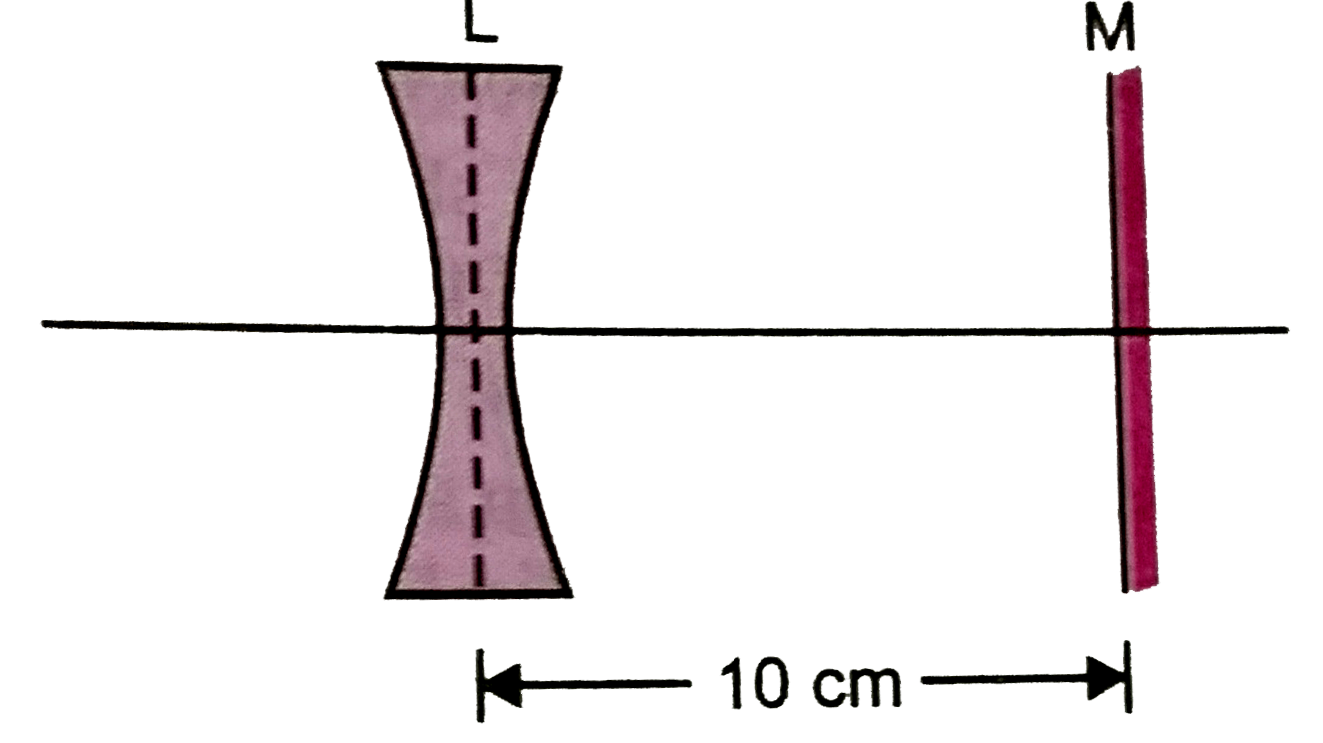
View SolutionFig. shows a plane mirror M placed at a distance of 10cm from a concave lens L. A point object is placed at a distance of 60cm from the lens. The image formed due to refraction by the lens and reflection by the mirror is 30cm behind th mirror. What is the focal length of this lens ?
 .
.
View Solution
Knowledge Check
An object is placed at a distance of 30 cm from a concave mirror and its real image is formed at a distance of 30 cm from the mirror. The focal length of the mirror is
A15 cmB45 cmC30 cmD20 cmA convex lens of focal length 30 cm , a concave lens of focal length 120 cm , and a plane mirror are arranged as shown . For an object kept at a distance of 60 cm from the convex lens, the final image, formed by the combination , is a real image , at a distance of :
_E01_195_Q01.png)
|Focal length|=|Focal length|
= 30cm =120cm
_S01_267_Q02.png) A70 cm from the convex lensB60 cm from the convex lensC60 cm from the concave lensD70 cm from the concave lens
A70 cm from the convex lensB60 cm from the convex lensC60 cm from the concave lensD70 cm from the concave lensAn object is placed 40 cm from a concave mirror of focal length 20 cm. The image formed is
AReal, inverted and same in sizeBReal, inverted and smallerCVirtual, erect and largerDVirtual, erect and smaller
An object is placed at a distance of 30cm from a concave lens of focal length 15cm. Find the nature and position of the image.
View SolutionAn object is placed at a distance of 50cm from a concave lens of focal length 20cm. Find the nature and position of the image.
View SolutionAn object is placed at a distance of 40 cm in front of a concave mirror of focal length 20 cm. The image produced is
View SolutionAn object is placed at a distance of 15 cm from a concave mirror and its real image is formed at 30 cm in front of that mirror. The focal length of the mirror is
View SolutionAn object is placed at a distance of 40 cm in front of a concave mirror of focal length 20 cm the image produced is
View Solution
