In AM wave, carrier power is given by :
Pc=(2E)2CR
Pc=(E)2CR
Pc=(E)2C2R
Pc=(E)2C√2(R)
The correct Answer is:C
To find the carrier power (PC) in an Amplitude Modulated (AM) wave, we can follow these steps:
Step 1: Understand the formula for carrier power
The carrier power (PC) in an AM wave is given by the formula:
PC=E2RMSR
where:
- ERMS is the root mean square (RMS) value of the carrier signal.
- R is the resistance across which the power is measured.
Step 2: Relate RMS value to peak value
The RMS value of the carrier signal can be related to its peak value (EC) using the formula:
ERMS=EC√2
where:
- EC is the peak value of the carrier signal.
Step 3: Substitute the RMS value into the power formula
Substituting the expression for ERMS into the carrier power formula, we get:
PC=(EC√2)2R
Step 4: Simplify the expression
Now, simplify the expression:
PC=E2C2R
Step 5: Final expression for carrier power
Thus, the final expression for the carrier power in an AM wave is:
PC=E2C2R
Step 6: Identify the correct option
Now, we can check which option matches this expression. The correct answer is:
PC=E2C2R
This corresponds to option C.
Summary
The carrier power in an AM wave is given by:
PC=E2C2R
---
The carrier power (PC) in an AM wave is given by the formula:
PC=E2RMSR
where:
- ERMS is the root mean square (RMS) value of the carrier signal.
- R is the resistance across which the power is measured.
The RMS value of the carrier signal can be related to its peak value (EC) using the formula:
ERMS=EC√2
where:
- EC is the peak value of the carrier signal.
Substituting the expression for ERMS into the carrier power formula, we get:
PC=(EC√2)2R
Now, simplify the expression:
PC=E2C2R
Thus, the final expression for the carrier power in an AM wave is:
PC=E2C2R
Now, we can check which option matches this expression. The correct answer is:
PC=E2C2R
This corresponds to option C.
The carrier power in an AM wave is given by:
PC=E2C2R
Topper's Solved these Questions
- SEMICONDUCTOR AND DEVICEBook:FIITJEEChapter:SEMICONDUCTOR AND DEVICEExercise:ASSIGNMENT PROBLEMS(Subjective: Level-I)
Explore 40 Videos
- SEMICONDUCTOR AND DEVICEBook:FIITJEEChapter:SEMICONDUCTOR AND DEVICEExercise:ASSIGNMENT PROBLEMS( objective : Level-I)
Explore 41 Videos
- SEMICONDUCTOR AND DEVICEBook:FIITJEEChapter:SEMICONDUCTOR AND DEVICEExercise:SOLVED PROBLEMS (SUBJECTIVE)
Explore 30 Videos
- ROTATIONAL MECHANICSBook:FIITJEEChapter:ROTATIONAL MECHANICSExercise:COMPREHENSION - IV
Explore 2 Videos
- SIMPLE HARMONIC MOTIONBook:FIITJEEChapter:SIMPLE HARMONIC MOTIONExercise:Assignment Problems (Objective) (Level-II)
Explore 20 Videos
Similar Questions
A modulating signal is a square wave is shown in figure. The carrier wave is given by C(t)=2sin(8πt) volt.
(i) Sketch the amplitude modulated wave form.
(ii) What is the modulating index?

View SolutionThe carrier wave of a signal is given by C(t) = 3sin(8πt)volt.
The modulating signal is a square wave as shown. Find its modulation index.
View Solution
Knowledge Check
A modulating signal is a square wave as shown in the figure
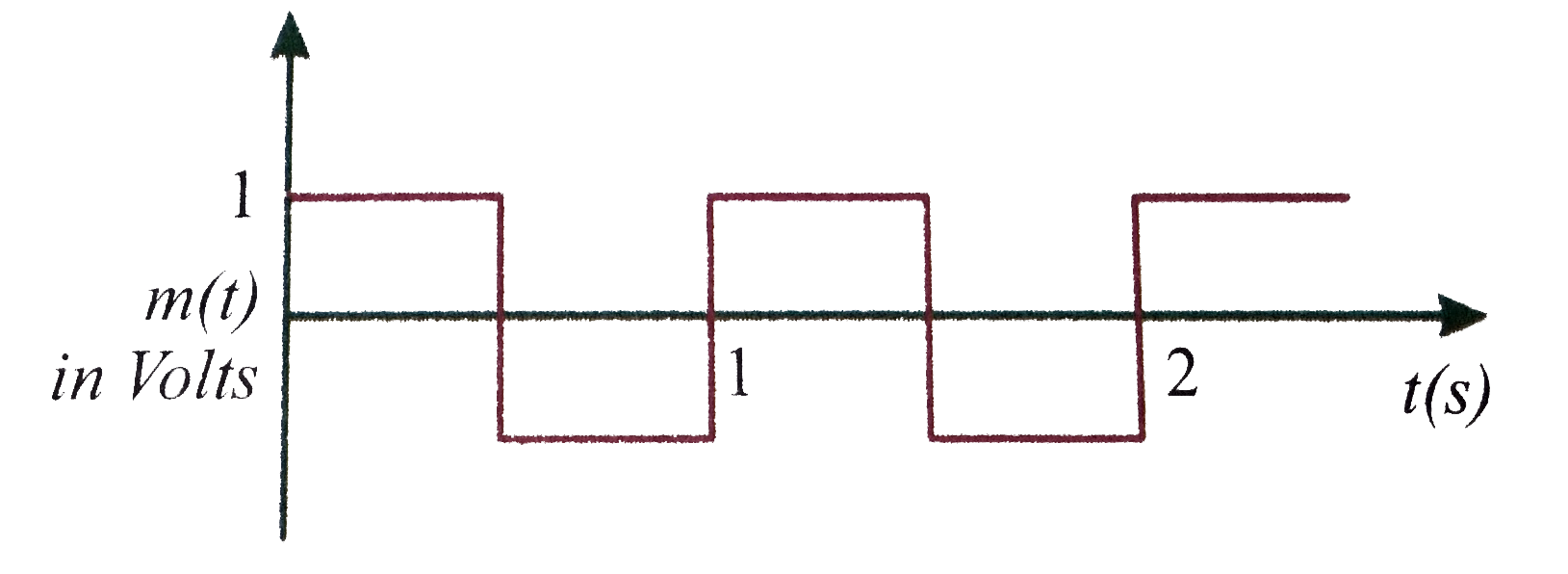
The carrier wave is given by.
c(t)=1.33sin(8πt)volts
The modulation index isA2B0.75C0.5D1.5A modulating signal is a square wave as shown in figure.
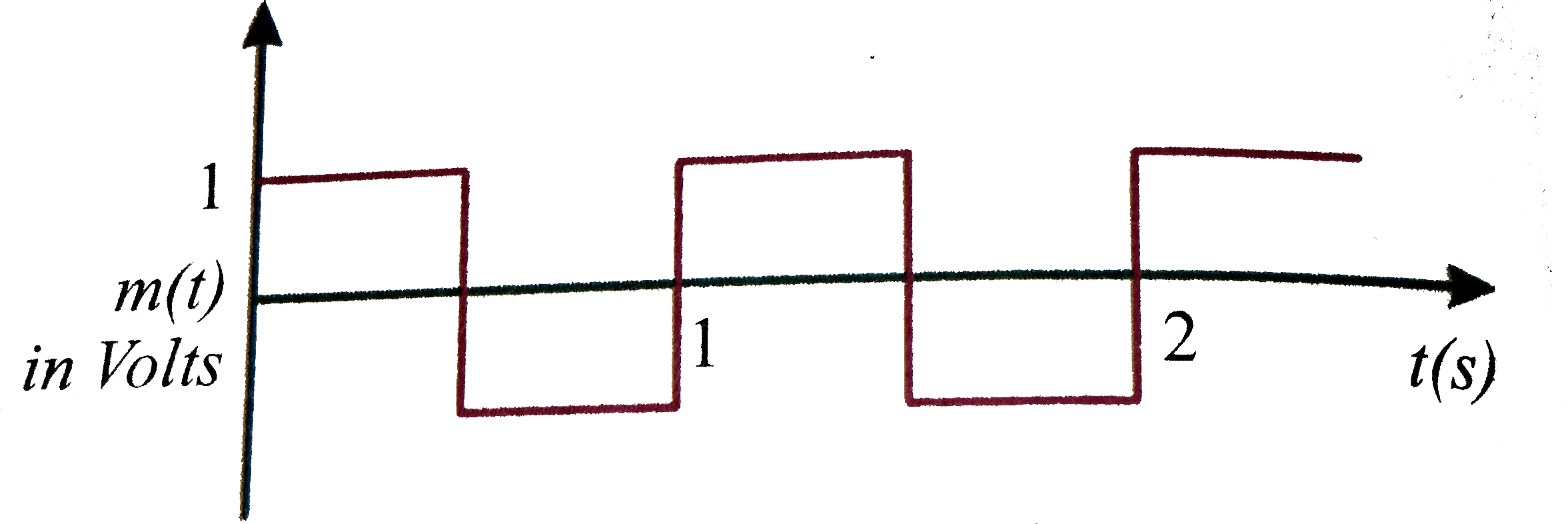
The carrier wave is given by
c(t)=2sin(8πt)volt.
The modulation index isA2B0.75C0.5D1.5In AM wave total power of side bands is given by :
APS=E2C4RBPs=4E2CRCPs=m2E2c4RDPs=E2c4m2R
Power Of Wave
View SolutionA separate high freq. wave (i.e. carrier wave) is needed in modulation why ?
View SolutionA Broadcast AM transmitter radiates 50 kW of carrier power. What will be the radiated power at 85% modulation ?
View SolutionIn AM wave total power of side bands is given by :
View SolutionIf carrier wave is given by yc=Acsin(ωct) and message signal is ys=Assin(ωst) find bandwidth of AM wave (in hz)
View Solution