When a 'j' shaped conducting rod is rotating in its own plane with constant angular velocity ω about one of its ends P in a uniform magnetic field →B ( directed normally into the plane of paper ) the magnitude of emf induced across it will be

Bω√L2+l2
12BωlL2
12Bω(L2+l2)
12Bωl2
The correct Answer is:C
Step by step video, text & image solution for When a 'j' shaped conducting rod is rotating in its own plane with constant angular velocity omega about one of its ends P in a uniform magnetic field rarr B ( directed normally into the plane of paper ) the magnitude of emf induced across it will be by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - FIITJEECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSIGNMENT PROBLEMS (OBJECTIVE ) LEVEL -I
1 Video
- ELECTROMAGNETIC INDUCTIONBOOK - FIITJEECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSIGNMENT PROBLEMS (ASSERTION REASONING TYPE)
2 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - FIITJEECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSIGNMENT PROBLEMS ( SUBJECTIVE ) LEVEL - II & III
15 Videos
- ELASTICITY AND WAVES BOOK - FIITJEECHAPTER - ELASTICITY AND WAVES EXERCISE - Assignment Problems (Objective) Level-II
15 Videos
- ELECTROMAGNETIC INDUCTION AND AC CURRENTBOOK - FIITJEECHAPTER - ELECTROMAGNETIC INDUCTION AND AC CURRENTEXERCISE - Example
15 Videos
Similar Questions
A conducting rod of length l is rotating with constant angular velocity ω about point O in a uniform magnetic field B as shown in the figure . What is the emf induced between ends P and Q ?

View SolutionA conducting disc of radius r rotates with a small but constant angular velocity ω about its axis. A uniform magnetic field B exists parallel to the axis of rotation. Find the motional emf between the centre and the periphery of the disc.
View Solution
Knowledge Check
When a 'J' shaped conducting rod rotating in its own plane with constant angular velocity omega, avout one of its end P, in a uniform magnetic field →B directed normally into the plane of paper) then magnetic of emf induced across it will be
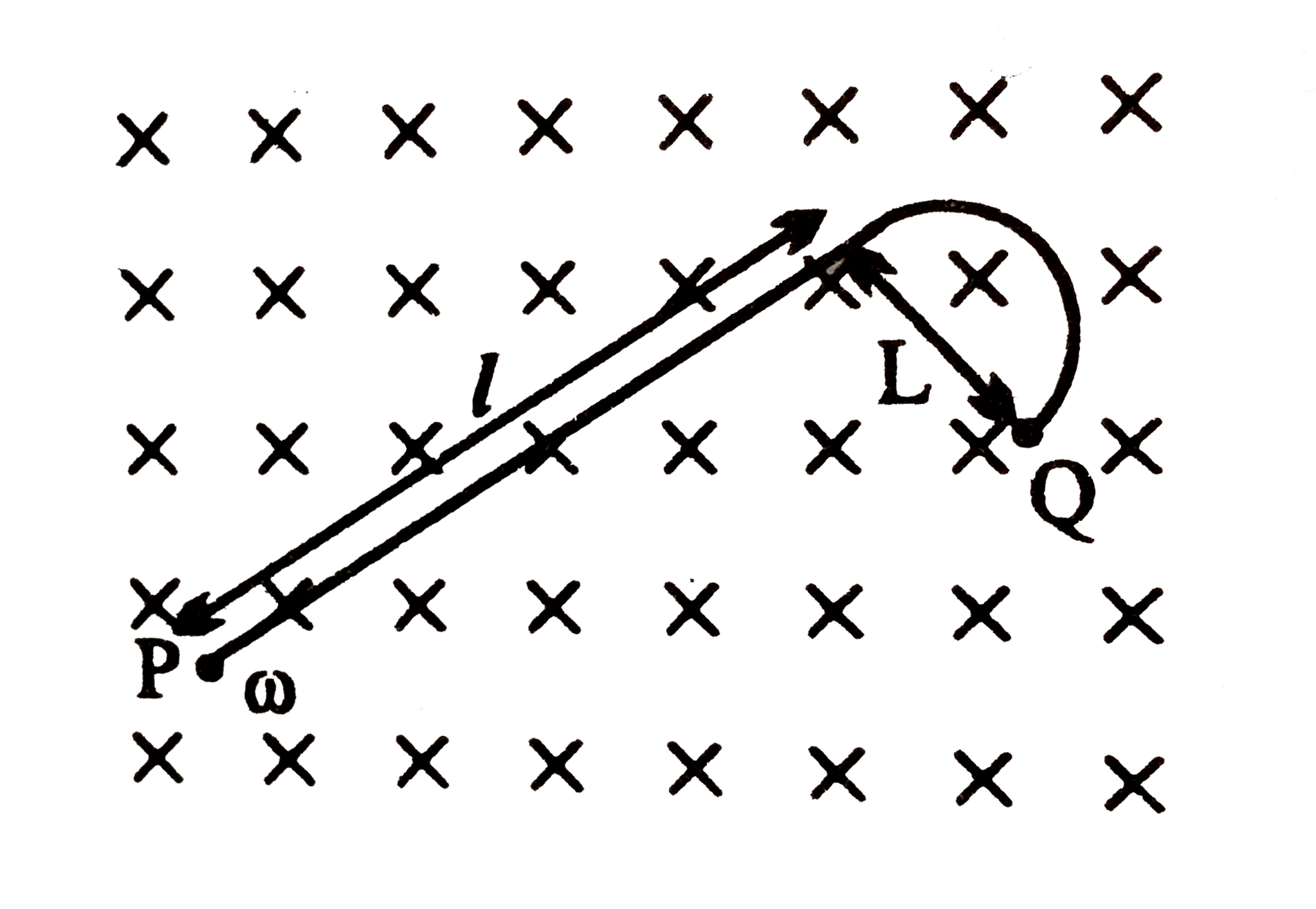 ABω√L2+l2B12BωL2C1Bω(L2+l2)D12Bωl2
ABω√L2+l2B12BωL2C1Bω(L2+l2)D12Bωl2A conducting loop rotates with constant angular velocity about its fixed diameter in a uniform magnetic field, whose direction is perpendicular to that fixed diameter.
A(a) The emf will be maximum at the moment when flux is zeroB(b) The emf will be 0 at the moment when flux ismaximumC( c) The emf will be maximum at the moment when plane of the loop is parallel to the magnetic fieldD(d) The phase difference between the flux and the emf π/2A non-conducting disk of radius R is rotating about its own axis with constant angular velocity ω in a perpendicular uniform magnetic field B as shown in figure. The emf induced between centre and rim of disk is
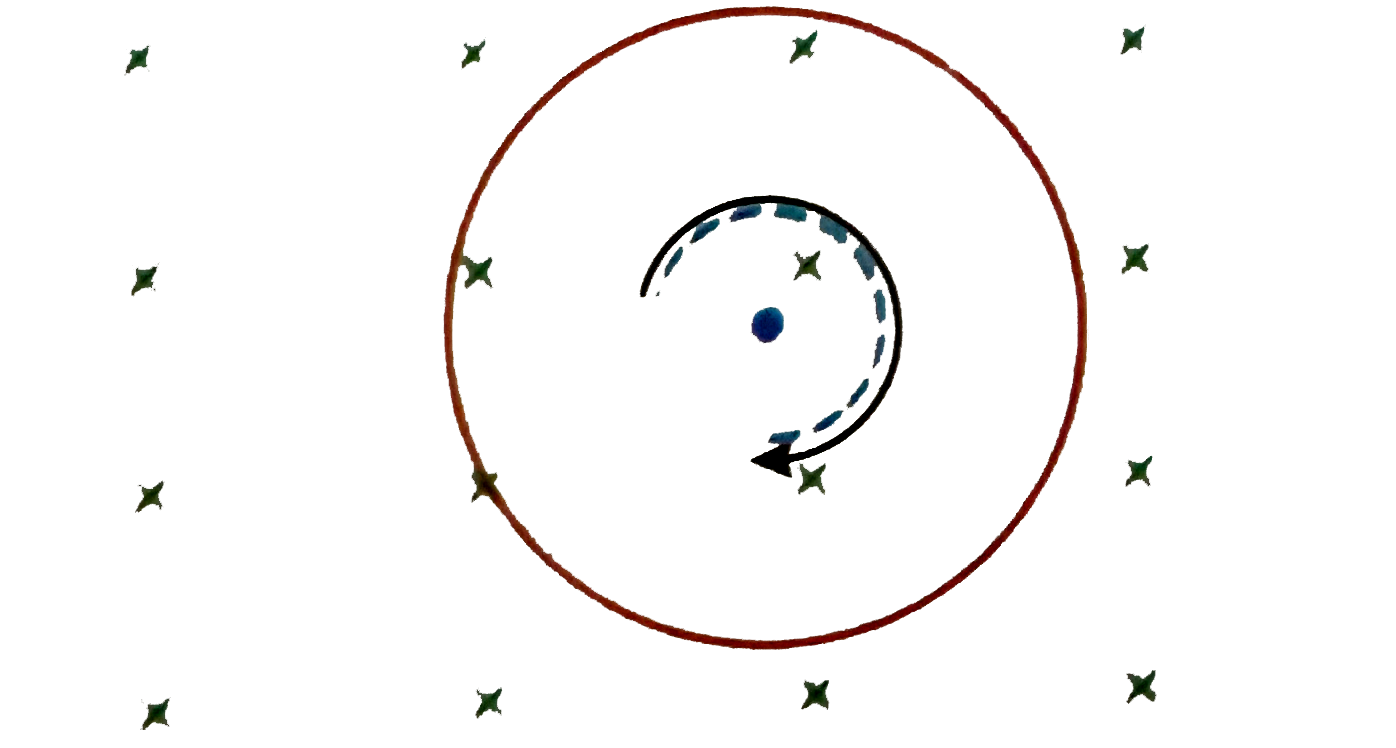 ABωR22BBωR2CZeroDBωR23
ABωR22BBωR2CZeroDBωR23
A conducting loop confined in a plane is rotated in its own plane with some angular velocity.A uniform and constant magnetic field exist in the region.Find the current induced in the loop.
View SolutionFor an L shaped conducting rod placed in an uniform magnetic field →B rotating with constant angular velocity ω about an axis passing through one of its ends A and normal to the plane of the conductor induced emf
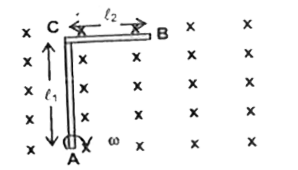
View SolutionA conducting ring of radius 'r' is rolling without slipping with a constant angular velocity ω (figure). If the magnetic field strengh is B and is directed into the page the emf induced across PQ is
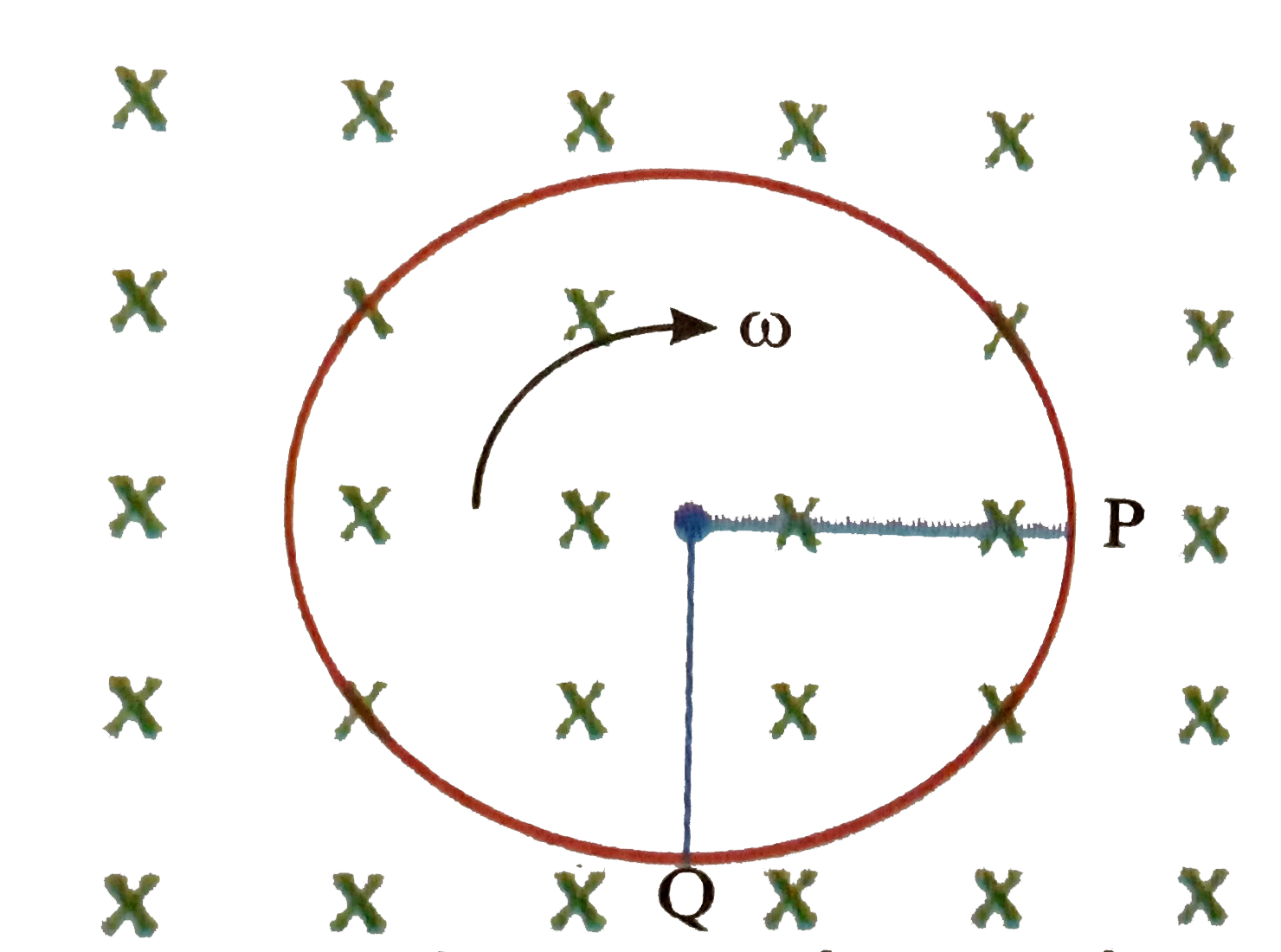
View SolutionA coil of N turns and mean area A is rotating with uniform angular velocity ω about an axis ai right angles to a uniform magnetic field B. The induced emfe in the coil will be
View SolutionA conducting rod of length 2l is rotating with constant angular speed ω about is perpendicular bisector. A uniform magnetic field →B exists parallel to the axis of rotation. The e.m.f. induced between two ends of the rod is
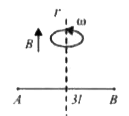
View Solution
