A particle dropped from a height of 100 m, collides with the horizontal surface where coefficient of restitution is 0.5, the velocity attained by the particle in 5th collision is
4.2 m/s
6.2 m/s
8.4 m/s
6.4 m/s
The correct Answer is:A
To solve the problem step by step, we will follow the outlined approach in the video transcript.
Step 1: Calculate the initial velocity just before the first collision
The particle is dropped from a height h=100m. We can use the third equation of motion to find the velocity just before the collision:
v=√u2+2gh
Where:
- u=0 (initial velocity, since the particle is dropped),
- g=10m/s2 (acceleration due to gravity),
- h=100m (height).
Substituting the values:
v=√0+2×10×100=√2000=44.72m/s
Step 2: Calculate the velocity after the first collision
The coefficient of restitution e=0.5. The velocity after the first collision is given by:
v1=e⋅v
Substituting the values:
v1=0.5×44.72=22.36m/s
Step 3: Calculate the velocity after subsequent collisions
The velocity after each collision can be expressed as:
- After the second collision: v2=e⋅v1=e2⋅v
- After the third collision: v3=e⋅v2=e3⋅v
- After the fourth collision: v4=e⋅v3=e4⋅v
- After the fifth collision: v5=e⋅v4=e5⋅v
Thus, the velocity after the fifth collision is:
v5=e5⋅v
Step 4: Substitute the values to find the velocity after the fifth collision
Now substituting the values we have:
v5=(0.5)5⋅44.72
Calculating (0.5)5=132=0.03125:
v5=0.03125⋅44.72≈1.4m/s
Final Answer
The velocity attained by the particle in the 5th collision is approximately 1.4m/s.
---
The particle is dropped from a height h=100m. We can use the third equation of motion to find the velocity just before the collision:
- u=0 (initial velocity, since the particle is dropped),
- g=10m/s2 (acceleration due to gravity),
- h=100m (height).
The coefficient of restitution e=0.5. The velocity after the first collision is given by:
The velocity after each collision can be expressed as:
- After the third collision: v3=e⋅v2=e3⋅v
- After the fourth collision: v4=e⋅v3=e4⋅v
- After the fifth collision: v5=e⋅v4=e5⋅v
Now substituting the values we have:
The velocity attained by the particle in the 5th collision is approximately 1.4m/s.
Topper's Solved these Questions
- COLLISION Book:FIITJEEChapter:COLLISION Exercise:ASSIGNMENT PROBLEMS (OBJECTIVE) Level - I (ASSERTION REASONING TYPE)
Explore 2 Videos
- COLLISION Book:FIITJEEChapter:COLLISION Exercise:ASSIGNMENT PROBLEMS (OBJECTIVE) Level - II
Explore 20 Videos
- COLLISION Book:FIITJEEChapter:COLLISION Exercise:ASSIGNMENT PROBLEMS (SUBJECTIVE) Level - II
Explore 17 Videos
- AC CIRCUITSBook:FIITJEEChapter:AC CIRCUITSExercise:ASSERTION REASONING TYPE
Explore 1 Video
- CURRENT ELECTRICITYBook:FIITJEEChapter:CURRENT ELECTRICITYExercise:Comprehension -4
Explore 3 Videos
Similar Questions
A ball is dropped from a height of 3 m. What is the height upto which the ball will rebound ? The cofficient of restitution is 0.5.
View SolutionA ball falls from a height of 5m on to a horizontal plane.If the coefficient of restitution is 0.4, the height to which it rebounds after 2 collisions is approximately
View Solution
Knowledge Check
A ball falls from a height of 10m on to a horizontal plane. If the coefficient of restitution is 0.4, then the velocity with which it rebounds from the plane after second collision is
A2.24ms−1B5.6ms−1C2.8ms−1D0.9ms−1A ball is dropped from height 'H' onto a horizontal surface. If the coefficient of restitution is 'e' then the total time after which it comes to rest is
A√2Hg(1−e1+e)B√2Hg(1+e1−e)C√2Hg(1+e21−e2)D√2Hg(1−e21+e2)A ball is dropped from a height h on a horizontal plane and the coefficient of restitution for the impact is e, the velocity with which the ball rebounds from the flor is
AehBeghCe√(gh)De√(2gh)
A particle is dropped from rest from a height h0 on a horizontal floor. The coefficient of restitution between the floor and particle is e. Maximum height attained by the particle after first rebound is
View SolutionA ball is dropped freely from a height of 20m on to a hard fiat surface. The coefficient of restitution between the ball and the surface is 0.5. Find the time (in second) it takes to strike the surface for the second time.
View SolutionA glass sphere of mass 5mg, falls from a height of 3 meters on to a horizontal surface. If the coefficient of restitution is 0.5, then after the impact the sphere will rise to a height of
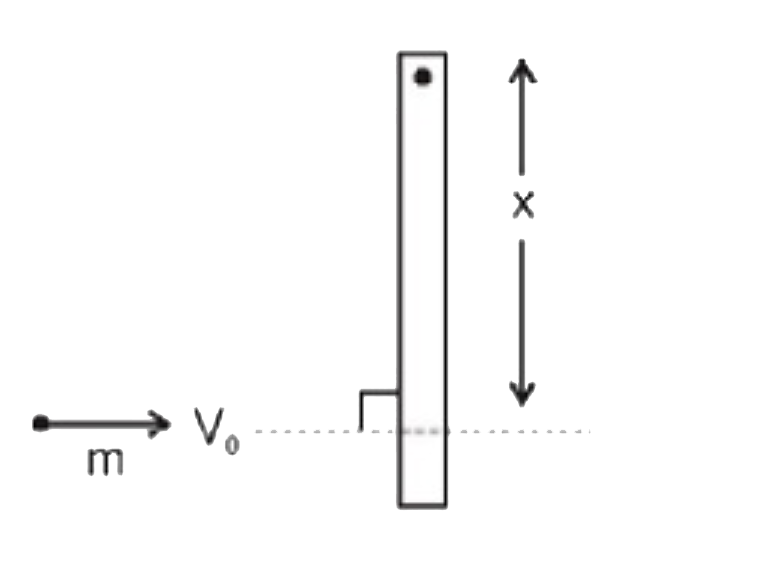
View SolutionA uniform rod of mass m and length L is hinged at one end and free to rotate in the horizontal plane. All the surface are smooth. A particle of same mass m collides with the rod with a speed V0. The coefficient of restitution for the collision is e=12. If hinge reaction during the collision is zero then the value of x is :
View SolutionA particle falls from a height h on a fixed horizontal plate and rebounds. If e is the coefficient of restitution, the total distance travelled by the particle before it stops rebounding is
View Solution