A bullet of mass 0.04 kg moving with a speed of 600 m/s penetrates a heavy wooden block. It stops after a penetration of 500 cm. The resistive force exerted by the block on the bullet is
1440 N
1480 N
270 N
310 N
The correct Answer is:A
To solve the problem step by step, we will use the work-energy theorem, which states that the work done on an object is equal to the change in its kinetic energy.
Step 1: Identify the given data
- Mass of the bullet, m=0.04kg
- Initial speed of the bullet, vi=600m/s
- Final speed of the bullet, vf=0m/s (since the bullet stops)
- Penetration distance, d=500cm=5m
Step 2: Calculate the initial kinetic energy of the bullet
The initial kinetic energy (KEi) can be calculated using the formula:
KEi=12mv2i
Substituting the values:
KEi=12×0.04kg×(600m/s)2
KEi=12×0.04×360000
KEi=0.02×360000=7200J
Step 3: Calculate the final kinetic energy of the bullet
The final kinetic energy (KEf) is zero since the bullet comes to a stop:
KEf=0J
Step 4: Apply the work-energy theorem
According to the work-energy theorem:
KEf−KEi=Work done by the resistive force
Substituting the values:
0−7200=Work done by the resistive force
Work done by the resistive force=−7200J
Step 5: Relate work done to the resistive force
The work done by the resistive force can also be expressed as:
Work=F⋅d
Where F is the resistive force and d is the distance penetrated. Rearranging gives:
F=Workd
Substituting the values:
F=−7200J5m
F=−1440N
Step 6: Determine the magnitude of the resistive force
The magnitude of the resistive force is:
|F|=1440N
Final Answer
The resistive force exerted by the block on the bullet is 1440 N.
- Mass of the bullet, m=0.04kg
- Initial speed of the bullet, vi=600m/s
- Final speed of the bullet, vf=0m/s (since the bullet stops)
- Penetration distance, d=500cm=5m
The initial kinetic energy (KEi) can be calculated using the formula:
KEi=12mv2i
Substituting the values:
KEi=12×0.04kg×(600m/s)2
KEi=12×0.04×360000
KEi=0.02×360000=7200J
The final kinetic energy (KEf) is zero since the bullet comes to a stop:
KEf=0J
According to the work-energy theorem:
KEf−KEi=Work done by the resistive force
Substituting the values:
0−7200=Work done by the resistive force
Work done by the resistive force=−7200J
The work done by the resistive force can also be expressed as:
Work=F⋅d
Where F is the resistive force and d is the distance penetrated. Rearranging gives:
F=Workd
Substituting the values:
F=−7200J5m
F=−1440N
The magnitude of the resistive force is:
|F|=1440N
The resistive force exerted by the block on the bullet is 1440 N.
Topper's Solved these Questions
- COLLISION BOOK - FIITJEECHAPTER - COLLISION EXERCISE - ASSIGNMENT PROBLEMS (OBJECTIVE) Level - I (ASSERTION REASONING TYPE)
2 Videos
- COLLISION BOOK - FIITJEECHAPTER - COLLISION EXERCISE - ASSIGNMENT PROBLEMS (OBJECTIVE) Level - II
20 Videos
- COLLISION BOOK - FIITJEECHAPTER - COLLISION EXERCISE - ASSIGNMENT PROBLEMS (SUBJECTIVE) Level - II
17 Videos
- AC CIRCUITSBOOK - FIITJEECHAPTER - AC CIRCUITSEXERCISE - ASSERTION REASONING TYPE
1 Video
- CURRENT ELECTRICITYBOOK - FIITJEECHAPTER - CURRENT ELECTRICITYEXERCISE - Comprehension -4
3 Videos
Similar Questions
A bullet of mass 0.04 kg moving with a speed of 90ms−1 enters a heavy wooden block and is stooped after a distance of 60 cm . What is the average resistive force exerted by the block on the bullet ?
View SolutionA bullet of mass 0.06 kg moving with a speed of 90ms−1 enters a heavy wooden block and it stopped after a distance of 60 cm. What is the average resistive force exerted by the block on the bullet ?
View Solution
Knowledge Check
A bullet of mass 0.05 kg moving with a speed of 80m/s enters a wooden block and is stopped after a distance of 0.40 m The average resistive force exerted by the block on the bullet is:
A300 NB20 NC400 ND40 NA bullet of mass 40g moving with a speed of 90ms−1 enters a heavy wooden block and is stopped after a direction of 60cm. The average resistive force exerted by the block on the bullet is
A180NB220NC270ND320N
A bullet of mass 20 g moving with a speed of 120 m/s hits a thick muddy wall and penetrates into it. It takes 0.03 s to stop in the wall find the force exerted by the bullet on the wall,
View SolutionA bullet of mass 0.03 kg moving with a speed of 400m/s penerates 12 cm into fixed block of wood. Calculate the average force exerted by the wood on the bullet.
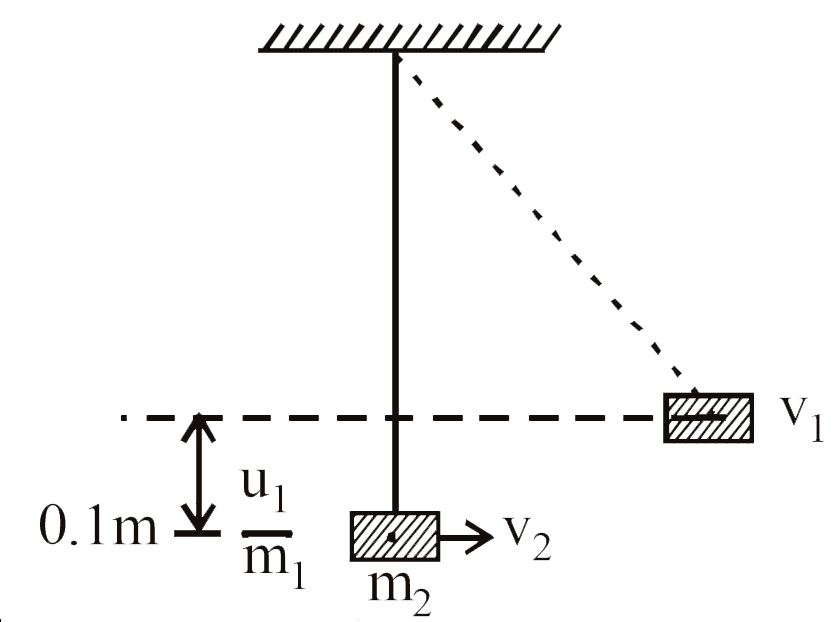
View SolutionA bullet of mass 0.01 kg travelling at a speed of 500 m/s strikes a block of mass 2 kg, which is suspended by a string of length 5 m. The centre of gravity of the block is found to rise a vertical distance of 0.1 m. The speed of the bullet after it emerges from the block will be -
View SolutionA 10 g bullet moving at 200 m/s stops after penetrating 5 cm of wooden plank. The average force exerted on the bullet will be
View SolutionA bullet of mass 100gram moving with 20m/s Strikes a wooden plank and penetrates upto 20 cm. Calculate the resistance offered by the wooden plank .
View SolutionA bullet of mass 5 g travels with a speed of 500m/s. If it penetrates a fixed target which offers a constant resistive force of 1000N to the motion of the bullet, find (a) the initial kinetic energy of the bullet (b) the distance through which the bullet has penetrated.
View Solution
