A shop keeper sells three varieties of perfumes and he has a large number of bottles of the same size of each variety in this stock. There are 5 places in a row in his show case. The number of different ways of displaying the three varieties of perfumes in the show case is
A. 250
B. 125
C. 150
D. 300
The correct Answer is:B
To find the number of different ways to display three varieties of perfumes in five places in a showcase, we can follow these steps:
Step 1: Understand the Problem
We have three varieties of perfumes (let's call them A, B, and C) and five places in a row to display them. Since there is a large number of bottles of each variety, we can use any variety in any place.
Step 2: Calculate the Total Combinations
Since there are no restrictions on how we can fill the places, each of the 5 places can be filled with any of the 3 varieties. Therefore, for each place, we have 3 choices.
The total number of ways to fill the 5 places is given by:
Total Ways=35
Step 3: Calculate 35
Now we calculate 35:
35=3×3×3×3×3=243
Step 4: Consider Restrictions
Next, we consider the restrictions mentioned in the video transcript. We need to subtract the cases where all places are filled with only one variety and where only two varieties are used.
Case 1: All Places with One Variety
If all 5 places are filled with the same variety, we can choose any of the 3 varieties. Thus, there are:
3 ways (A, B, or C)
Case 2: All Places with Two Different Varieties
To find the number of ways to fill the places with exactly two different varieties, we first choose 2 varieties from the 3 available. The number of ways to choose 2 varieties from 3 is given by:
(32)=3
For each pair of varieties chosen, we can fill the 5 places in such a way that at least one of each variety is used. The total arrangements for 5 places using 2 varieties can be calculated as:
25−2=32−2=30
(The subtraction of 2 accounts for the cases where all places are filled with only one of the two chosen varieties.)
Step 5: Combine All Cases
Now we combine all the cases:
1. Total arrangements without restriction: 35=243
2. Subtract arrangements with one variety: 3
3. Subtract arrangements with exactly two varieties: 3×30=90
So, the final calculation is:
Total Ways=243−3−90=150
Final Answer
The number of different ways of displaying the three varieties of perfumes in the showcase is:
150
We have three varieties of perfumes (let's call them A, B, and C) and five places in a row to display them. Since there is a large number of bottles of each variety, we can use any variety in any place.
Since there are no restrictions on how we can fill the places, each of the 5 places can be filled with any of the 3 varieties. Therefore, for each place, we have 3 choices.
Total Ways=35
Now we calculate 35:
35=3×3×3×3×3=243
Next, we consider the restrictions mentioned in the video transcript. We need to subtract the cases where all places are filled with only one variety and where only two varieties are used.
If all 5 places are filled with the same variety, we can choose any of the 3 varieties. Thus, there are:
3 ways (A, B, or C)
To find the number of ways to fill the places with exactly two different varieties, we first choose 2 varieties from the 3 available. The number of ways to choose 2 varieties from 3 is given by:
(32)=3
For each pair of varieties chosen, we can fill the 5 places in such a way that at least one of each variety is used. The total arrangements for 5 places using 2 varieties can be calculated as:
25−2=32−2=30
(The subtraction of 2 accounts for the cases where all places are filled with only one of the two chosen varieties.)
Now we combine all the cases:
1. Total arrangements without restriction: 35=243
2. Subtract arrangements with one variety: 3
3. Subtract arrangements with exactly two varieties: 3×30=90
Total Ways=243−3−90=150
The number of different ways of displaying the three varieties of perfumes in the showcase is:
150
Topper's Solved these Questions
- FUNCTIONS AND GRAPHBOOK - ARIHANT SSCCHAPTER - FUNCTIONS AND GRAPHEXERCISE - Final Round
40 Videos
- FUNCTIONS AND GRAPHBOOK - ARIHANT SSCCHAPTER - FUNCTIONS AND GRAPHEXERCISE - EXERCISE(LEVEL 1)
84 Videos
- ELEMENTS OF ALGEBRABOOK - ARIHANT SSCCHAPTER - ELEMENTS OF ALGEBRAEXERCISE - EXERCISE(LEVEL 1)
32 Videos
- FUNDAMENTALS BOOK - ARIHANT SSCCHAPTER - FUNDAMENTALS EXERCISE - TEST OF YOU - LEARNING - 2
40 Videos
Similar Questions
A shopkeeper sells three varieties of perfumes and he has a large number of bottles of the same size of each variety in his stock. There are 5 places in a row in his showcase.The number of different ways of displaying the three varieties of perfumes in the show case is
View SolutionAn ice cream parlour has ice cream in 8 different varieties. Number of ways of choosing 3 ice creams taking atleast 2 ice creams of the same variety is
View Solution
Knowledge Check
A shopkeeper sells three varieties of perfumes and he has a large number of bottles of the same size of each variety in his stock. There are 5 places in a row in his showcase. The number of different ways of displaying the three varieties of perfumes in the show case, is
A6B50C150Dnone of theseA shopkeeper sells three varieties of perfumes and he has a large number of bottles of the same size of each variety in his stock. There are 5 places in a row in his showcase. The number of different ways of displaying the three varieties of perfumes in the show case, is
A6B50C150DNone of the aboveA sweet shop sells 9 verities of sweets. The shopkeeper wants to pick not more than one piece of any variety of sweets to make the assortment of sweets. He then packs equal number of pieces in each box from variety of sweets in order to have maximum number of distinct assortments. How many verities of sweets should he pack in a box so that each box contains distinct assortment of sweets ?
AA) 3BB) 4CC) 5DD) BOTH (B) AND ( C)
Walking down the alluring streets of Paris Jasmine gets attracted to a Vintage shop. The squad enters the shop and they found out a lot of amazing vintage stuff and decided to buy some. The shopkeeper offered them to take the products for free if they are able to solve his riddle. He stated that a person has 2 cubes on his desk. Every day he arranges both the cubes so that the front faces show the current day of the month. Different faces of both the cubes are coloured in 6 different colours. What can be the maximum possible sum of the product of numbers appearing on the same coloured face?
View SolutionHarmeet Singh owns a large scale retail outlet in Chandigarh. He deals with various kinds of products in his shop like textiles footwear, cosmetics, stationary, Grocery, etc. He sells tham in different divisions in his big shop.
In context of the above cases:
1. Identify the type of retail outlet being described in the above lines.
2. State any three features of that outlet.
View SolutionThree different positions of the same dice are shown have which number is on the face opposite the face showing 5?

View SolutionFive balls of different colours are to be placed in three boxes of different sizes. Each box can hold all the five balls. In how many ways can we place the balls so that no box remains empty?
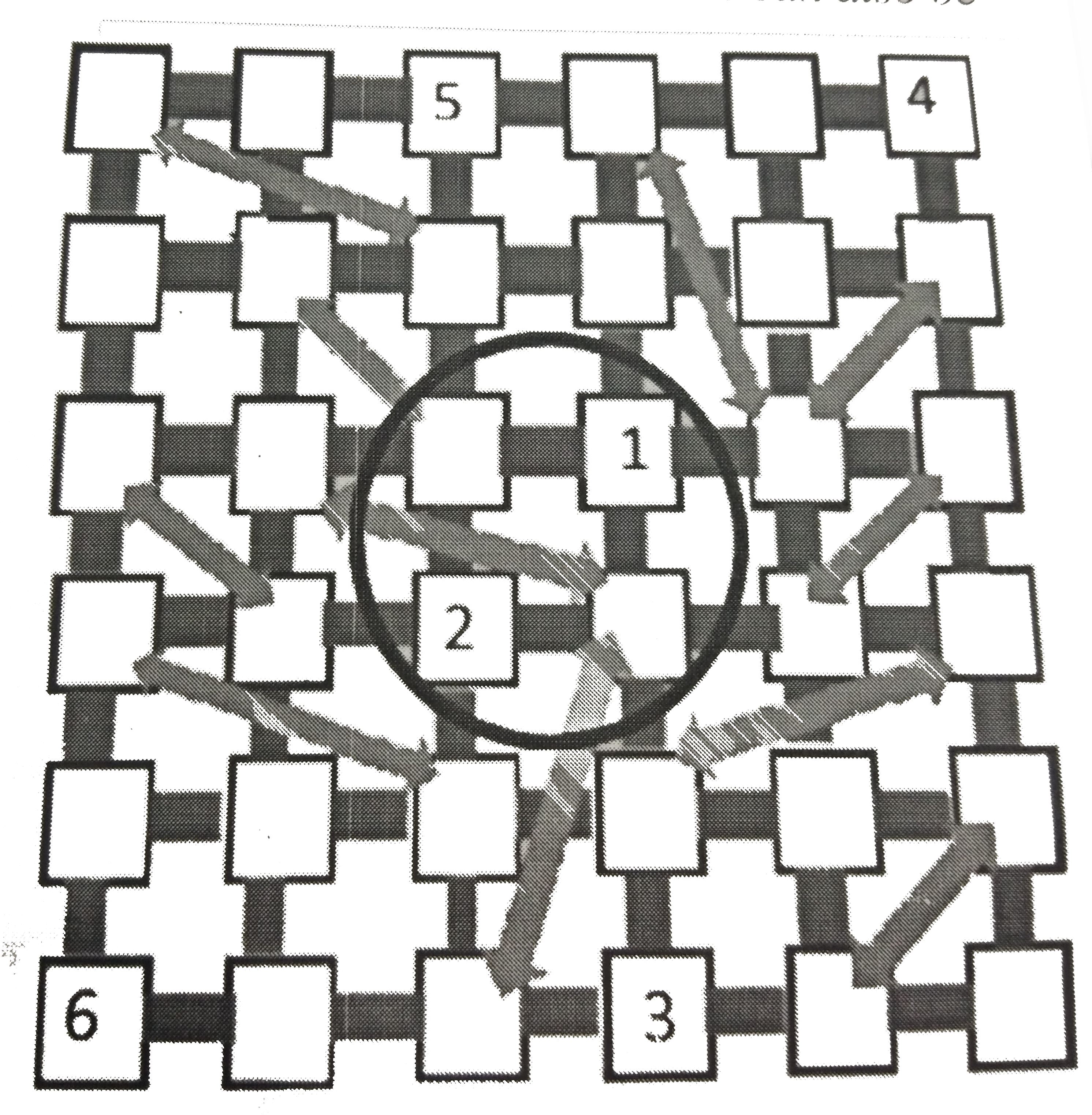
View SolutionAfter having celebrated his brother’s birthday, Max makes his way to Fuchsia city, to take a boat to Cinnabar Island. The sailor, Mr. Hendricks, is however in a dilemma. There is a castle in the middle of the sea on the way to Cinnabar Is land. Owing to issues, he needs to bribe each and every soldier with 10 PokéDollars to pass through. He is not sure of the number of soldiers in the main towers due to the new rules that have been imposed. He seeks Max’s help. In the castle, there are passways as straight row and column (passways are the dark grey strips) at any point when you see left or right or up or down . Also there are some magical tunnels joining one stop (stops are the places where troops of soldiers stand) to the other in a non-straight manner. The magic of the tunnel is clon- ing the soldiers. If you place any number of soldiers in one stop, then all stops joined to it through the tunnel will have same number of cloned soldiers. Cloned soldiers can also be cloned if there is one more. Number of soldiers at any stop in any row or column are more than one and sum of all soldiers at all stops in any row or column is same. Every stop is marked a number from 1 to 6 and stops in any row or column have different numbers. Soldier troop with ‘x’ number of soldier will stand on the stop marked as ‘x’. There are some soldier having all time duty and they are depicted in the figure with their positions. But fearing for safety, the king increased the security and ordered to place a soldier on every stop in the above way of his convenience. The circle in the middle represents the main tower and Max has to find the number of extra soldiers. The answer is ________
View Solution
